5 Essential Mole Ratio Worksheet Answer Key Tips
In the realm of chemistry, understanding mole ratios is fundamental for balancing equations, determining reaction yields, and making predictions about chemical processes. Mole ratio worksheets serve as an excellent tool for students to solidify their comprehension of these concepts. However, mastering the intricacies of mole ratio worksheets can be daunting. This post delves into five essential tips that will help you conquer these worksheets and enhance your understanding of stoichiometry.
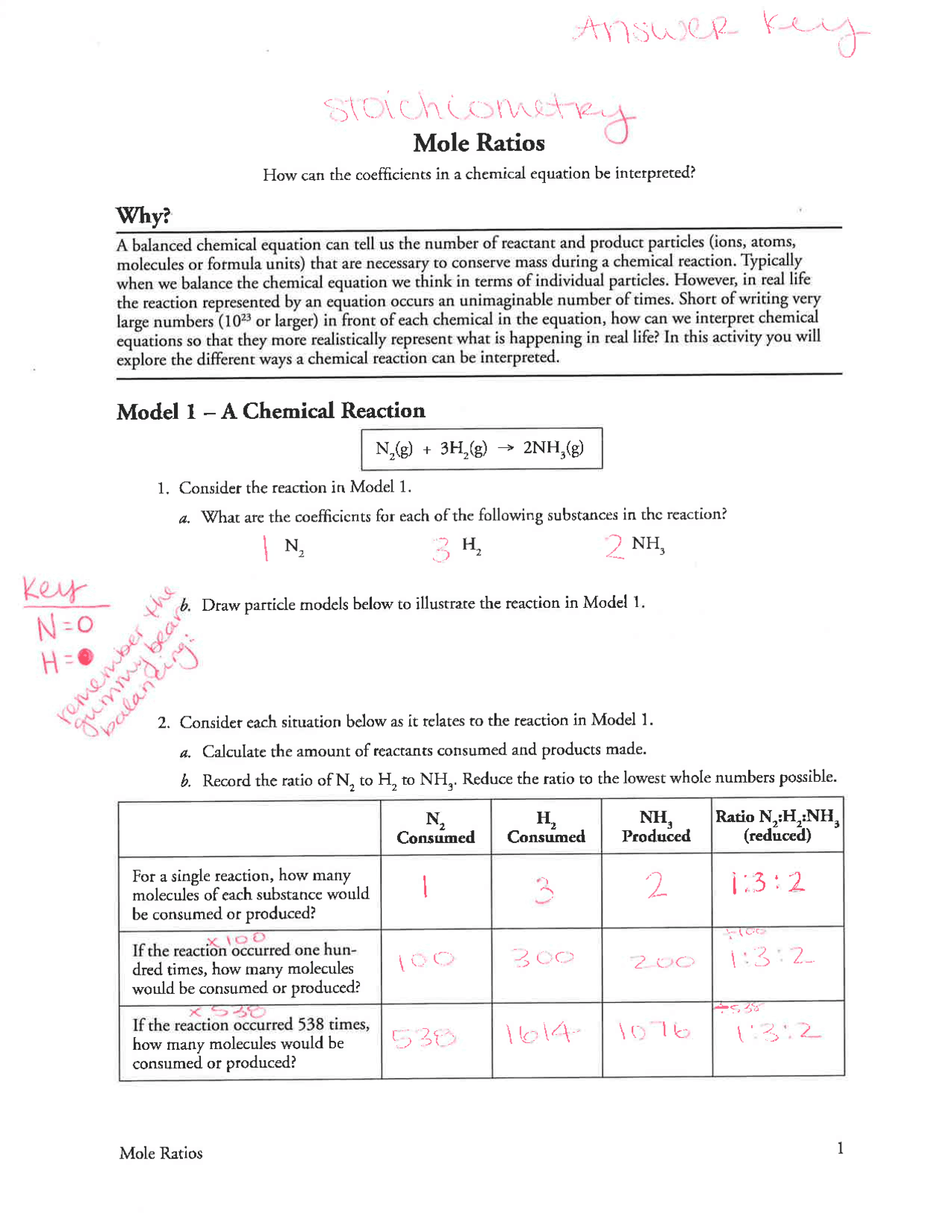
1. Understand the Balanced Equation

Before diving into calculations, make sure you fully comprehend the balanced chemical equation. A mole ratio worksheet starts with:
- Identifying the reactants and products.
- Ensuring the equation is balanced, where the number of atoms for each element on the reactant side equals the number on the product side.
An unbalanced equation will lead to incorrect mole ratios and consequently, incorrect answers. Always double-check the equation’s balance before proceeding.

📝 Note: A balanced equation is crucial for accurate mole ratio calculations. If the equation isn’t balanced, adjust the coefficients before proceeding with calculations.
2. Master the Concept of Mole Ratios

Mole ratios are derived from the coefficients of a balanced equation. For instance, in the reaction:
2H₂(g) + O₂(g) → 2H₂O(g)
The mole ratios can be:
- 2 moles of H₂ to 1 mole of O₂
- 1 mole of O₂ to 2 moles of H₂O
To solve problems:
- Identify the substances involved in the reaction.
- Locate their respective coefficients in the balanced equation.
- Use these coefficients to form ratios.
Remember, these ratios remain constant regardless of the quantities of reactants used.

3. Convert Mass to Moles

When working with mole ratios, often you’ll encounter mass rather than moles. Here’s the process to convert:
- Use the molar mass of a substance to convert grams to moles.
- Apply the balanced equation’s coefficients to determine the mole ratios.
Here’s how:
| Element | Atomic Mass (amu) |
|---|---|
| Hydrogen (H) | 1.008 |
| Oxygen (O) | 16.00 |
| Water (H₂O) | 18.02 (rounded) |

To find moles from mass:
(mass of substance in grams) ÷ (molar mass of substance in g/mol) = moles
🧮 Note: If you're struggling with calculations, ensure your unit conversions are correct and double-check your molar masses.
4. Use Dimensional Analysis

Dimensional analysis, or the factor-label method, is invaluable for mole ratio problems:
- Start with what you know (e.g., grams of reactant).
- Use conversion factors to move to moles.
- Apply the mole ratios from the balanced equation to solve for unknowns.
This technique helps in keeping track of units and ensuring consistency in your calculations.

5. Check Your Work

Finally, always verify your work to ensure accuracy:
- Recheck the balancing of the equation.
- Confirm that your mole ratios correspond to the coefficients.
- Re-examine your conversions from mass to moles.
- Calculate any yield (theoretical or percentage) to ensure reasonability.
🔍 Note: A common mistake is misinterpreting or misapplying the coefficients from the equation. Always relate your answer back to the balanced equation for coherence.
As you conclude your journey through mole ratio worksheets, remember that these tips are not merely shortcuts but essential methods for understanding the intricate world of chemistry. Balancing equations, converting mass to moles, and applying mole ratios are foundational skills that underpin stoichiometry. By applying these techniques, you'll develop a deeper appreciation for how substances react and how they're quantified in chemical reactions, enhancing both your problem-solving skills and your comprehension of chemistry.
What is the significance of mole ratios in chemistry?

+
Mole ratios are crucial in understanding the quantitative relationships between reactants and products in chemical reactions, allowing chemists to predict outcomes, balance equations, and calculate reaction yields.
How do you find the molar mass?

+
The molar mass of a substance is found by summing the atomic masses of all atoms in its formula, typically measured in grams per mole (g/mol). You can find atomic masses on the periodic table.
Can mole ratios change with different conditions?

+
No, mole ratios derived from a balanced equation remain constant regardless of changes in temperature, pressure, or concentration, as they are based solely on the stoichiometry of the reaction.



