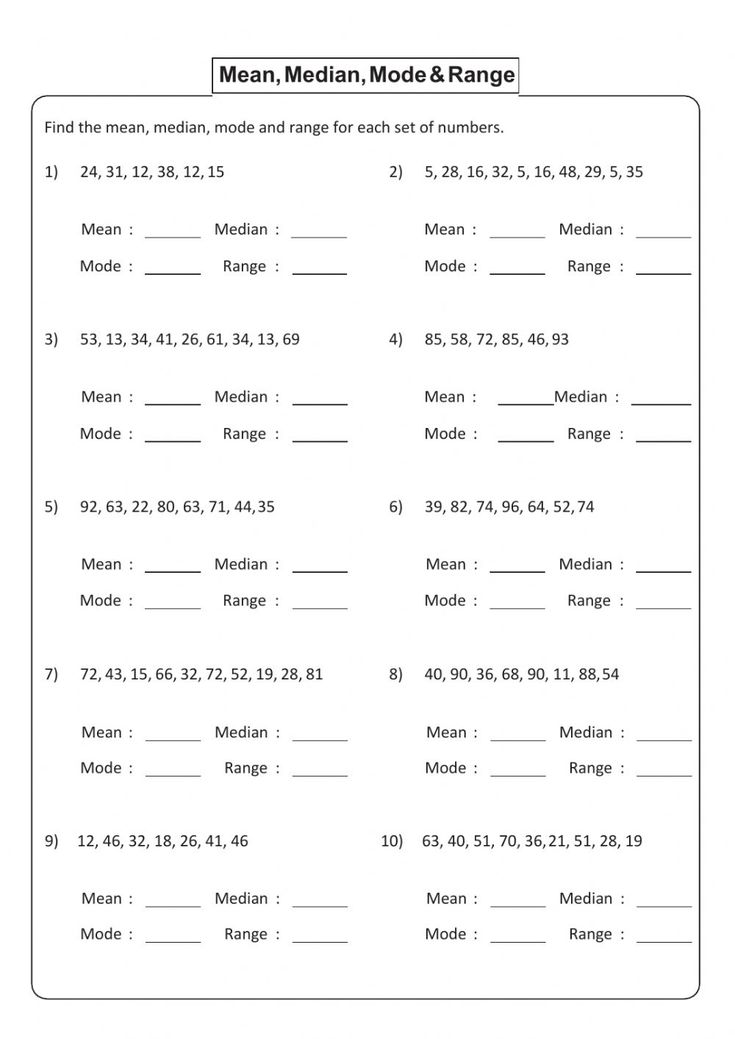
Mean, Mode, Median, Range: Worksheet with Answers

Welcome back to our series on basic statistics! Today, we delve into the fundamental concepts of mean, mode, median, and range, which are essential tools in understanding datasets in various fields like economics, psychology, engineering, and more. This post will guide you through these concepts with examples, provide practical exercises, and enhance your understanding through direct application. Whether you're a student, teacher, or statistics enthusiast, this worksheet will serve as a valuable resource.
The Basics of Measures of Central Tendency

The mean, median, and mode are known as the measures of central tendency because they tell us where the 'center' or average of a data set lies.
- Mean: Also known as the average, this is calculated by summing all values in a data set and then dividing by the number of observations.
- Median: This is the middle number in a sorted dataset. If there is an even number of observations, it's the average of the two middle numbers.
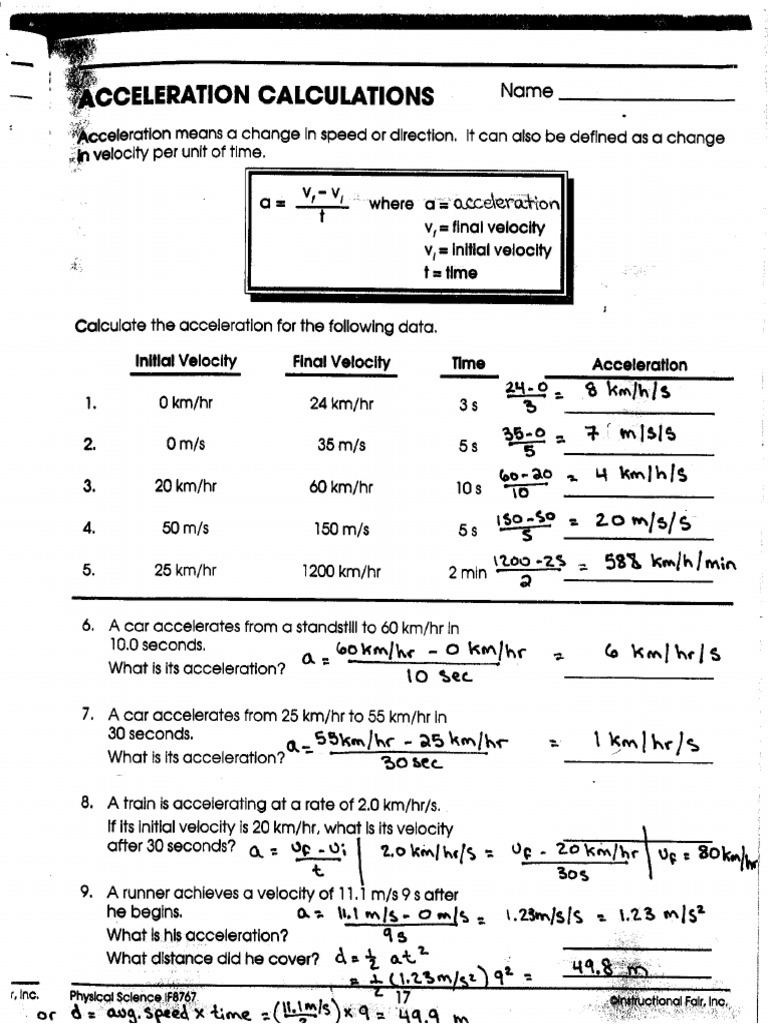
- Mode: This is the value that appears most frequently in the dataset. There can be no mode, one mode, or multiple modes.
Range
The range is the simplest measure of dispersion, calculated as the difference between the highest and the lowest values in the dataset.
Example Problems

Calculating Mean

Here’s how you can calculate the mean for a simple dataset:
| Data Set | Calculation |
|---|---|
| 2, 4, 4, 5, 6 | (2 + 4 + 4 + 5 + 6) / 5 = 4.2 |

Finding the Median

Consider the following dataset to find the median:
| Original Data Set | Sorted Data Set | Median |
|---|---|---|
| 5, 2, 9, 1, 7 | 1, 2, 5, 7, 9 | 5 |
📌 Note: For an even-numbered dataset, the median is the average of the two middle values.
Identifying the Mode

Identify the mode in this dataset:
| Data Set | Mode |
|---|---|
| 4, 5, 4, 2, 3, 4 | 4 |
🌟 Note: The mode does not exist if no number repeats, and there can be multiple modes if several values occur the same maximum number of times.
Range Calculation

Calculate the range using the following dataset:
| Data Set | Range |
|---|---|
| 12, 23, 34, 54, 13, 29 | 54 - 12 = 42 |
Interactive Worksheet

Let's put these concepts into practice with an interactive worksheet. Below, you'll find exercises to help you test your understanding:
Problem Set

- Calculate the mean, median, mode, and range for the dataset: 18, 15, 12, 18, 19, 17.
- What is the mean, median, and mode of: 5, 9, 8, 8, 12, 5?
- If a data set includes all values from 1 to 10, what is the range?
Solutions

- Mean: (18+15+12+18+19+17)/6 = 16.5
- Median: (18+15+12+18+19+17) -> sorted = 12, 15, 17, 18, 18, 19 -> Median = 17.5
- Mode: 18
- Range: 19 - 12 = 7
- Mean: (5+9+8+8+12+5)/6 = 7.83
- Median: (5+5+8+8+9+12) -> sorted = 5, 5, 8, 8, 9, 12 -> Median = 7.5
- Mode: 5 & 8
- Range: 12 - 5 = 7
- Range: 10 - 1 = 9
Today, we've explored the definitions, calculations, and applications of mean, median, mode, and range. These measures are essential in summarizing and analyzing data, providing insights into the central values and dispersion of a dataset. The practice exercises should have solidified your grasp on these topics, making you better prepared to handle statistical data in your studies or work.
Why are there several measures of central tendency?

+
Each measure (mean, median, mode) provides a different perspective on the data. The mean gives the arithmetic average, the median helps to understand the middle value which is not influenced by outliers, and the mode indicates the most frequent value in the dataset. Different measures are used depending on the nature of the data or the analytical context.
Can a dataset have no mode?

+
Yes, a dataset can have no mode if all values are unique. In this case, no single value occurs more frequently than others.
How does the range relate to data variability?

+
The range measures the spread or variability of the data. A larger range indicates that the data points are spread out over a wide range of values, while a smaller range suggests that the data points are closely packed together.