5 Quick Tips for Mastering Acceleration Calculations
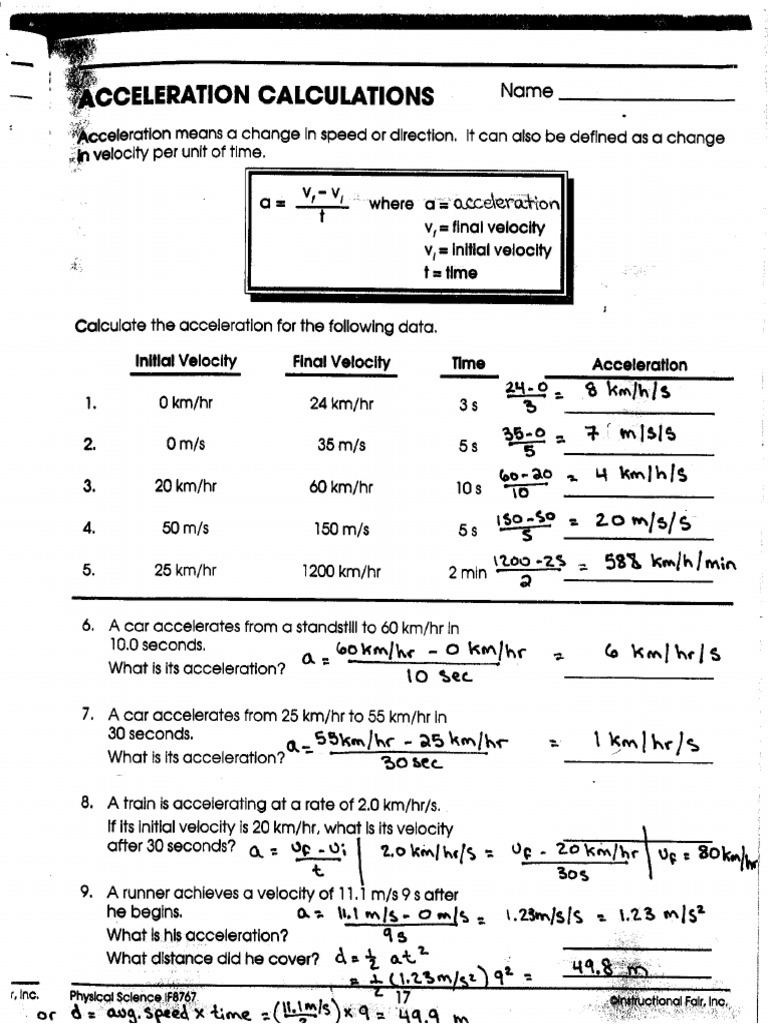
Mastering acceleration calculations in physics or engineering can be a pivotal skill for enhancing your understanding of motion dynamics. This article will provide you with five essential tips to ace your acceleration calculations, whether you're tackling complex physics problems or simply looking to deepen your knowledge of kinematics.
Understanding the Basics of Acceleration

Before diving into complex calculations, it’s crucial to understand what acceleration means. Acceleration is defined as the rate of change of velocity with respect to time. Here are some fundamental points:
- Formula: Acceleration (a) = Change in Velocity (Δv) / Time (Δt)
- The unit of acceleration is meters per second squared (m/s²)
- Acceleration can be positive (speeding up), negative (slowing down), or zero (constant velocity).

Tip 1: Use the Correct Equations

Selecting the right equation for your calculations can make all the difference. Here are some commonly used acceleration equations:
| Equation | Description |
|---|---|
| a = (v - u) / t | Where ‘u’ is initial velocity, ‘v’ is final velocity, and ’t’ is time |
| v² = u² + 2as | Connects velocity, initial velocity, acceleration, and displacement ’s’ |
| s = ut + (1⁄2)at² | Relates distance with time, initial velocity, and acceleration |

Tip 2: Consider Directional Signs

Acceleration is vector-based, meaning it has both magnitude and direction. Keep these points in mind:
- If an object is moving in the positive direction, positive acceleration means it’s speeding up, and negative means it’s slowing down.
- If the object is moving in the negative direction, these signs are reversed.
Tip 3: Employ Graphical Methods

Graphical representations can simplify the understanding of acceleration:
- Plotting velocity-time graphs where the slope represents acceleration.
- Distance-time graphs where the curvature indicates acceleration.
Here’s an example of how acceleration might look on a velocity-time graph:
Tip 4: Practice With Real-World Scenarios

Applying acceleration in real-life situations can solidify your understanding:
- Imagine accelerating a car from a stoplight to highway speed.
- Consider the deceleration of a skydiver using a parachute.
- Think about how an elevator moves between floors, including its acceleration up and down.
Tip 5: Utilize Software and Tools

Physics simulation software and graphing calculators can be excellent tools:
- Software like PhET Interactive Simulations for visual learning.
- Spreadsheet programs like Excel to plot graphs and solve equations.
- Scientific calculators or apps designed for physics calculations.
💡 Note: While technology is a great aid, understanding the underlying principles without reliance on tools can lead to deeper learning and better problem-solving skills.
In summing up the key tips for mastering acceleration calculations, we have emphasized the importance of understanding fundamental concepts, choosing the right equations, considering directionality, using graphical analysis, practicing with real-world scenarios, and employing various tools for learning. Remember, mastering acceleration requires patience, practice, and a methodical approach to ensure accuracy and efficiency in your calculations.
What is acceleration?

+
Acceleration is the rate at which an object changes its velocity. It is a vector quantity, which means it has both magnitude (speed) and direction.
How does one calculate acceleration?

+
To calculate acceleration, you subtract the initial velocity from the final velocity and divide by the time taken: a = (v - u) / t.
Why is it important to consider the direction of acceleration?

+
The direction of acceleration is crucial because it dictates whether an object is speeding up, slowing down, or changing direction. It affects how we interpret the motion of an object.