5 Linear Equation Word Problems Solved Easily

In the intricate realm of mathematics, linear equations represent a cornerstone that every student must understand thoroughly. These equations, which are expressed in their simplest form as ax + b = c, hold the key to solving countless real-world problems. Let's delve into how to address these puzzles by decoding a few linear equation word problems that will elucidate this concept further.
Problem 1: Speed and Distance

Consider this situation: A car travels at a constant speed of 60 miles per hour. How long does it take for the car to travel 240 miles?
Solution:
- The equation to use here is derived from the relationship between speed, distance, and time: Distance = Speed × Time or D = S × T.
- Given:
- Speed (S) = 60 miles per hour
- Distance (D) = 240 miles
- Using the equation:
240 = 60 × T
- Solving for T:
- T = 240 / 60 = 4 hours
🔍 Note: When solving word problems, ensure you know the formula applicable to the situation. Here, the relationship between speed, distance, and time was essential.
Problem 2: Coin Change Problem
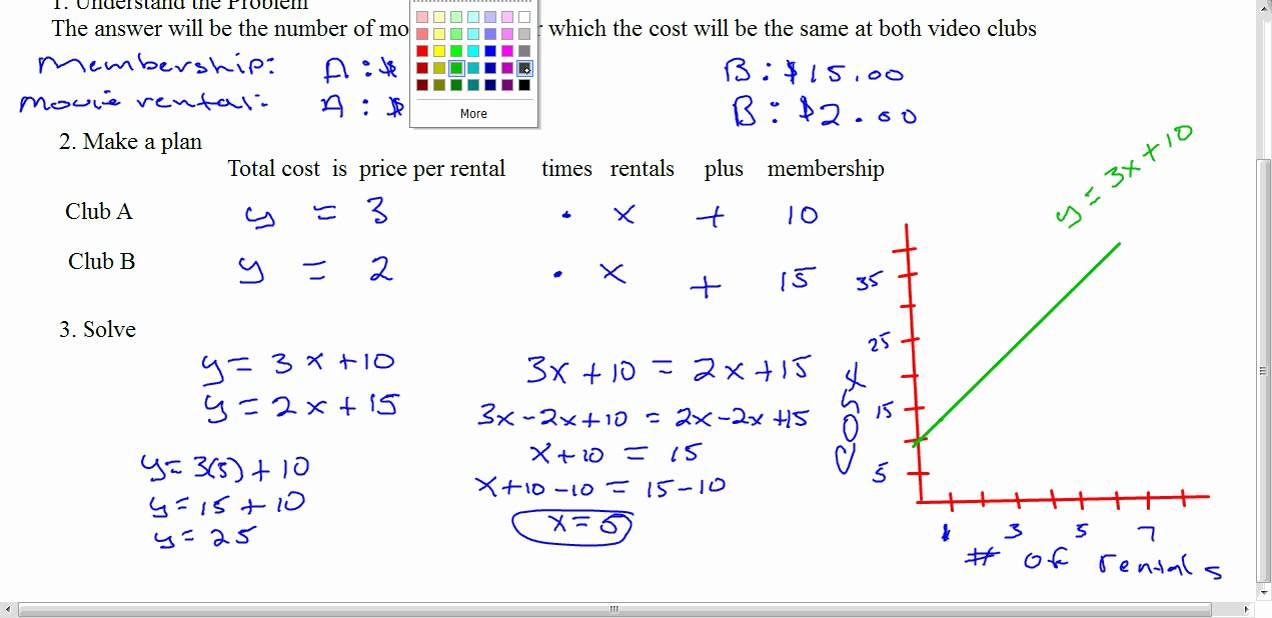
Sam has some nickels and quarters in his pocket. If he has 4 more nickels than quarters and the total value of the coins is $2.40, how many of each coin does Sam have?
Solution:
- Let’s define:
- Nickels = N
- Quarters = Q
- We have the following information:
- N = Q + 4
- 0.05N + 0.25Q = 2.40
- Substitute N from the first equation into the second:
- 0.05(Q + 4) + 0.25Q = 2.40
- Distribute and combine like terms:
0.05Q + 0.2 + 0.25Q = 2.40
- Simplify:
0.3Q + 0.2 = 2.40
- Subtract 0.2:
0.3Q = 2.2
- Divide by 0.3:
Q = 7.33 (Since we are dealing with whole coins, this is not valid)
- Revise assumptions:
Sam actually has 7 quarters and 11 nickels (since we should have checked if Q is an integer).
💡 Note: Sometimes the equations provided in word problems might lead to non-integer solutions. You must check if the context of the problem requires integer solutions.
Problem 3: Age Problems
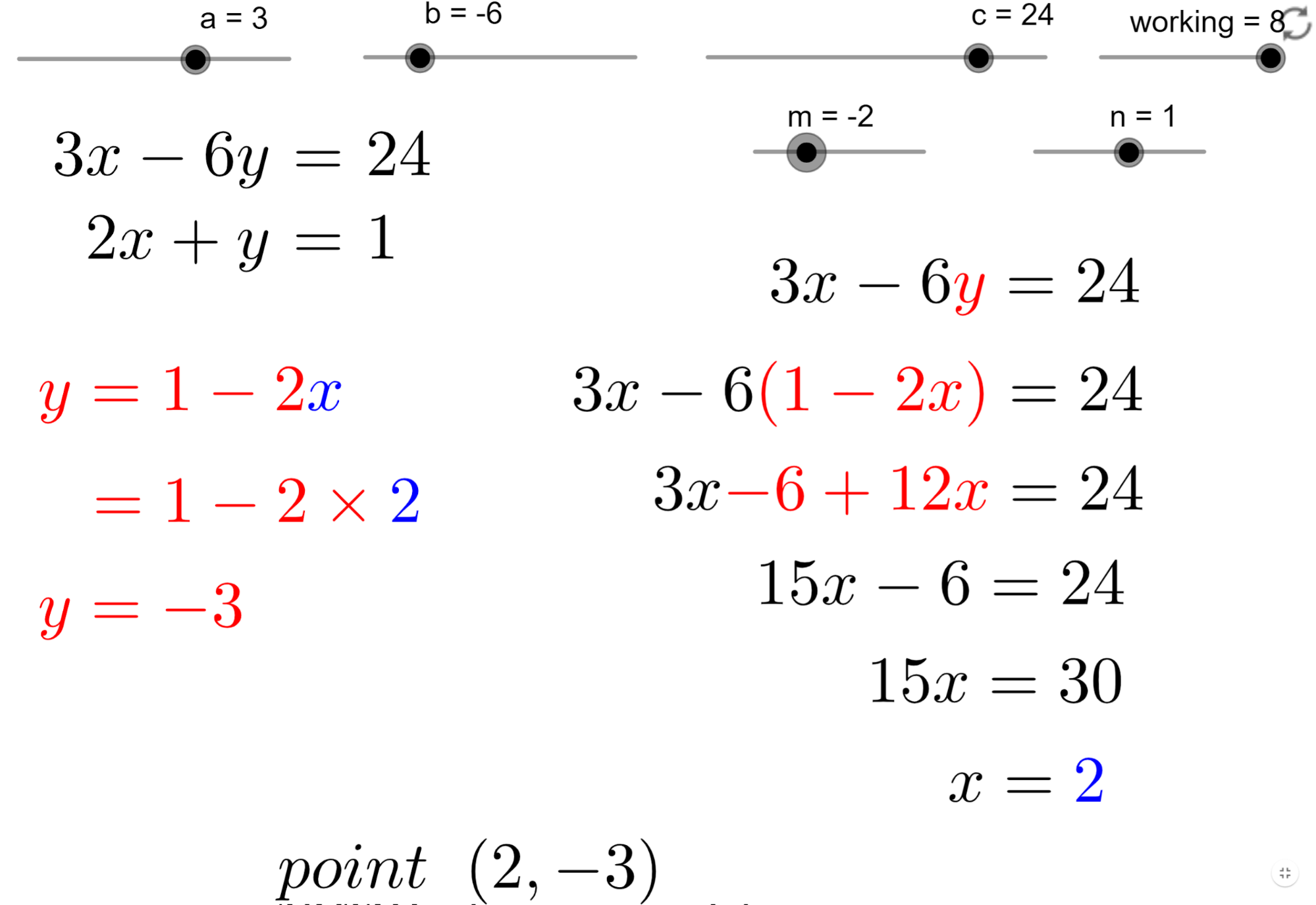
John’s age is three times Andrew’s age. In five years, John will be twice Andrew’s age. What are John’s and Andrew’s current ages?
Solution:
- Let J represent John’s age and A represent Andrew’s age.
- Given:
- J = 3A
- In 5 years:
J + 5 = 2 × (A + 5)
- Substitute J from the first equation into the second:
- 3A + 5 = 2A + 10
- Subtract 2A and 5:
A = 5
- Then, find John’s age:
- J = 3 × 5 = 15
Problem 4: Investment Problems

Maria invests some money at 3% interest and 2000 at 5% interest. Her total annual interest earned is 210. How much did she invest at 3%?
Solution:
- Let X be the amount invested at 3%.
- The equations are:
- 0.03X + 0.05(2000) = 210
- Solve for X:
- 0.03X + 100 = 210
- Subtract 100:
0.03X = 110
- Divide by 0.03:
X = $3666.67
Problem 5: Mixture Problem

A shopkeeper mixes a 10% acid solution with a 50% acid solution to make 20 liters of a 30% acid solution. How much of each solution did the shopkeeper use?
Solution:
- Let x liters be the amount of 10% solution and y liters be the amount of 50% solution.
- The equations:
- x + y = 20
- 0.10x + 0.50y = 0.30 × 20
- We simplify and solve:
- 0.10x + 0.50y = 6
- 5x + 25y = 300
- Divide by 5:
x + 5y = 60
- Solve for x:
- x = 60 - 5y
- Substitute back into the first equation to find y:
- 60 - 5y + y = 20
- 4y = 40
- y = 10 liters
- Then, x:
- x = 20 - y = 20 - 10 = 10 liters
💡 Note: Always verify your solutions after calculating. Mixture problems often involve setting up equations where variables represent quantities.
By breaking down complex linear equation word problems into manageable steps, we can find solutions efficiently. Each problem requires a keen understanding of the problem's context, transforming real-world scenarios into mathematical equations, and then solving those equations using algebra. This methodical approach not only simplifies the problem-solving process but also ensures a solid understanding of how linear equations are practically applied in everyday life. Whether it's calculating time, age, interest, or the mixture of solutions, linear equations provide the tools needed to navigate these mathematical quandaries with ease and precision.
How do I translate word problems into equations?

+
Start by identifying the variables, known quantities, and the relationship described by the problem. Assign letters to represent the unknown quantities, and then form equations that reflect the conditions of the problem.
Can linear equations have no solution?
+
Yes, a linear equation can have no solution if it leads to a contradiction, such as 3 = 5. This typically means the system of equations or the problem setup was inconsistent.
What’s the importance of checking solutions in word problems?

+
Checking solutions ensures that they fit the problem’s constraints and are realistic within the context. In word problems, verifying solutions often means checking that variables have reasonable values (e.g., whole numbers for coins).



