5 Essential Limits Calculus Tips for Students

Calculus, often deemed a challenging math subject, plays a crucial role in understanding the behavior of functions and solving problems in engineering, physics, and many other fields. While limits form the foundational blocks of calculus, students often grapple with this concept, finding the logic and techniques elusive. Here, we'll explore five essential limits calculus tips that can empower students to not only grasp this concept but also excel in applying it.
Understanding the Concept of Limits
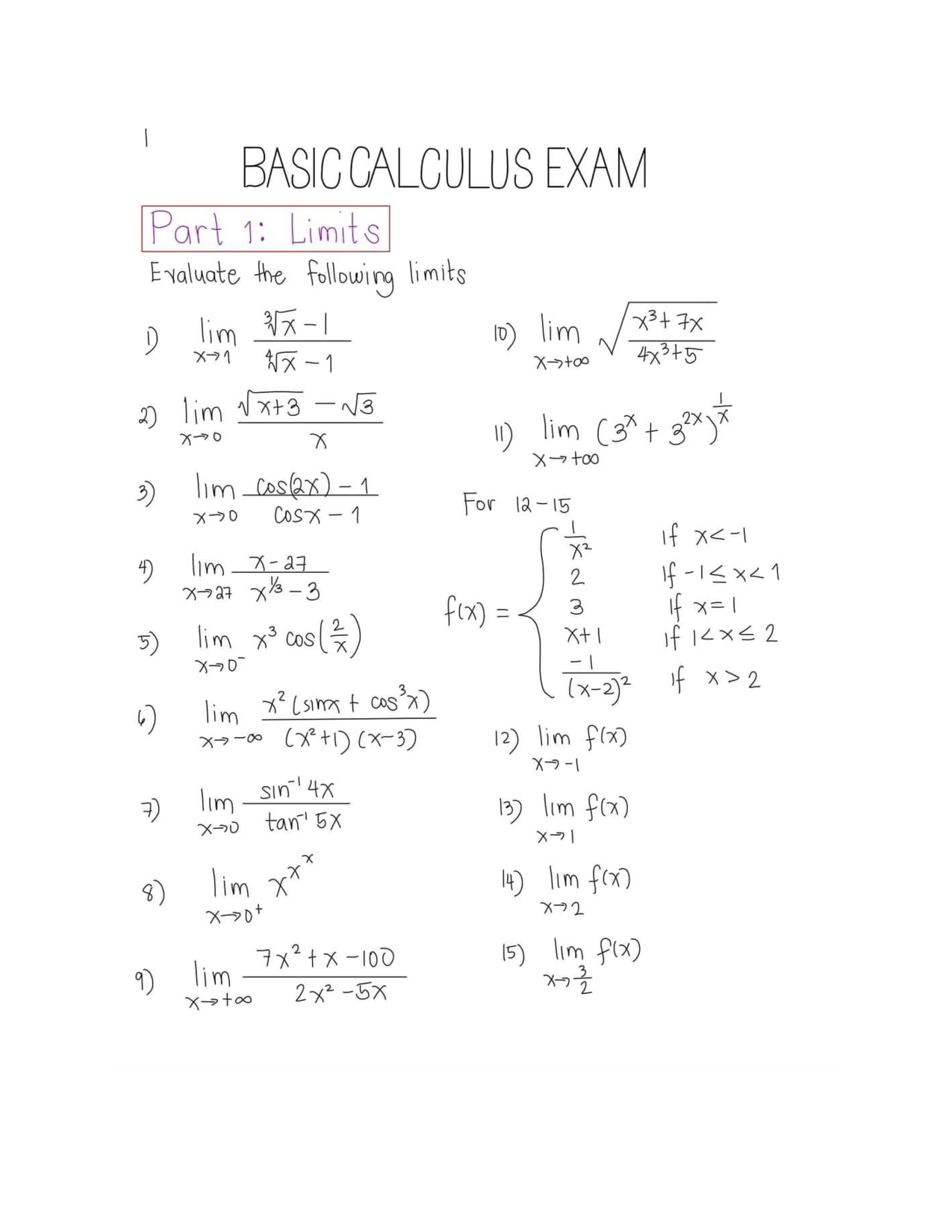
Limit is a fundamental concept in calculus, representing the value that a function approaches as the input (or variable) approaches some value. Here are some tips:
- Visualization: Visualize functions through graphs. The limit at a particular point is where the function seems to be heading when you get very close to that point.
- Right and Left Hand Limits: Understand that the limit from the left might not always be the same as from the right. Sometimes, limits from different sides don’t match, indicating that the limit does not exist.
- Infinite Limits: Recognize that as you approach infinity or negative infinity, a function might approach a specific value or diverge to positive or negative infinity.
Mastering the Squeeze Theorem

The Squeeze Theorem is a powerful tool for finding limits that are otherwise difficult to compute:
- How it works: If you have three functions, where one function lies between the other two, and the outer functions approach the same limit, then the middle function also approaches that same limit.
- Application: For instance, to find (\lim_{x \to 0} x^2 \sin(\frac{1}{x})), we can use the fact that (-1 \leq \sin(\frac{1}{x}) \leq 1), squeeze (x^2 \sin(\frac{1}{x})) between (-x^2) and (x^2), which both approach 0.
💡 Note: Squeeze Theorem is particularly useful when you encounter trigonometric functions combined with polynomials.
Using L’Hôpital’s Rule Effectively

L’Hôpital’s Rule is a shortcut to finding limits of indeterminate forms:
- Indeterminate Forms: It’s applicable when you encounter (\frac{0}{0}) or (\frac{\infty}{\infty}) as a limit.
- Process: Instead of evaluating the limit directly, differentiate the numerator and denominator separately and then take the limit again. Keep doing this until the indeterminate form disappears or you reach a calculable limit.
- Example: Consider (\lim_{x \to \infty} \frac{\ln(x)}{x}). Applying L’Hôpital’s Rule, (\ln(x) \div x) becomes (\frac{1}{x} \div 1), which simplifies to (\frac{1}{x}) approaching 0.
📌 Note: L’Hôpital’s Rule doesn’t work for every situation where the limit is indeterminate; always check if applying the rule results in a defined limit.
Employing Continuity and Direct Substitution

If a function is continuous at a point, you can find its limit by direct substitution:
- Check Continuity: If the function is continuous at (a), then (\lim_{x \to a} f(x) = f(a)).
- Substitution: This approach simplifies many limit calculations, especially for polynomial and rational functions.
- Common Pitfalls: Watch out for cases where direct substitution results in an undefined expression or indeterminate form; these require additional techniques like factoring or rationalization.
Handling Limits at Infinity and Asymptotes

Asymptotic behavior is a vital part of understanding limits:
- Horizontal Asymptotes: If a function (f(x)) approaches a finite value as (x) goes to infinity or negative infinity, it has a horizontal asymptote.
- Vertical Asymptotes: These occur when the function approaches infinity or negative infinity as (x) approaches a specific finite value from one or both sides.
- Using Transformations: Techniques like polynomial division or factoring can simplify functions to reveal their asymptotic behavior.
| Type of Limit | Description |
|---|---|
| At Infinity | Deals with the function’s behavior as (x) approaches (\pm \infty) |
| Asymptotes | Indicates where the function becomes unbounded or approaches a constant value |

Understanding these tips and integrating them into your calculus problem-solving toolkit can significantly enhance your ability to solve complex limit problems. Each of these techniques provides a methodical approach to dealing with different types of limit problems, making calculus not just easier but also more enjoyable to learn.
Embracing these strategies not only aids in mastering limits but also paves the way for deeper understanding of calculus concepts like derivatives, integrals, and beyond. Keep practicing, and remember that each type of limit problem might require a different approach. The journey through calculus is an exciting exploration of mathematical landscapes, where each problem solved reveals new insights into the behavior of functions.
What is the difference between one-sided and two-sided limits?

+
One-sided limits approach the limit value from either the left or right side of the function, whereas two-sided limits consider the limit as the variable approaches from both directions. For a limit to exist, both one-sided limits must exist and be equal.
How do you know when to use L’Hôpital’s Rule?

+
Use L’Hôpital’s Rule when you encounter an indeterminate form, like (\frac{0}{0}) or (\frac{\infty}{\infty}). Differentiate the numerator and denominator, then take the limit again.
Can limits exist if the function is not defined at a point?

+
Yes, a limit can exist even if the function is undefined at that point. This is often seen with rational functions where the denominator might be zero at a specific point, but the limit can still be calculated from the behavior around that point.
Why are limits important in calculus?

+
Limits are essential because they define key concepts like continuity, derivatives, and integrals. They help us understand how functions behave as inputs approach certain values, which is crucial for solving real-world problems involving rates of change, optimization, and accumulation.



