5 Easy Steps for Adding Fractions Worksheet Practice

Adding fractions is a fundamental skill in arithmetic that can challenge many students, particularly when the denominators are different. Fortunately, with the right steps and a bit of practice, mastering the addition of fractions can become a straightforward task. This article outlines 5 easy steps to teach or learn how to add fractions effectively. Let's dive into these steps and incorporate a worksheet practice to solidify your understanding.
Step 1: Understanding the Basics

Before we can add fractions, understanding what a fraction represents is crucial. A fraction consists of two parts:
- Numerator: The number above the line, representing the part of the whole.
- Denominator: The number below the line, which signifies how many parts the whole has been divided into.
💡 Note: Remember, when adding fractions, we are combining parts into a total, not comparing the size of those parts.
Step 2: Finding a Common Denominator

When you have fractions with different denominators, you can’t simply add the numerators. Here’s what you do:
- Identify the Least Common Multiple (LCM) of the denominators or find the smallest number both can evenly divide into.
- Convert each fraction into equivalent fractions with this common denominator.
| Fractions | Denominators | Common Denominator |
|---|---|---|
| 1⁄3 + 2⁄5 | 3, 5 | 15 |
| 3⁄7 + 4⁄9 | 7, 9 | 63 |

⚠️ Note: Finding the LCM can sometimes be tricky, but using a calculator or following a step-by-step method can help.
Step 3: Converting the Fractions

Once you have the common denominator, you convert the fractions:
- Multiply the numerator and denominator of each fraction by the same number that will make the denominator equal to the common denominator.
Let’s convert the above examples:
- 1⁄3 becomes 5⁄15 (1*5⁄3*5)
- 2⁄5 becomes 6⁄15 (2*3⁄5*3)
- 3⁄7 becomes 27⁄63 (3*9⁄7*9)
- 4⁄9 becomes 28⁄63 (4*7⁄9*7)
Step 4: Adding the Fractions
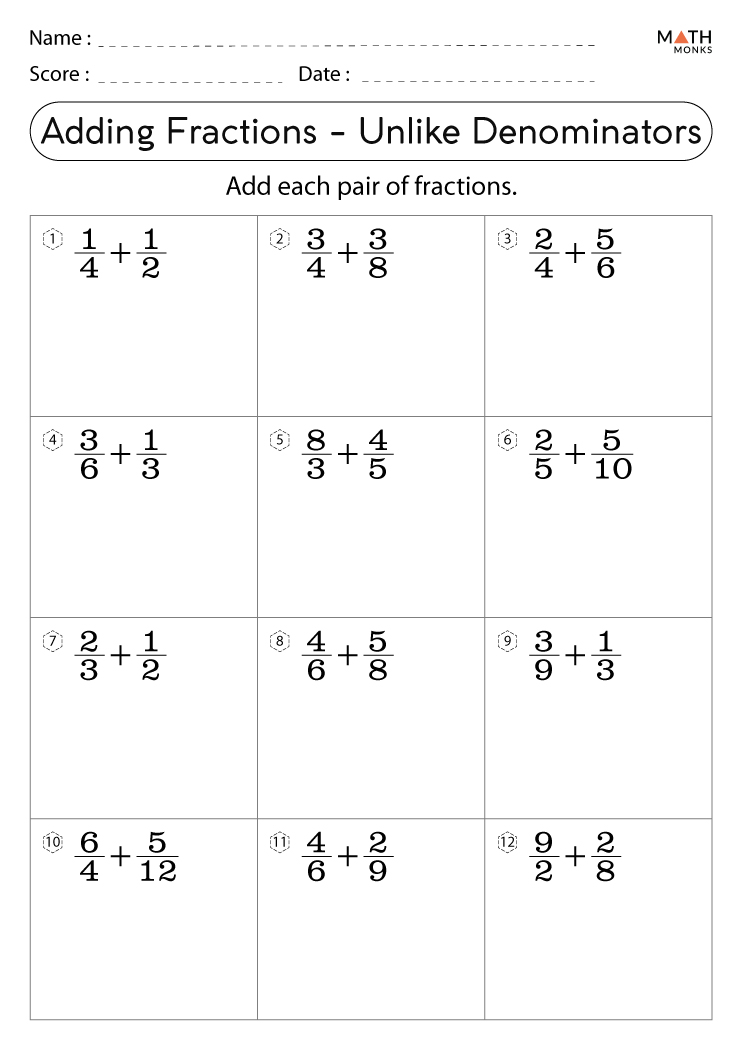
Now that the fractions have the same denominator, add the numerators:
- Add the new numerators, and place the result over the common denominator.
Following our examples:
- 5⁄15 + 6⁄15 = 11⁄15
- 27⁄63 + 28⁄63 = 55⁄63
💡 Note: When adding, if the sum of the numerators is greater than or equal to the denominator, you might need to simplify the fraction or convert it into a mixed number.
Step 5: Simplify if Necessary

Simplifying the result is the final touch to make your answer neat:
- Check if the numerator and the denominator have a common factor other than 1.
- If so, divide both by this common factor.
In the case of 11⁄15, no simplification is needed as 11 is a prime number and doesn’t share factors with 15. However, if you have 6⁄12, you can simplify it to 1⁄2 by dividing both by 6.
In wrapping up our journey through the addition of fractions, we’ve covered not only the mathematical technique but also the reasoning behind each step. These steps equip learners with the tools to tackle any adding fractions problem with confidence:
- Understanding the basics of what fractions represent and why they need the same base (denominator) for addition.
- Finding a common denominator which is essential for adding fractions.
- Converting those fractions into equivalent ones for seamless addition.
- Actually adding the numerators, now that the fractions are aligned.
- Simplifying to present the final answer in its most reduced form.
By applying these steps, students can turn the complexity of adding different fractions into a manageable and logical process. Practice with worksheets that incorporate various types of fractions can help reinforce these skills, making this mathematical concept second nature over time.
What if one of the fractions is a whole number?

+
Treat the whole number as a fraction with the same denominator as the common denominator you’ve found. For example, 2 can be written as 2⁄1 or as 6⁄3 if your common denominator is 3.
Why do we need a common denominator?

+
A common denominator is necessary because fractions represent parts of a whole, and you need to combine these parts accurately. If the denominators are different, you can’t compare or combine them directly.
Can I use a calculator to find the common denominator?

+
Yes, calculators can find the Least Common Multiple (LCM) of denominators, which can serve as the common denominator. This can be particularly useful for large numbers or when you’re learning the method.