5 Essential Trigonometry Worksheets With Answers
Trigonometry is often considered a challenging branch of mathematics, especially for students who are encountering its concepts for the first time. However, with the right tools and practice, mastering trigonometric functions becomes an achievable goal. In this comprehensive guide, we will explore five essential trigonometry worksheets with answers that can significantly enhance your understanding and proficiency in this area.
Worksheet 1: Understanding the Basics

The first step in mastering trigonometry involves understanding the basic principles and definitions. Here’s what you should focus on:
- Definition of Sine, Cosine, and Tangent: Knowing these fundamental ratios will pave the way for all further calculations.
- Unit Circle and Trigonometric Values: Use a unit circle to memorize the values of sine, cosine, and tangent for key angles.
- Converting Between Degrees and Radians: Understanding how to switch between these units is crucial for many trigonometric problems.
| Function | Definition | Key Values at 0°, 30°, 45°, 60°, 90° |
|---|---|---|
| Sine (sin) | Opposite/Hypotenuse | 0, 1/2, √2/2, √3/2, 1 |
| Cosine (cos) | Adjacent/Hypotenuse | 1, √3/2, √2/2, 1/2, 0 |
| Tangent (tan) | Opposite/Adjacent | 0, 1/√3, 1, √3, undefined |

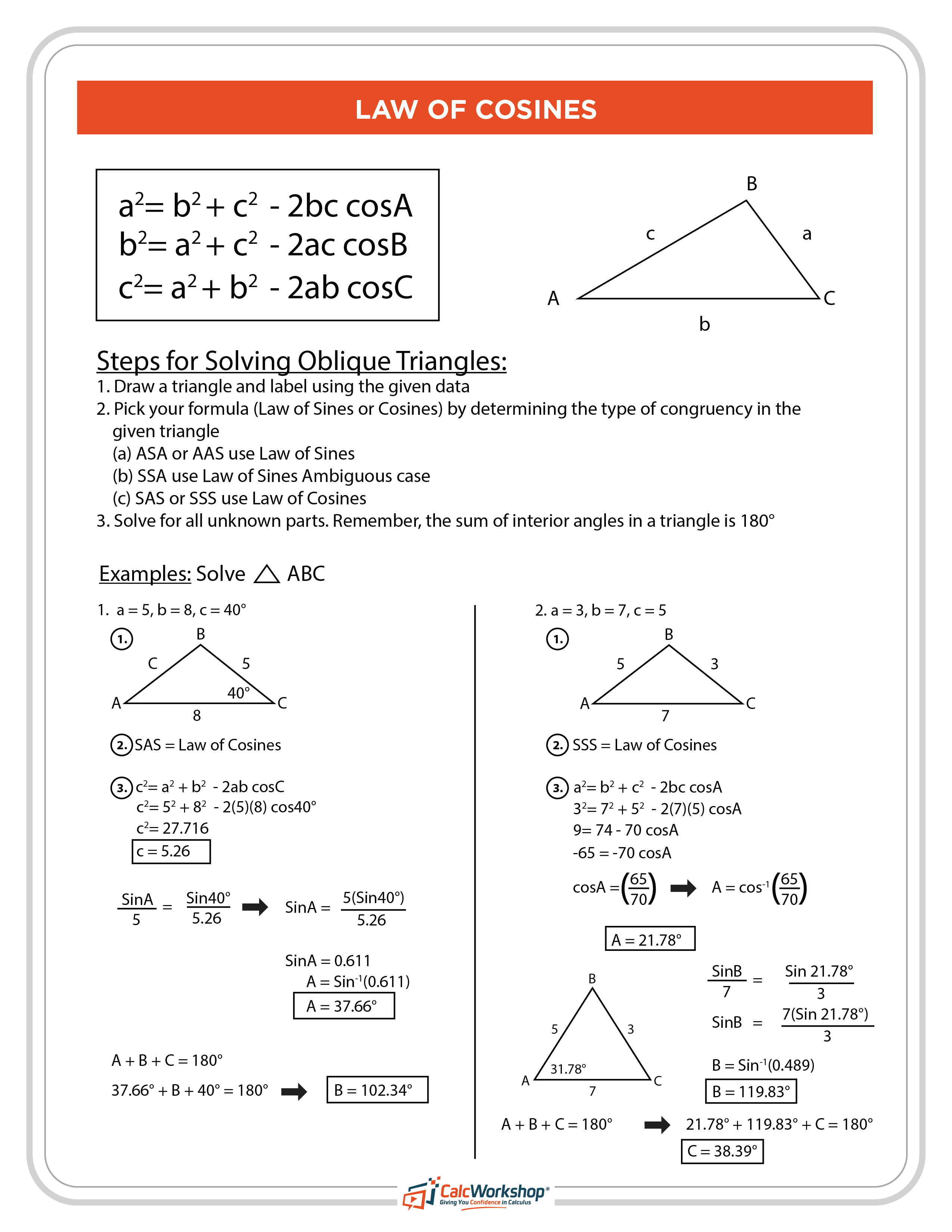
Worksheet 2: Solving Trigonometric Equations

After grasping the basics, you’ll need to apply this knowledge to solve equations. This worksheet focuses on:
- Solving for Angles: Use inverse trigonometric functions (sin⁻¹, cos⁻¹, tan⁻¹) to find unknown angles.
- Ambiguous Case: Handle cases where there might be more than one solution.
- Identities: Apply trigonometric identities to simplify and solve equations.
Example:

Find the value of x in the equation sin(x) = 1⁄2.
The answer would be x = 30° or x = 150° since sine is positive in the first and second quadrants.
💡 Note: Always consider the quadrant of the angle when solving trigonometric equations as it can lead to multiple solutions.
Worksheet 3: Trigonometric Functions and Their Graphs

Understanding how trigonometric functions behave graphically is crucial:
- Graphical Analysis: Learn to sketch and identify properties like amplitude, period, phase shift, and vertical shift.
- Transformations: Recognize how altering parameters affects the graph.
- Applications: Use graphs to solve real-world problems like oscillation in physics.
Here's a simple example:
Sketch the graph of y = 2 sin(x - π/4) + 1.
- Amplitude: 2
- Period: 2π
- Phase Shift: π/4 to the right
- Vertical Shift: Up by 1
Worksheet 4: Applications in Real Life

Trigonometry isn't just theoretical; it has practical applications:
- Architecture: Angles of elevation and depression to design structures.
- Navigation: Use bearings for travel direction.
- Engineering: Harmonic motion, signal processing.
Example Problem:

A surveyor needs to measure the height of a tree. From a point 20 feet away, the angle of elevation to the top of the tree is 35°. How tall is the tree?
Using tan(35°) = h/20, we get h ≈ 14.05 feet.
🧭 Note: The "height" in these calculations often refers to the vertical distance from the ground to the top of the structure in question.
Worksheet 5: Advanced Trigonometry

This worksheet delves into:
- Inverse Trigonometric Functions: Understanding their domain and range.
- Double and Half-Angle Formulas: Simplifying complex expressions.
- Product-to-Sum and Sum-to-Product Identities: For solving trigonometric equations involving products and sums.
Example:

Simplify sin(3x)cos(x) using product-to-sum identities.
We use the identity: sin(A)cos(B) = 1⁄2[sin(A+B) + sin(A-B)]
So, sin(3x)cos(x) = 1⁄2[sin(4x) + sin(2x)].
Summarizing the Journey

Throughout these five worksheets, we’ve covered essential aspects of trigonometry from basic concepts to advanced applications. The journey through trigonometry involves understanding the definitions, equations, graphs, and practical applications, each worksheet building on the knowledge from the previous. Remember, consistent practice with these problems will not only improve your problem-solving skills but also deepen your conceptual understanding, making you well-prepared for any mathematical challenge or exam involving trigonometry.
What are the primary trigonometric functions?

+
The primary trigonometric functions are sine (sin), cosine (cos), and tangent (tan). They are defined in terms of ratios of the sides of a right triangle: sine is opposite over hypotenuse, cosine is adjacent over hypotenuse, and tangent is opposite over adjacent.
How do I solve trigonometric equations?

+
To solve trigonometric equations, use the inverse trigonometric functions to find unknown angles, be mindful of multiple solutions, and apply identities to simplify the equations. Remember to check the quadrant for each potential solution.
What are some practical applications of trigonometry?

+
Trigonometry is used in various fields like architecture for designing slopes and angles, navigation for determining distances and bearings, and in engineering for analyzing oscillatory systems and signal processing.