Unlock the Power: Kinetic Energy Worksheet Explained
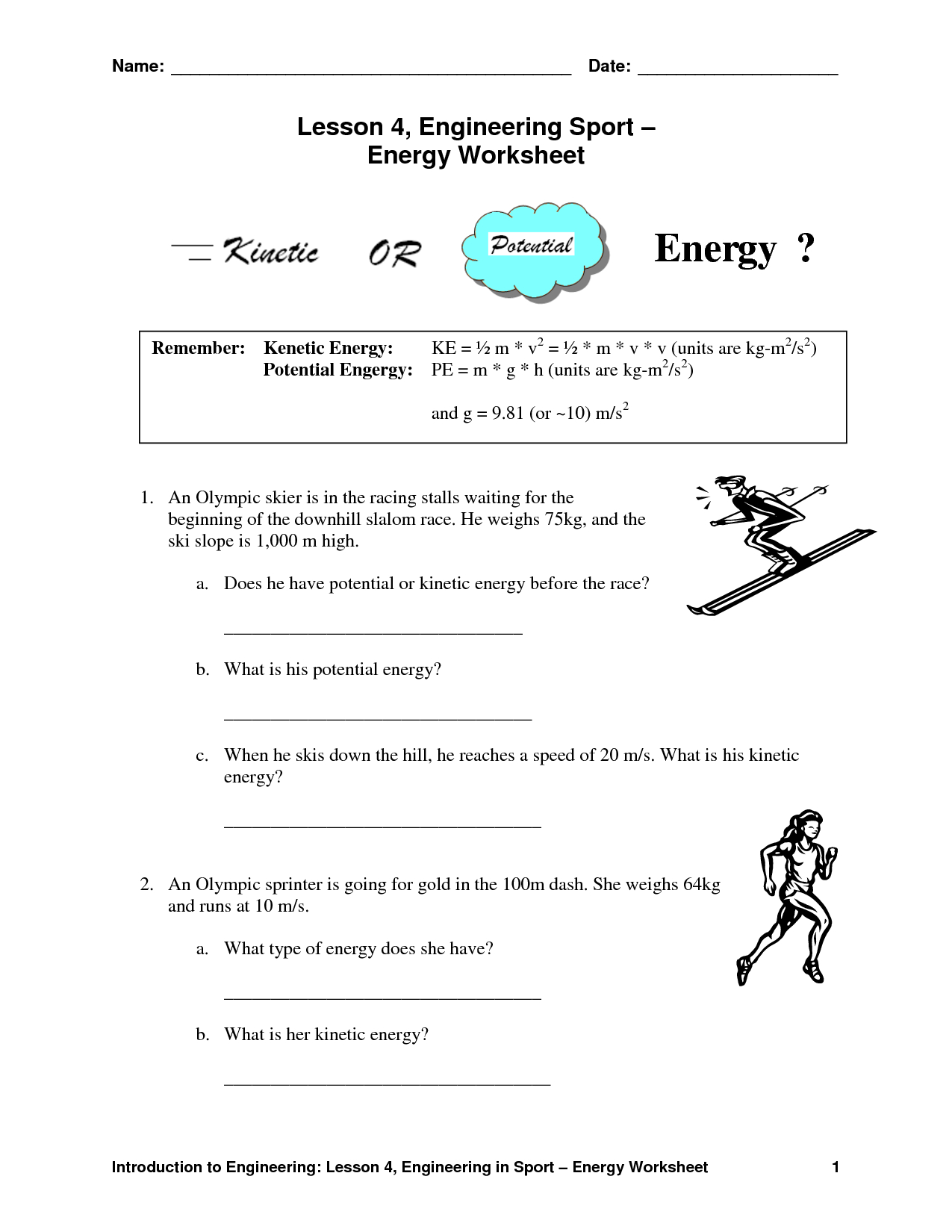
Unlocking the power of kinetic energy involves understanding the fundamental principles that govern how objects move and interact with their environment. Whether you're a student struggling with physics concepts or a curious individual looking to deepen your knowledge of how the world works, this comprehensive guide on kinetic energy worksheets will help illuminate the subject. Let's delve into what kinetic energy is, how it's calculated, and explore practical examples through worksheets designed to enhance comprehension and application.
What is Kinetic Energy?

Kinetic energy (KE) is the energy an object possesses due to its motion. It's a scalar quantity, meaning it has magnitude but no direction. The formula to calculate kinetic energy is:
\[ KE = \frac{1}{2}mv^2 \]
where:
- m is the mass of the object (measured in kilograms, kg),
- v is the velocity of the object (measured in meters per second, m/s).
Worksheet Examples: Understanding Kinetic Energy

Worksheet 1: Basic Calculation

Consider the following problem:
A 2 kg ball is moving at a speed of 4 m/s. Calculate its kinetic energy.
Answer:
Using the formula KE = \frac{1}{2}mv^2 , we get:
\[ KE = \frac{1}{2} \times 2 \times 4^2 = 16 \text{ joules} \]
Worksheet 2: Work and Kinetic Energy Theorem

The work-kinetic energy theorem states that the work done on an object equals its change in kinetic energy. Here’s an example:
A force of 10 N is applied to push a 3 kg block, accelerating it from rest to a velocity of 5 m/s. How much work was done by the force?
Answer:
- Initial kinetic energy (KE_i): 0 (since the object was at rest).
- Final kinetic energy (KE_f): \frac{1}{2} \times 3 \times 5^2 = 37.5 \text{ joules}
- The work done: W = KE_f - KE_i = 37.5 \text{ joules}
Worksheet 3: Conservation of Kinetic Energy

In ideal conditions, kinetic energy can be conserved during interactions like elastic collisions. Consider:
Two identical billiard balls each with a mass of 0.16 kg, one moving at 5 m/s collides head-on with the second ball at rest. After the collision, the first ball stops, and the second ball moves forward. Calculate the velocity of the second ball post-collision.
Answer:
Given that kinetic energy is conserved:
- The initial kinetic energy of the first ball: KE_{initial} = \frac{1}{2} \times 0.16 \times 5^2 = 2 \text{ joules}
- The second ball moves with the same energy, so its velocity can be found:
\[ 2 = \frac{1}{2} \times 0.16 \times v^2 \rightarrow v^2 = \frac{2}{0.08} \rightarrow v = \sqrt{25} \approx 5 \text{ m/s}\]
⚠️ Note: This assumes an elastic collision where no energy is lost to sound, heat, or deformation.
Advanced Concepts in Kinetic Energy

Energy Transfer in Systems
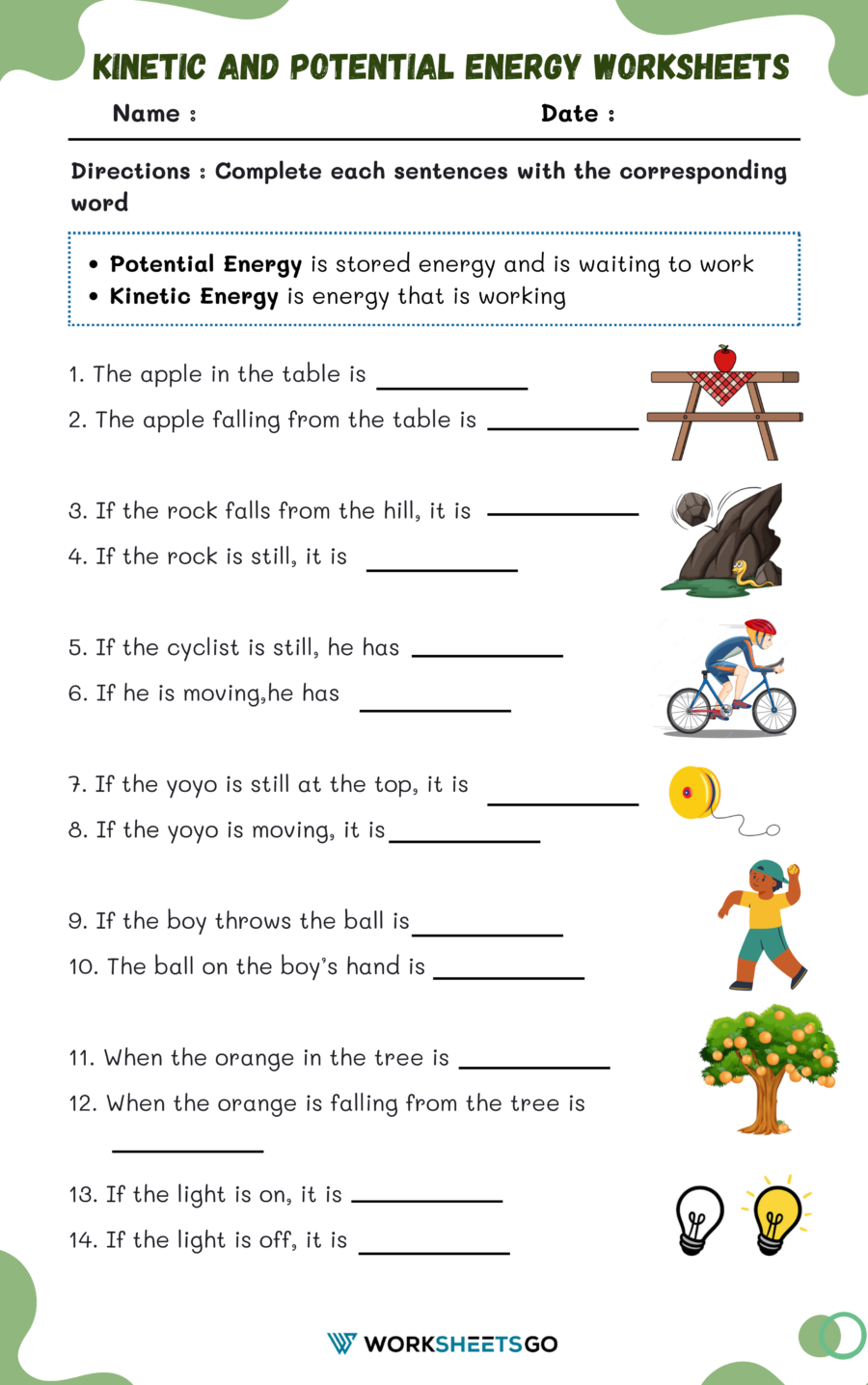
In more complex systems, kinetic energy can be transferred to potential energy or dissipated as heat or sound. Here are key points:
- When a roller coaster climbs a hill, its kinetic energy is converted into gravitational potential energy.
- During the descent, this potential energy is converted back into kinetic energy, accounting for energy loss due to friction.
Momentum and Energy

Understanding the interplay between momentum and kinetic energy helps in analyzing:
- Conservation of momentum in all types of collisions.
- Energy conservation, primarily in elastic collisions.
🌟 Note: The conservation of momentum can always be applied to physical systems, even when kinetic energy is not conserved.
Applications of Kinetic Energy in Daily Life

Kinetic energy isn’t just a theoretical concept; it’s fundamental in various practical applications:
- Safety: Airbags and crumple zones in vehicles absorb kinetic energy to protect passengers during collisions.
- Sports: The physics of how objects (like a football) move through the air, involving both kinetic and potential energy.
- Alternative Energy: Wind turbines and hydroelectric dams convert kinetic energy into electrical energy.
In summarizing, the exploration of kinetic energy through worksheets provides a solid foundation for understanding this crucial aspect of physics. Kinetic energy worksheets facilitate the learning process by offering practical, hands-on exercises that bridge theoretical knowledge with real-world applications. They not only help in reinforcing the understanding of the kinetic energy formula and principles but also encourage critical thinking, problem-solving, and visualization of physical concepts in action.
Why is kinetic energy important in physics?

+
Kinetic energy is fundamental because it describes how objects move and interact. It’s integral for understanding energy transfers, motion, and many physical phenomena like momentum, work, and collisions.
How can I calculate kinetic energy?

+
You can calculate kinetic energy using the formula ( KE = \frac{1}{2}mv^2 ), where m is the mass in kg and v is the velocity in m/s.
What’s the difference between potential and kinetic energy?

+
Kinetic energy is the energy of motion, while potential energy is stored energy that has the potential to do work. For example, a raised weight has gravitational potential energy, which can be converted into kinetic energy as it falls.
Can kinetic energy be negative?

+
No, kinetic energy cannot be negative as it is defined by the square of velocity, which is always positive, thus ensuring KE is always non-negative.
How does kinetic energy relate to safety in vehicles?

+
During a collision, the kinetic energy of moving vehicles must be dissipated. Car safety features like airbags and crumple zones are designed to absorb or redirect this kinetic energy safely, reducing the impact on passengers.



