Key Features of Algebra 2 Functions Explained

Algebra 2 introduces a variety of functions that are pivotal for understanding higher mathematics. From exploring quadratic functions to delving into the complexities of rational and trigonometric functions, Algebra 2 functions expand our toolkit for solving complex problems. This comprehensive guide will dive into the key features of these functions, providing clarity and practical insights for students and educators alike.

Quadratic Functions

Quadratic functions are a fundamental part of Algebra 2. They are represented by equations of the form:

y = ax² + bx + c

Here are the key features:

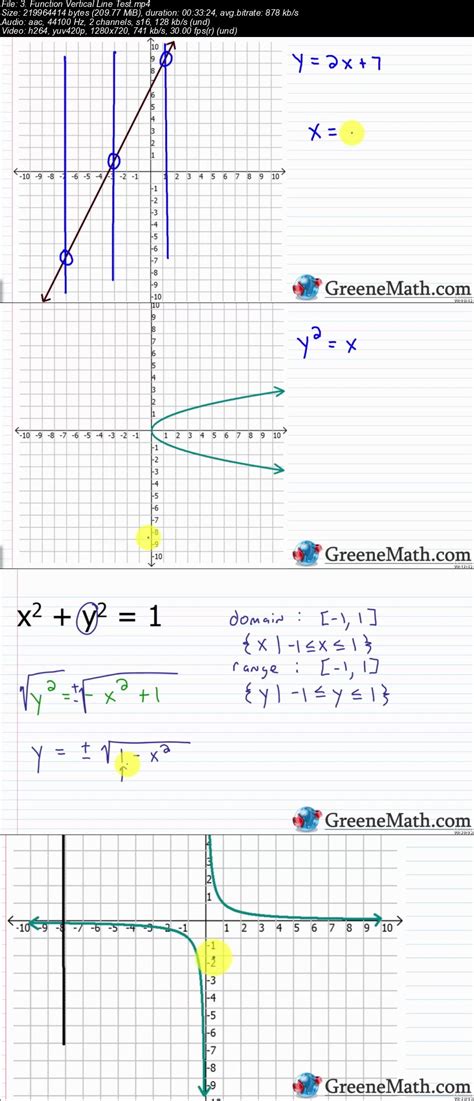
• Parabola Shape: Quadratic functions graph as parabolas, which can open upwards or downwards.
• Vertex: The vertex represents the lowest or highest point of the parabola, where the function has a minimum or maximum value, respectively. The vertex formula is given by x = -b/2a.
• Axis of Symmetry: This is a vertical line that divides the parabola into two mirror images. It passes through the vertex with the equation x = -b/2a.
• Roots: The points where the parabola intersects the x-axis, also known as zeros or solutions, can be found using the quadratic formula: x = [-b ± √(b² - 4ac)]/2a.

💡 Note: Always remember the “shape” of a quadratic function: a positive a-value leads to an upward parabola, while a negative a-value leads to a downward parabola.

Polynomial Functions

Polynomial functions include quadratic functions as a subset but go beyond. They are of the form:

f(x) = a_nxⁿ + a_n-1x^n-1 + … + a₁x + a₀

Key features:

• Degree: The highest power of x is the degree of the polynomial, which determines the number of potential roots or turning points.
• End Behavior: Polynomial functions have unique end behavior based on their degree and leading coefficient. For example, an odd degree polynomial will have opposite end behaviors, while an even degree will have the same behavior.
• Roots and Multiplicities: Polynomial roots can be real or complex, and their multiplicities affect the behavior of the function near those points.

Rational Functions

Rational functions are the ratio of two polynomials, given by:

f(x) = P(x) / Q(x)

Key points to understand:

• Vertical Asymptotes: Occur at values of x where the denominator is zero (and the numerator is not).
• Horizontal or Oblique Asymptotes: As x approaches ±∞, rational functions can approach a horizontal line or, if the degree of the numerator exceeds that of the denominator by exactly one, an oblique (slant) line.
• Intercepts: Rational functions have x-intercepts where the numerator is zero and y-intercepts where x = 0 (unless the function has a vertical asymptote at x = 0).
• Holes: If a factor in the numerator and denominator cancel out, there will be a hole in the graph at that x-value.

Exponential Functions

Exponential functions, where the variable x is in the exponent:

f(x) = ab^x

• Base and Growth Factor: a represents the initial amount, and b is the base or growth factor. If b > 1, the function grows; if 0 < b < 1, it decays.
• End Behavior: As x approaches ±∞, the function either grows without bound or decays to zero.
• Domain and Range: The domain is all real numbers, but the range depends on the base b. If b > 1, range is y > 0; if 0 < b < 1, range is 0 < y < 1.

Logarithmic Functions

The inverse of exponential functions, logarithmic functions:

f(x) = log_b(x)

Important features:

• Vertical Asymptote: At x = 0, where the function is undefined.
• Domain: x > 0 (logarithms of negative numbers are undefined in real number system).
• Base: The base b determines the rate at which the function grows or decays.

Trigonometric Functions

Trigonometric functions like sine, cosine, and tangent are periodic in nature:

• Periodicity: Functions like sin(x) and cos(x) repeat their behavior every 360° or 2π radians.
• Amplitude: This is the maximum deviation from the x-axis. For sin(x) and cos(x), it’s 1.
• Phase Shift: A horizontal shift in the function graph, denoted as “c” in the general form f(x) = a * sin(bx + c) + d.
• Vertical Shift: The “d” in the same form indicates the vertical shift.

⚠️ Note: Understanding the unit circle is crucial for trigonometric functions as it provides visual insight into their periodicity and behavior.

In summary, Algebra 2 functions come with unique features that form the building blocks for further mathematical study. From quadratic equations to trigonometric functions, each has distinct properties that students must understand for problem-solving and real-world applications. Remember, these functions don’t exist in isolation; their interplay forms the core of mathematical analysis and modeling in higher education and beyond.

What are the practical uses of Algebra 2 functions?

+

Algebra 2 functions are not only theoretical constructs but also have numerous real-world applications in fields like engineering, finance, physics, and more. For example, quadratic functions can model the flight path of projectiles, exponential functions can be used to understand population growth, and trigonometric functions are essential in understanding oscillations and wave behaviors.

How can I tell if a function is odd or even?

+

An even function satisfies f(-x) = f(x), meaning the function’s graph is symmetric about the y-axis. An odd function satisfies f(-x) = -f(x), meaning it’s symmetric about the origin. Determining this involves substituting -x into the function and comparing the result with f(x).

Why are asymptotes important in rational functions?

+

Asymptotes help us understand the function’s behavior as the input (x) approaches certain values. Vertical asymptotes indicate where the function approaches infinity, which is where the denominator equals zero. Horizontal and oblique asymptotes show what happens as x approaches ±∞, providing insight into the function’s growth or decay rate.