Improper Fractions Worksheet for Math Practice
Understanding and Working with Improper Fractions
Improper fractions are a fundamental concept in mathematics, and mastering them is essential for advanced math operations. In this article, we will delve into the world of improper fractions, explore their characteristics, and provide a comprehensive worksheet for practice.
What are Improper Fractions?
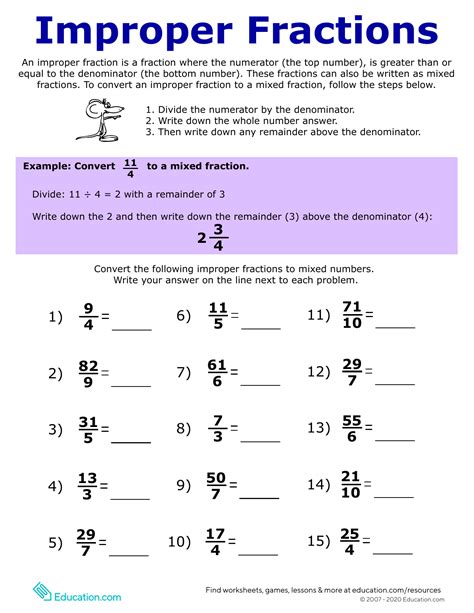
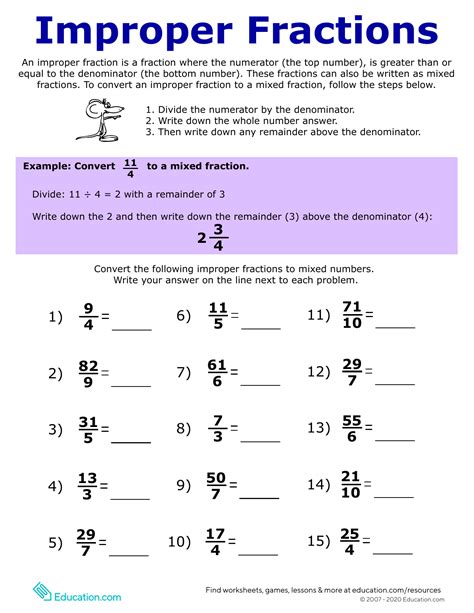
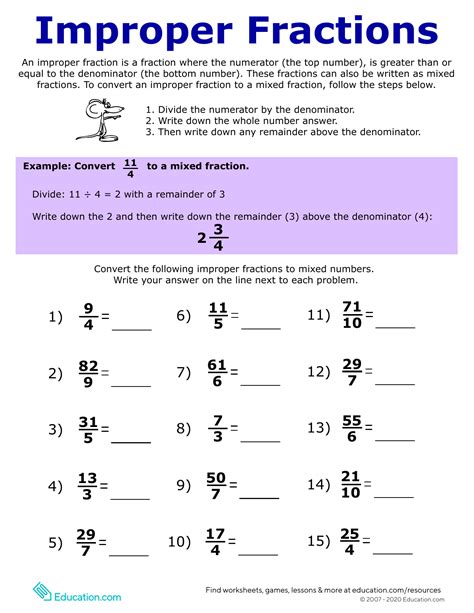
An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). This type of fraction is called “improper” because it does not follow the traditional rule of fractions, where the numerator is always less than the denominator.
Examples of Improper Fractions

Here are a few examples of improper fractions:
- 3⁄2
- 5⁄3
- 7⁄4
- 9⁄6
As you can see, the numerator in each of these fractions is greater than or equal to the denominator.
Converting Improper Fractions to Mixed Numbers
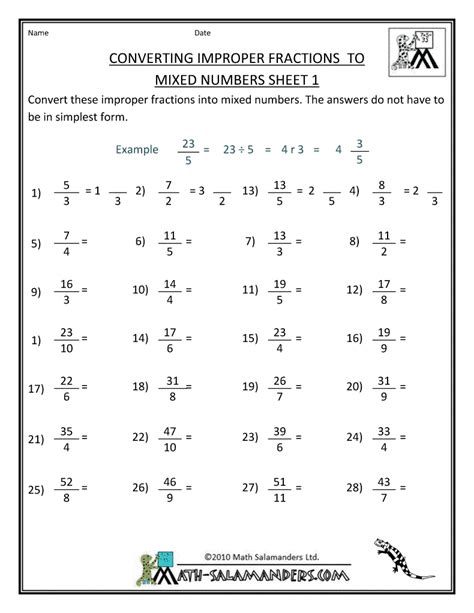
One way to simplify improper fractions is to convert them to mixed numbers. A mixed number is a combination of a whole number and a proper fraction. To convert an improper fraction to a mixed number, divide the numerator by the denominator and write the remainder as a proper fraction.
For example, let’s convert the improper fraction 3⁄2 to a mixed number:
- Divide 3 by 2: 3 ÷ 2 = 1 with a remainder of 1
- Write the remainder as a proper fraction: 1⁄2
- Combine the whole number and the proper fraction: 1 1⁄2
Worksheet: Practicing Improper Fractions

Now that we have a solid understanding of improper fractions, it’s time to practice! Here’s a comprehensive worksheet to help you master this concept:
Section 1: Simplifying Improper Fractions
Simplify each of the following improper fractions:
| Improper Fraction | Simplified Form |
|---|---|
| 4⁄3 | |
| 7⁄2 | |
| 9⁄5 | |
| 11⁄6 |

Section 2: Converting Improper Fractions to Mixed Numbers
Convert each of the following improper fractions to a mixed number:
| Improper Fraction | Mixed Number Form |
|---|---|
| 5⁄2 | |
| 3⁄4 | |
| 7⁄3 | |
| 9⁄2 |
Section 3: Word Problems
Solve each of the following word problems involving improper fractions:
- A recipe calls for 3⁄2 cups of flour. If you only have a 1⁄4 cup measuring cup, how many times will you need to fill it to get the required amount of flour?
- A bookshelf has 5⁄3 feet of space available for books. If you want to place 2 books on the shelf, and each book takes up 1⁄2 foot of space, how much space will be left over?
- A bike ride is 7⁄2 miles long. If you ride at a speed of 2 miles per hour, how many hours will it take you to complete the ride?
Section 4: Challenge Problems
Solve each of the following challenge problems involving improper fractions:
- Simplify the improper fraction 11⁄4.
- Convert the improper fraction 9⁄2 to a mixed number.
- Solve the equation 3⁄2 + 2⁄3 =?
🤔 Note: Remember to simplify your answers and check your work carefully.
What is an improper fraction?

+
An improper fraction is a fraction where the numerator is greater than or equal to the denominator.
How do I simplify an improper fraction?

+
To simplify an improper fraction, divide the numerator by the denominator and write the remainder as a proper fraction.
Can I convert an improper fraction to a mixed number?
+
Yes, you can convert an improper fraction to a mixed number by dividing the numerator by the denominator and writing the remainder as a proper fraction.
In conclusion, improper fractions are a crucial concept in mathematics, and mastering them is essential for advanced math operations. With this worksheet, you’ll be well on your way to becoming proficient in working with improper fractions. Remember to practice regularly and challenge yourself with more complex problems to reinforce your understanding.
Related Terms:
- Improper fractions worksheet pdf
- Improper fractions worksheet with answers