Mastering Absolute Value Equations and Inequalities with Ease

Understanding absolute value equations and inequalities can often feel like navigating a mathematical labyrinth. However, with the right strategies and understanding, you can solve these problems with surprising ease. This guide will walk you through what absolute value is, how it applies in equations and inequalities, and provide you with practical steps to master these concepts.
What is Absolute Value?

At its core, absolute value represents the distance a number is from zero on the number line, disregarding its sign. Symbolized by vertical bars (| |), the absolute value of a number (x), denoted as (|x|), is always non-negative, meaning:
- (|x| = x) if (x) is non-negative
- (|x| = -x) if (x) is negative
💡 Note: Absolute value measures magnitude or size, not direction.
Solving Absolute Value Equations
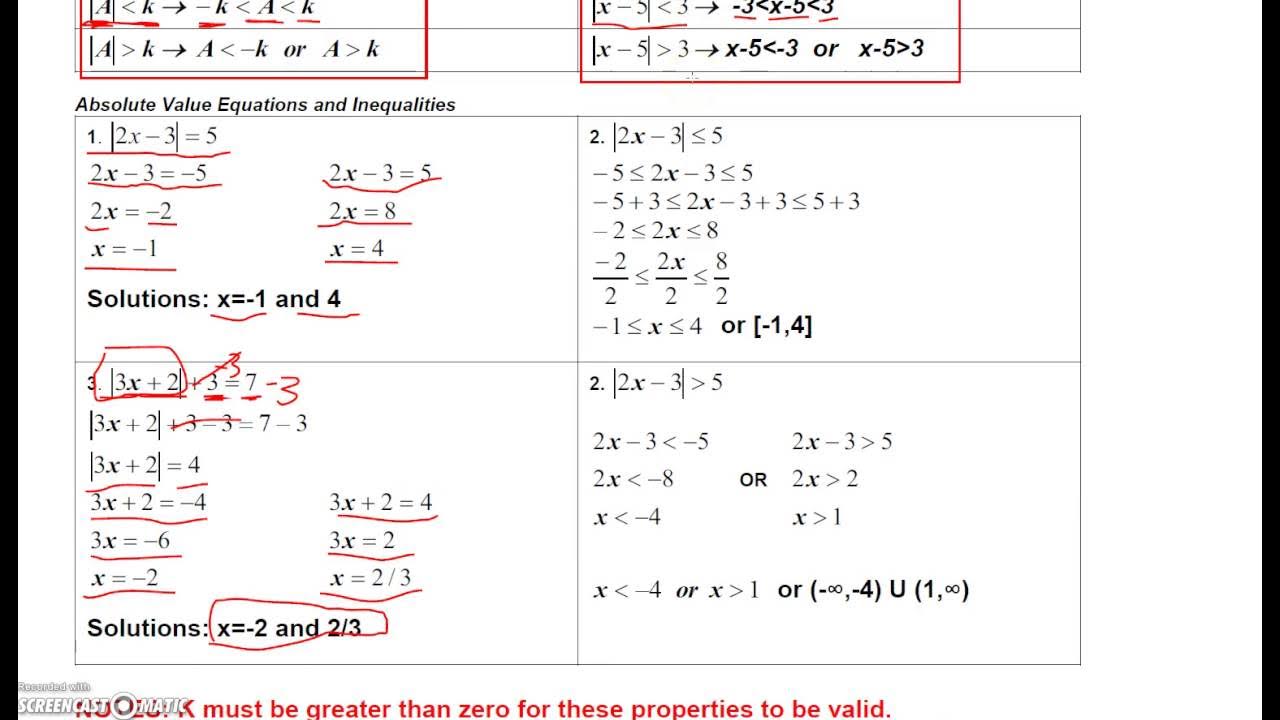
When dealing with absolute value equations, such as (|ax + b| = c), here are the steps to solve them:
- Set up two equations:
- ax + b = c
- ax + b = -c
- Solve each equation separately: Treat these as standard linear equations now.
- Find the intersection: Look for the intersection of your solutions to ensure they are valid.
Here’s an example:
| Equation | Solution |
|---|---|
| |2x - 3| = 7 | Leads to:
|
🔍 Note: When solving, remember that the absolute value equation might have two solutions, one on either side of zero.
Dealing with Absolute Value Inequalities

Solving absolute value inequalities involves considering the values for which the absolute value is less than, greater than, or equal to a certain number. Here’s how you handle each case:
- Less than or equal to (≤):
- |ax + b| \le c
- Split this into: -c \le ax + b \le c
- Solve both inequalities simultaneously.
- Greater than or equal to (≥):
- |ax + b| \ge c
- Split this into: ax + b \le -c or ax + b \ge c
- Solve both inequalities separately, considering the union of the solutions.
Let's look at an example for each:
Example for ≤

(|x + 2| \le 4)
| Equation | Solutions |
|---|---|
| |x + 2| \le 4 |
|
Example for ≥
(|x - 3| \ge 5)
| Equation | Solutions |
|---|---|
| |x - 3| \ge 5 |
|
📝 Note: Always consider the direction of the inequality when converting from absolute value form to compound inequalities.
Key Tips for Mastering Absolute Value Problems

- Understand the concept: Absolute value deals with distance from zero.
- Visualization: Use number lines to visualize where solutions lie.
- Practice: Regular practice will help you quickly recognize the different scenarios.
- Remember compound inequalities: For less than or equal to inequalities, think about the number between two extremes. For greater than or equal to, think about the numbers outside two extremes.
- Check your work: Substitute your solutions back into the original problem to verify accuracy.
In summary, mastering absolute value equations and inequalities involves understanding the core concept of distance from zero, using structured methods to solve the equations, and practicing regularly. With these strategies, you'll find yourself adept at solving these once-intimidating problems. Absolute value is not just a mathematical concept; it's a tool for solving real-world problems involving distances and magnitudes.
Why do we need to consider the negative case when solving absolute value equations?

+
Absolute value measures distance from zero, so the positive and negative values inside the bars represent the same distance but in opposite directions. Including both cases ensures we account for all possible distances.
How can I visualize absolute value inequalities?

+
Draw a number line, place your “center” point (the value inside the absolute value), and consider the distances less than, greater than, or equal to the given value.
What if there are absolute values on both sides of the equation?

+
Treat each absolute value as its own entity, solving for both the positive and negative cases of each side separately. Then, find where these cases overlap or match to solve the equation.
Can absolute value problems ever have no solutions?
+
Yes, if the conditions set by the absolute value cannot be satisfied (e.g., (|x| = -3)), then there are no solutions since absolute values cannot be negative.
How do absolute values relate to real-world problems?

+
Absolute value can represent anything from measuring distances to calculating differences in time or temperature where direction or sign does not matter.