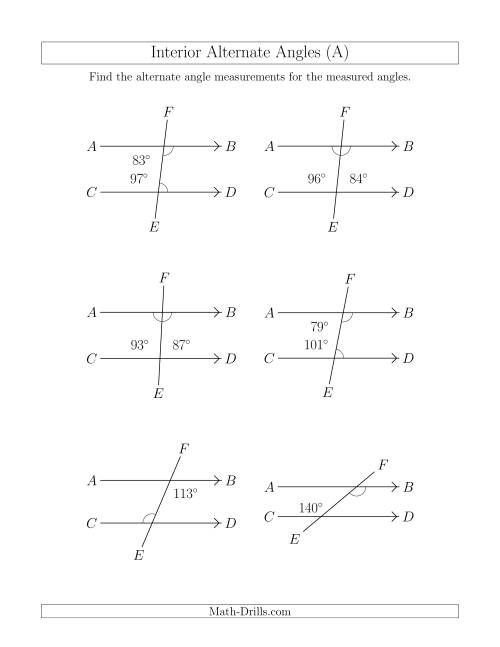
Master Slope Intercept Form with These Worksheets

Understanding and mastering the Slope Intercept Form is fundamental for students delving into algebra. This form, expressed as y = mx + b, provides a straightforward way to graph lines, understand slopes, and intercepts in a linear equation. If you're a student, teacher, or just someone brushing up on math, slope-intercept worksheets can be an invaluable tool in your learning journey. Here's how you can use these worksheets to elevate your grasp on this essential math concept.
Why Use Slope-Intercept Form Worksheets?

The slope-intercept form is not just about memorizing a formula; it’s about understanding the relationships between variables in a linear equation:
- Visualization: It helps in visualizing lines on a graph.
- Analysis: Understanding how changes in m (slope) and b (y-intercept) affect the line’s behavior.
- Practice: Consistent practice through worksheets reinforces the understanding of algebraic concepts.
Getting Started with Slope-Intercept Worksheets

Here’s how to begin:
- Identify a good set of worksheets that cover various aspects of slope-intercept form.
- Look for resources that offer:
- Step-by-step problem-solving
- Graphical representation exercises
- Practice with both positive and negative slopes
- Application problems to real-world scenarios
- Start with basic problems and gradually move to complex scenarios.
Steps to Work Through Slope-Intercept Problems

1. Understand the Formula:

y = mx + b where:
- y is the y-coordinate
- m is the slope
- x is the x-coordinate
- b is the y-intercept
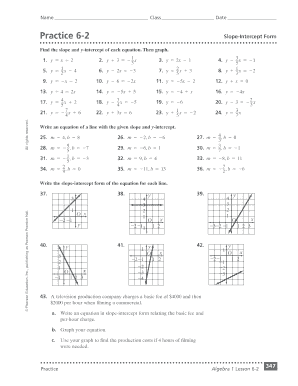
2. Identify Slope and Y-Intercept:

Worksheets often require you to:
- Identify the slope from given points or a graph.
- Determine the y-intercept from an equation or visual representation.
3. Graph the Equation:

Practice plotting lines by:
- Using the y-intercept as your starting point.
- Applying the slope to extend the line in both directions.
4. Solve for Specific Points:

Here are some typical worksheet problems:
- Find the x-intercept (where y = 0).
- Calculate the y-value for a given x-value.
5. Transform Equations:
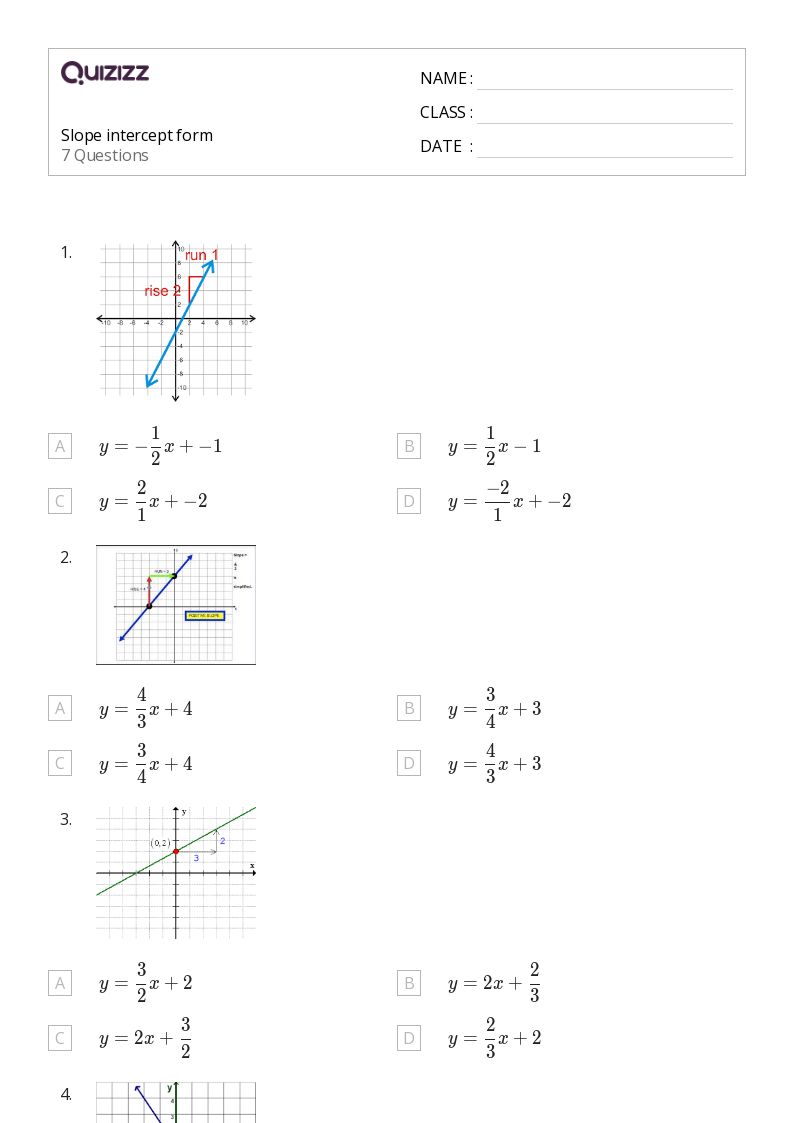
Worksheets may include exercises to convert:
- Other forms of linear equations to slope-intercept form.
- Find parallel or perpendicular lines.
6. Application Problems:

Advanced worksheets often ask you to:
- Solve word problems that require you to form and then interpret slope-intercept equations.
- Analyze data to determine lines of best fit.
Key Elements of Effective Worksheets
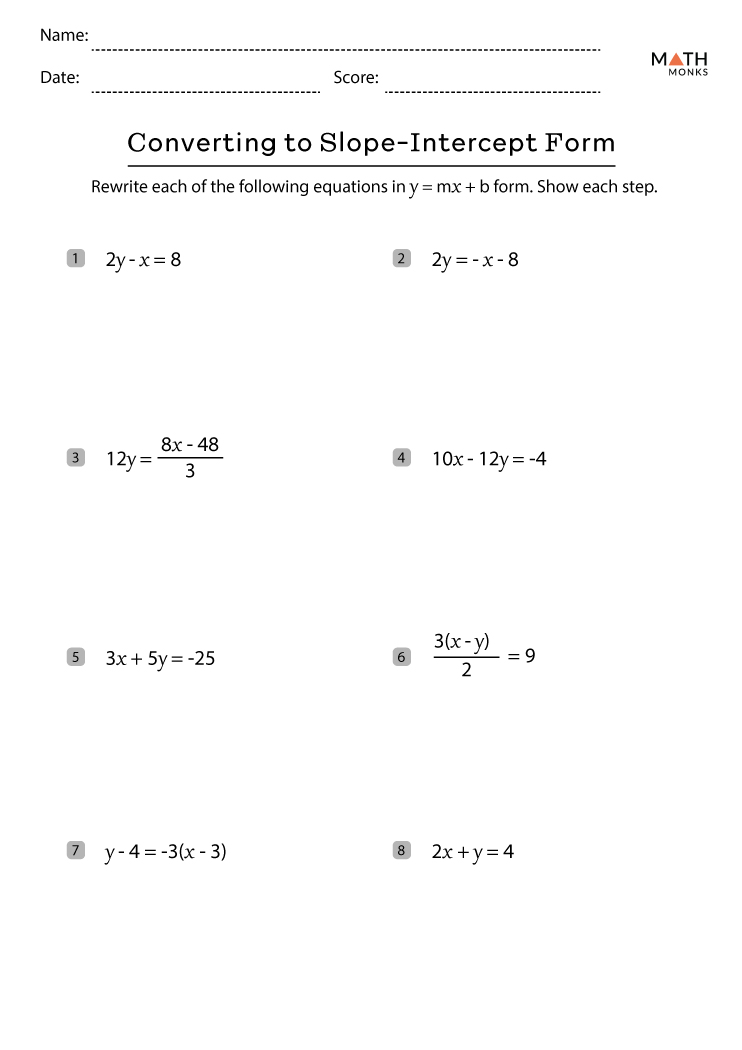
When selecting or creating worksheets, look for:
- Clear Instructions: Each problem should have explicit directions.
- Variety of Exercises: A mix of graph plotting, equation solving, and real-life applications.
- Gradual Difficulty: Problems should increase in complexity as you progress.
- Visual Aids: Graphs, grids, and pictures to aid in visualization.
Practical Tips for Working Through Slope-Intercept Worksheets

To optimize your learning:
- Always start with the basics, even if you feel proficient.
- Use a calculator for computations but ensure you understand the underlying math.
- Color-code graphs for different equations to better track multiple lines.
- Discuss and work through problems with peers or tutors.
💡 Note: Using color-coded pens or pencils can make graphs clearer and easier to interpret.
FAQ
How do you find the slope of a line from a graph?

+
To find the slope from a graph, pick two points on the line. The slope m is calculated by the change in y (rise) divided by the change in x (run): m = (y₂ - y₁) / (x₂ - x₁).
Can you graph a line when only given the y-intercept and slope?

+
Yes, you can start at the y-intercept (where x = 0) and move from there. For example, if the slope is 2, for every unit you move to the right, you move up 2 units. If the slope is negative, you’ll move down instead.
Why do we use the slope-intercept form?

+
The slope-intercept form, y = mx + b, makes it easy to understand the slope (m) and the y-intercept (b) of a line, which are essential properties for graphing and analyzing linear relationships.
Ultimately, mastering the slope-intercept form through focused practice with well-designed worksheets can significantly enhance your understanding and application of linear equations. These exercises not only solidify the core concepts of algebra but also empower you to tackle more complex mathematical challenges with confidence. Whether you’re studying for an exam, helping others learn, or just satisfying your curiosity about math, the journey through slope-intercept form is both practical and rewarding.