Master Graphing Lines in Slope-Intercept Form Easily
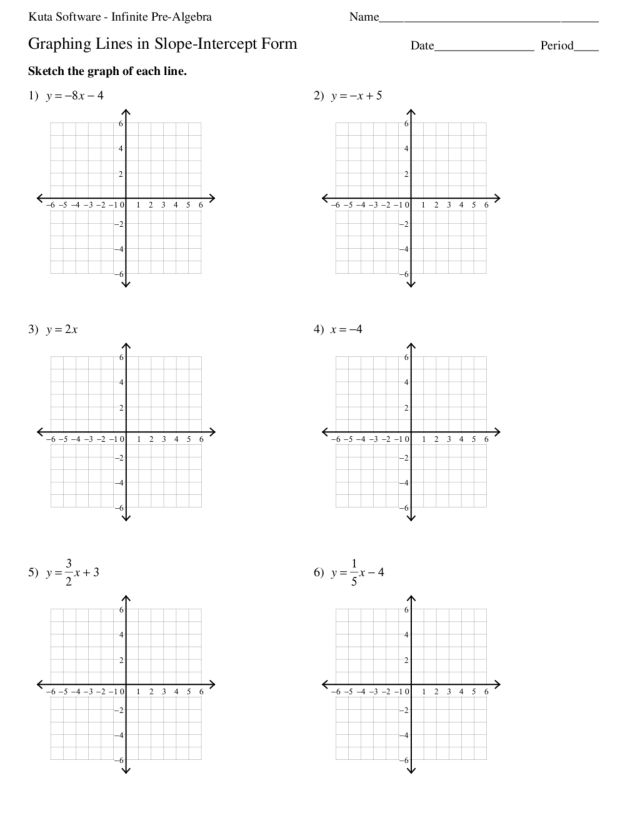
Graphing lines is a fundamental skill in algebra and beyond, with applications in economics, engineering, physics, and much more. One of the most straightforward methods for this task is through the slope-intercept form, which provides a clear and intuitive way to visualize lines on the coordinate plane. In this post, we'll break down how to easily master graphing lines using the slope-intercept form, step by step.
Understanding Slope-Intercept Form

The slope-intercept form of a line’s equation is:
y = mx + b
Where:
- m represents the slope of the line (how steep the line is).
- b represents the y-intercept (the point where the line crosses the y-axis).
Step-by-Step Guide to Graphing

Here’s how to graph a line using the slope-intercept form:
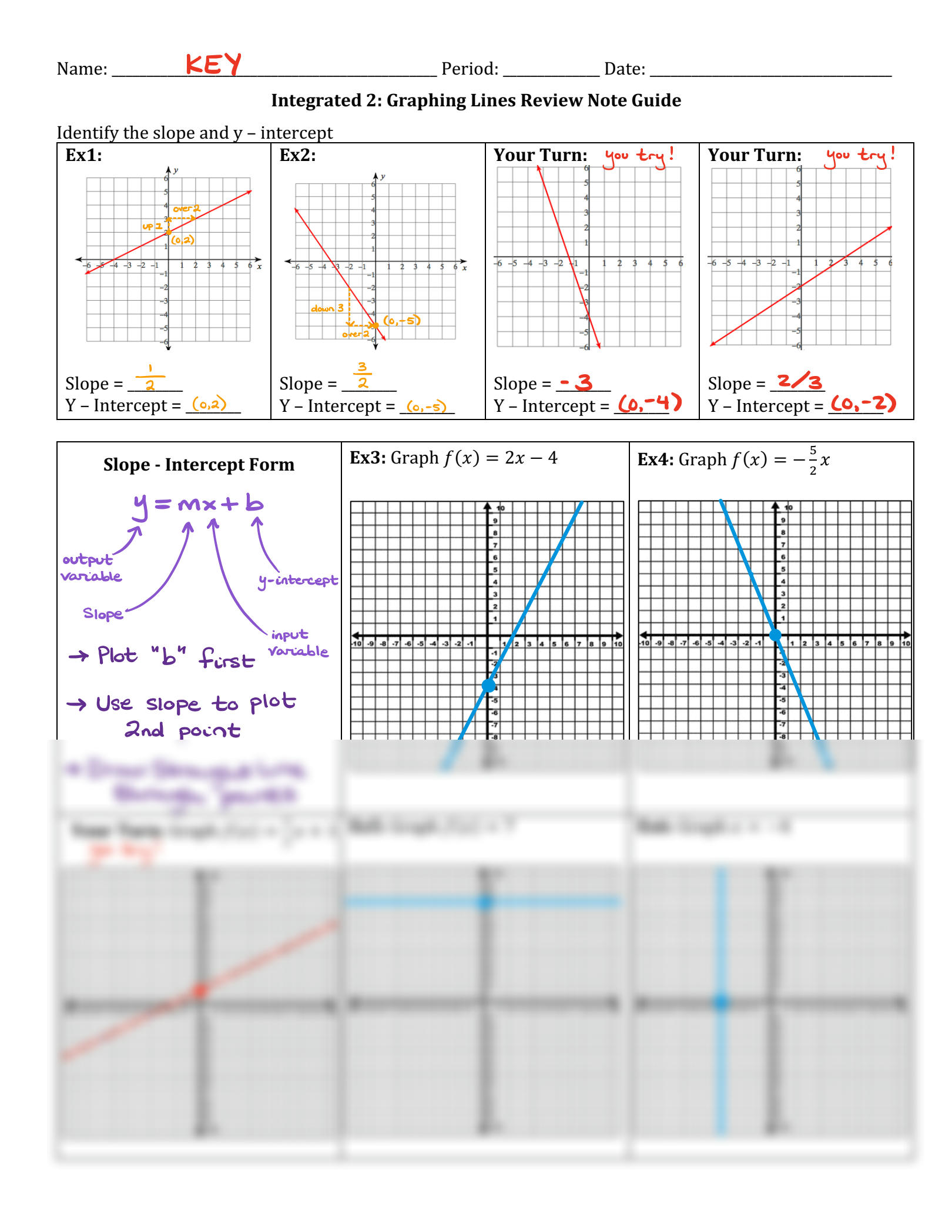
Identify the Slope and Y-Intercept

From your equation, determine the values of m and b. For example, if your equation is:
y = 2x + 3
- m = 2 (slope)
- b = 3 (y-intercept)
Plot the Y-Intercept

Locate the y-intercept on your coordinate plane. For our example, you would mark a point at (0, 3). This point represents where the line crosses the y-axis.
Determine the Slope’s Direction

The slope tells you how the line will ascend or descend from the y-intercept:
- If m is positive, the line will rise from left to right.
- If m is negative, the line will fall from left to right.
Plot Additional Points

Using the slope:
- From the y-intercept, move right by 1 unit (for the run) and then:
- Up by m units for positive slopes.
- Down by m units for negative slopes.
- Let’s take our example: Starting from (0, 3), move right 1 unit and up 2 units. This gives us the point (1, 5).
Draw the Line

After plotting your second point, use a ruler to draw a line through these two points, extending it in both directions. This line should pass through both the y-intercept and the newly plotted point.
Practice with an Example

Let’s graph another line with the equation:
y = -1/2x + 4
- m = -1⁄2
- b = 4
Here’s how it looks on the graph:

📝 Note: Ensure your graph covers a reasonable range to show both the slope and y-intercept accurately.
Tips for Complex Slopes

Not all slopes are integers or simple fractions. Here’s how to handle them:
- For a slope like m = -3⁄4, move right by 4 units (run) and down by 3 units (rise) to plot another point.
- Use a grid paper to facilitate this plotting, especially with irrational numbers or complex slopes.
Applications in Real Life

Understanding how to graph lines can be incredibly useful in:
- Physics: To plot the path of projectiles or the rate of acceleration.
- Economics: To represent supply and demand curves, or to forecast trends.
- Engineering: In designing and analyzing structures or systems with linear relationships.
By following these steps and practicing, you'll find that graphing lines becomes second nature. Each time you graph, you'll reinforce your understanding of linear relationships and how changes in the slope or intercept alter the line's behavior on the graph.
Keep practicing with different values for m and b. Try to challenge yourself with negative slopes, zero slopes, or even undefined slopes, and see how these affect the line's graph. This practice not only enhances your mathematical skills but also your ability to visualize and interpret mathematical relationships in real-world applications.
What if the slope is zero?

+
If the slope is zero (m = 0), the line is horizontal and does not rise or fall. The equation becomes y = b, indicating that for any value of x, y is always equal to the y-intercept.
Can a line have an undefined slope?

+
Yes, a line has an undefined slope when it is vertical. In this case, the equation takes the form x = c, where c is a constant. There is no ‘rise’ or ‘run’; the line doesn’t move horizontally.
Why is it important to understand slope and y-intercept?
+
Understanding slope and y-intercept helps in interpreting and analyzing data or models in various fields like science, economics, and engineering, allowing you to predict outcomes or visualize relationships more accurately.


