Polynomial Long Division Worksheets: Boost Your Math Skills

Welcome to a detailed guide on mastering polynomial long division, a crucial algebraic skill that enhances not just your math abilities but also your problem-solving prowess. Polynomial long division is analogous to dividing two large numbers by hand, with the process being quite similar but involving variables instead of just digits.
Understanding Polynomial Long Division

The first step towards proficiency in this skill is understanding what polynomial long division entails. It involves dividing a polynomial by another polynomial to find the quotient and the remainder.
Why Polynomial Division is Important
- Preparation for Advanced Math: Knowledge of polynomial division prepares students for more advanced topics in algebra and calculus.
- Real-World Applications: From calculating areas and volumes in engineering to signal processing in telecommunications, polynomial division has practical uses.
- Conceptual Understanding: It helps in understanding concepts like factoring, solving equations, and simplifying complex expressions.
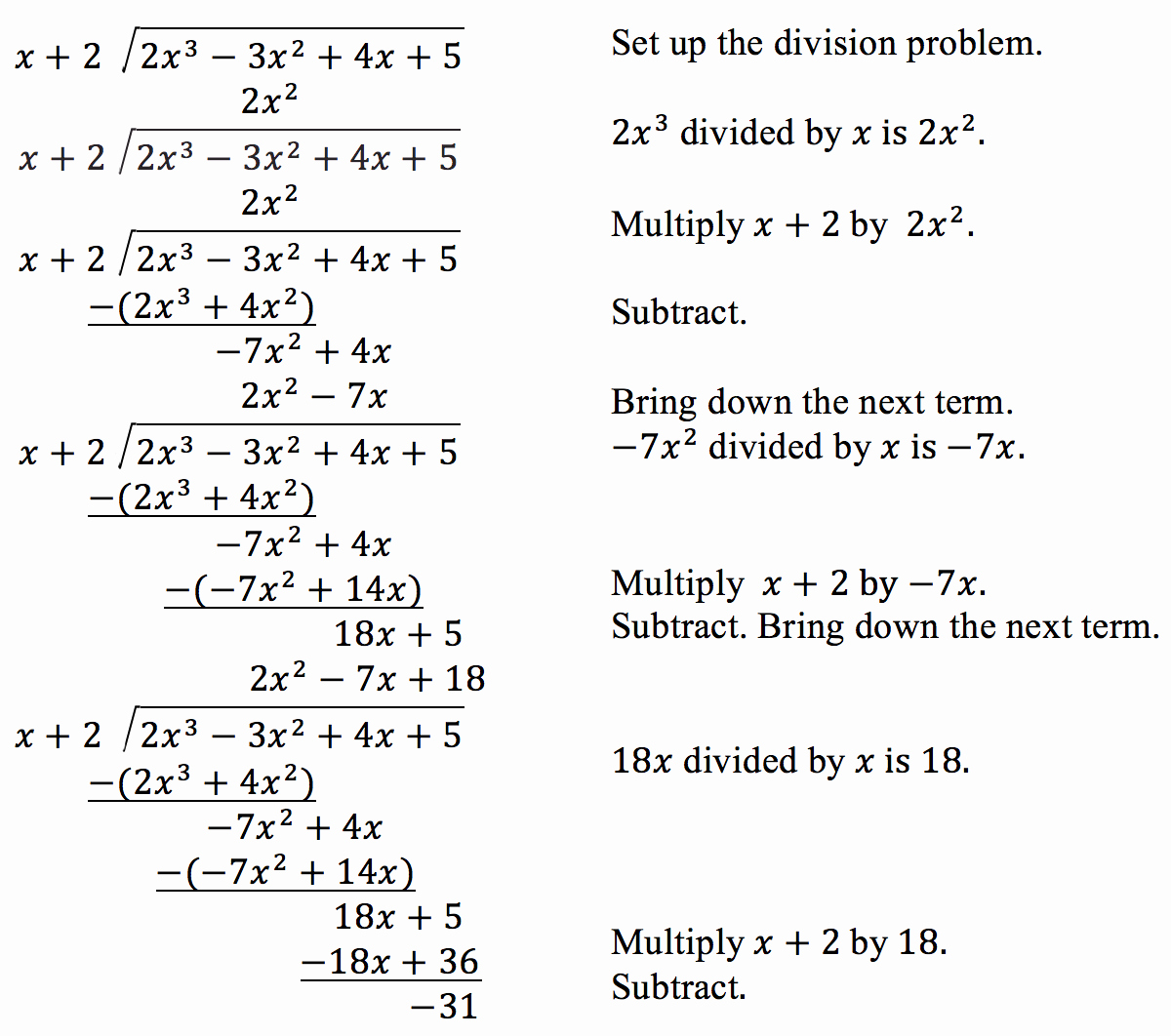
Key Steps in Polynomial Long Division

Here are the primary steps to follow when performing polynomial long division:
- Set Up: Write the polynomials in the standard form: descending powers of the variable.
- Divide: Divide the leading term of the dividend by the leading term of the divisor to find the first term of the quotient.
- Multiply: Multiply this term by the entire divisor and write the result below the dividend.
- Subtract: Subtract this result from the previous step from the dividend.
- Repeat: Continue the process until the degree of the remainder is less than the degree of the divisor.
Practicing with Worksheets

The best way to master polynomial long division is through regular practice. Here’s how you can utilize worksheets:
- Varied Exercises: Worksheets should include different levels of complexity, from simple linear division to cubic polynomials.
- Progressive Learning: Start with division by monomials and gradually increase difficulty by dividing by binomials and polynomials of higher degrees.
- Visual Aids: Use color coding or grid systems to make the process visually intuitive.
Example Worksheet Problem:

| Dividend | Divisor | Quotient | Remainder |
|---|---|---|---|
| x3 - 7x + 3 | x - 1 |

📝 Note: You would fill in the quotient and remainder after working through the problem.
Common Mistakes to Avoid

- Ignoring the Signs: Always consider the signs of each term when subtracting.
- Missing Steps: Ensure you follow all steps systematically, especially subtraction after each iteration.
- Carrying Over Incorrectly: When bringing down the next term, make sure to carry over correctly.
Beyond the Basics

Once you’re comfortable with the basics, here are some ways to expand your knowledge:
- Synthetic Division: A shorthand version of polynomial long division, useful when dividing by linear factors.
- Polynomial Factor Theorem: Learn how polynomial division relates to polynomial factorization.
- Error Analysis: Understand common errors in polynomial division through worksheets that require spotting and correcting mistakes.
As you continue to practice, remember that polynomial division isn't just about reaching an answer but also about understanding the underlying mathematical principles. The flexibility to tackle different polynomial expressions, the ability to visualize the process, and the confidence in solving real-world problems will be significantly enhanced through persistent practice.
Why is it important to set up polynomials correctly before division?

+
Proper setup ensures that the division process goes smoothly by aligning terms of like degrees, making it easier to divide and subtract systematically.
Can I use synthetic division instead of polynomial long division?

+
Yes, synthetic division is a more concise method when dividing by linear polynomials. However, for higher degree divisors or for understanding, polynomial long division is preferred.
What if the remainder is not zero in polynomial division?

+
Having a non-zero remainder means that the original polynomial cannot be fully factored by the divisor, but it gives the quotient and remainder as per the polynomial division algorithm.



