5 Easy Steps for Graphing Inequalities on a Number Line

Graphing inequalities on a number line might seem daunting at first, but it's a fundamental skill in mathematics that becomes straightforward once you understand the steps involved. Whether you're a student brushing up on algebra or someone interested in mathematical concepts for practical problem-solving, mastering this technique can be highly beneficial.
Understanding Inequalities

Before diving into the graphing process, it’s crucial to comprehend what inequalities are. In mathematics, an inequality compares two expressions and states that one is greater than, less than, or not equal to the other. Here are the basic symbols:
- < - Less than
- > - Greater than
- ≤ - Less than or equal to
- ≥ - Greater than or equal to
- ≠ - Not equal to
Step 1: Determine the Type of Inequality


Identify the type of inequality you are dealing with. Here’s how:
- If the inequality contains < or >, the endpoint of the graph will be an open circle.
- For ≤ or ≥, the endpoint will be a closed circle.
- If the inequality involves ≠, you’ll need to graph the number line in a unique way, showing that the number is not included.
Step 2: Identify the Interval

Once you know the type of inequality, locate the interval or intervals affected by this inequality. Here’s what you need to do:
- For single-variable inequalities like x < 5, you have one endpoint to deal with.
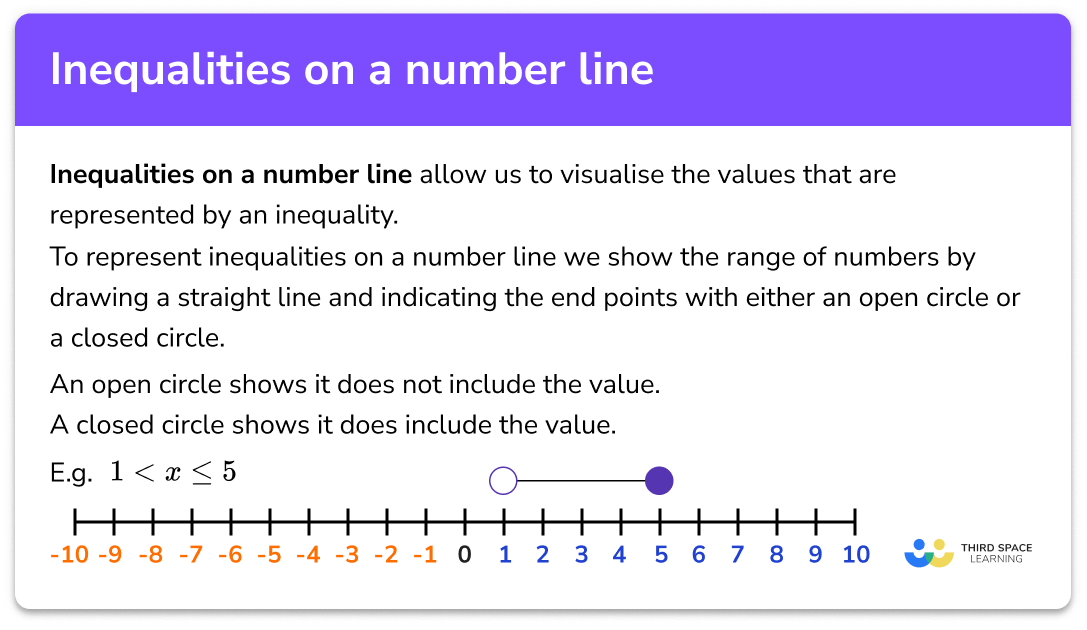
- For compound inequalities like -3 < x ≤ 5, you deal with a range between two endpoints.
Step 3: Choose Your Number Line


Graphing inequalities requires a number line. Here’s how to prepare it:
- Choose a number line that extends to cover all possible values within the inequality.
- Draw the line with tick marks to represent integer values or relevant increments.
⚠️ Note: For compound inequalities, you might need to use different scales on different parts of the number line depending on the range of values.
Step 4: Plot the Solution Set

Depending on the type of inequality, follow these steps:
- Less Than (or Greater Than): Place an open circle at the endpoint and draw a line to the left (or right) for less than (or greater than).
- Less Than or Equal To (or Greater Than or Equal To): Use a closed circle at the endpoint and shade towards the left (or right).
- Not Equal To: Mark the excluded number with a vertical line and shade away from it in both directions.
Step 5: Verify and Reflect


After plotting:
- Double-check that your graph reflects the inequality statement correctly. For example, if you graphed x ≥ 5, the arrow should be pointing towards positive infinity starting from a closed circle at 5.
- Reflect on the solution set. Consider the values that satisfy the inequality and how they relate to the real-world problem you might be solving.
In summary, graphing inequalities on a number line involves recognizing the type of inequality, identifying the relevant interval, choosing and setting up your number line, accurately plotting the solution set, and then verifying your work. This method not only aids in visual understanding but also provides a solid foundation for solving real-life problems where inequalities play a key role. Whether it's understanding financial thresholds or calculating optimal conditions in a scientific experiment, these steps can be universally applied.
What if I have a compound inequality?
+
Graph compound inequalities by dealing with each part separately. For -3 < x ≤ 5, you’ll have an open circle at -3, a closed circle at 5, and shade between them.
How do I know if I should use an open or closed circle?

+
An open circle represents that the endpoint is not included in the solution set (use for < or >), whereas a closed circle indicates that the endpoint is included (use for ≤ or ≥).
Can I graph inequalities in different dimensions?

+
Absolutely. In two dimensions, inequalities can be graphed using lines and shading to represent the solution set. This extends into three or more dimensions, but it gets increasingly complex.