Geometric Sequence and Series: Ultimate Worksheet Guide

Geometric sequences and series are fundamental concepts in mathematics that play a crucial role in understanding patterns and growth over time. Whether you're studying for a math exam, preparing a presentation, or simply exploring the beauty of numbers, mastering geometric sequences can be both a practical and an intellectually stimulating endeavor. This guide aims to offer an in-depth look into geometric sequences, providing a comprehensive worksheet to help you navigate through this mathematical territory with ease.
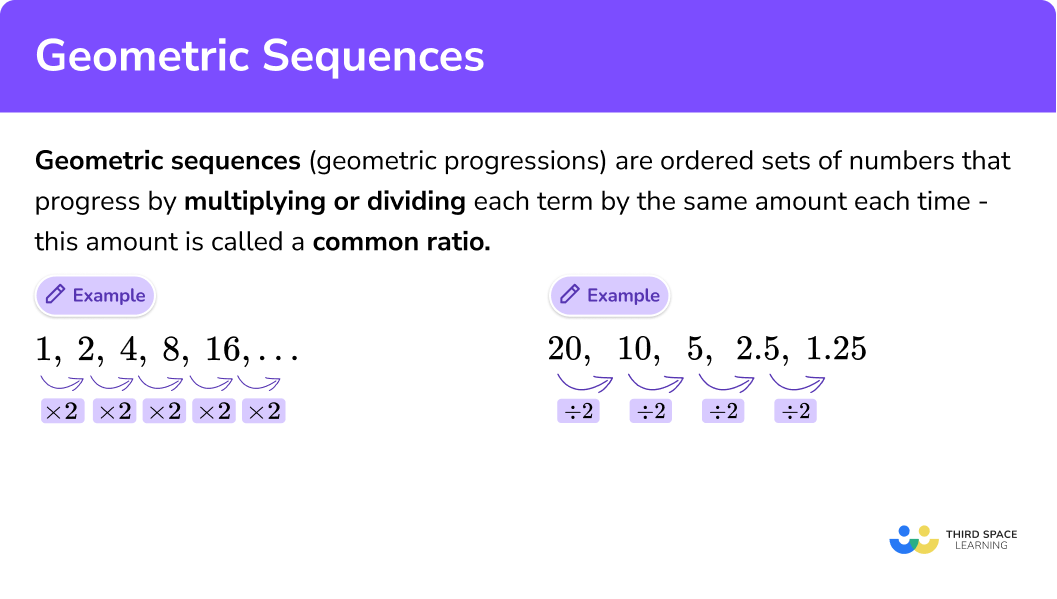
What is a Geometric Sequence?

A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio ®. Here’s how it’s defined:
- The first term is often denoted by ( a ).
- The ( n )-th term of a geometric sequence is given by ( a_n = a \cdot r^{n-1} ).
To illustrate, consider the sequence 2, 6, 18, 54…
💡 Note: In this sequence, ( a = 2 ) and ( r = 3 ).
Key Properties of Geometric Sequences

Understanding the properties of geometric sequences can help in solving related problems more efficiently:
- Monotonicity: If ( r > 1 ), the sequence is increasing; if ( 0 < r < 1 ), it’s decreasing.
- Boundedness: If ( r = 1 ), all terms are the same; if ( r ) is negative, the sequence oscillates.
- Convergence: The sequence converges if ( |r| < 1 ). The limit as ( n ) approaches infinity is 0.
Formulas for Geometric Series

A geometric series is the sum of the terms of a geometric sequence:
- The sum of the first ( n ) terms, denoted by ( S_n ), is given by: [ S_n = a \frac{r^n - 1}{r - 1} \quad \text{for } r \neq 1 ] [ S_n = a \times n \quad \text{for } r = 1 ]
- Infinite Series: If ( |r| < 1 ), the infinite series converges to: [ S = \frac{a}{1 - r} ]
Here’s an example of calculating the sum of a geometric series:
💡 Note: To find the sum of the first 5 terms of the sequence 2, 6, 18, 54… [ S_5 = 2 \frac{3^5 - 1}{3 - 1} = 2 \frac{243 - 1}{2} = 241 ]
Real-Life Applications

Geometric sequences are not just abstract mathematical constructs; they have real-world applications:
- Finance: Compound interest, population growth, or decay of radioactive materials.
- Biology: Growth rates of populations or bacterial cultures.
- Physics: Decay of particles, electrical resistance in circuits, etc.
Worksheet on Geometric Sequences and Series
| Problem | Solution |
|---|---|
| 1. Find the 7th term of the geometric sequence with ( a = 5 ) and ( r = 2 ). | Using ( a_n = a \cdot r^{n-1} ): [ a_7 = 5 \cdot 2^{6} = 5 \cdot 64 = 320 ] |
| 2. Sum the first 4 terms of the sequence starting with 3 and a common ratio of 1⁄3. | Applying ( S_n = a \frac{r^n - 1}{r - 1} ): [ S4 = 3 \frac{(\frac{1}{3})^4 - 1}{\frac{1}{3} - 1} = 3 \frac{\frac{1}{81} - 1}{- \frac{2}{3}} = \frac{3 \times \frac{80}{81}}{\frac{2}{3}} = \frac{240}{162} \approx 1.481 ] |
| 3. If ( S\infty = 10 ) and ( r = \frac{1}{2} ), find the first term ( a ). | From the infinite series sum formula ( S = \frac{a}{1 - r} ): [ 10 = \frac{a}{1 - \frac{1}{2}} = \frac{a}{\frac{1}{2}} \Rightarrow a = 10 \times \frac{1}{2} = 5 ] |

Engaging with geometric sequences through worksheets is an effective way to internalize this topic. Here are some additional tips for mastering geometric sequences:
- Practice: Regular practice with varying problems to understand different scenarios.
- Visualize: Use graphing tools to visualize the sequence's behavior, helping you grasp convergence or divergence visually.
- Conceptual Understanding: Go beyond mere calculation; understand why the formulas work and their implications in real life.
By working through examples and real-world problems, you'll develop a nuanced understanding of geometric sequences, enhancing your mathematical reasoning and problem-solving skills.
How do I find the common ratio of a geometric sequence?

+
To find the common ratio ( r ), divide any term by the previous one in the sequence. If ( a ) and ( an ) are terms, then ( r = \frac{a{n}}{a} ).
What’s the difference between arithmetic and geometric sequences?

+
An arithmetic sequence adds a common difference to get the next term, while a geometric sequence multiplies by a common ratio.
Can a geometric sequence have a negative common ratio?

+
Yes, a geometric sequence can have a negative common ratio, leading to terms that alternate in sign.
How do I know if an infinite geometric series will converge?

+
An infinite geometric series will converge if the absolute value of the common ratio ( |r| ) is less than 1.
What are some common real-life examples of geometric sequences?

+
Common examples include population growth, compound interest, the spread of diseases, and the dilution of solutions over time.



