Exponential Growth and Decay Problems: Master the Math

Exponential growth and decay problems often evoke a sense of complexity, yet understanding their mathematical foundations can be deeply rewarding. Whether you're a student tackling calculus, a biologist modeling population dynamics, or a financial analyst forecasting investment growth, mastering exponential functions can significantly enhance your problem-solving capabilities.
What is Exponential Growth?

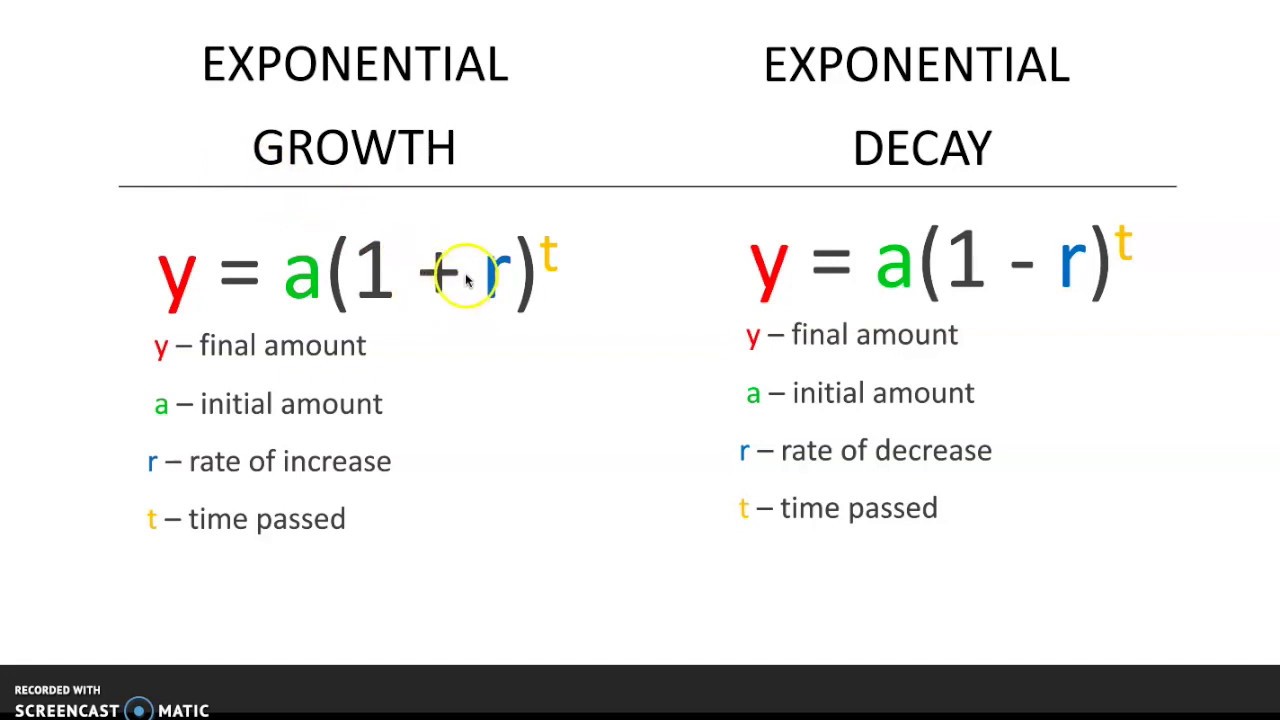
Exponential growth is the increase in a quantity at a rate that is proportional to its current value. The formula for exponential growth is:
- P(t) = P0 * ert
Where:
- P(t) - Amount at time t
- P0 - Initial amount
- r - Growth rate (expressed as a decimal)
- e - Euler’s number (approximately 2.718)
Understanding Exponential Decay

In contrast to growth, exponential decay describes the decrease in a quantity over time. This phenomenon is seen in radioactive decay, depreciating assets, or even cooling coffee. The formula for exponential decay is:
- P(t) = P0 * e-rt
Applications of Exponential Functions

Exponential functions have a wide range of applications:
- Finance: Interest rates, compound interest, stock valuations
- Physics: Decay of particles, electric charge in circuits
- Biology: Population growth, bacterial growth or decay, enzyme kinetics
- Engineering: Signal processing, heat dissipation
- Environmental Science: Radioactive decay of pollutants
Steps to Solve Exponential Growth Problems

Here’s a step-by-step guide to tackling exponential growth problems:
- Identify the initial conditions: Determine the starting value P0.
- Understand the growth rate: Convert the growth rate to a decimal if necessary.
- Formulate the equation: Use the formula P(t) = P0 * ert.
- Plug in the variables: Substitute known values for time and growth rate.
- Calculate: Solve the equation to find P(t) at the desired time.
Solving Exponential Decay Problems
The approach to solving decay problems mirrors growth problems:
- Identify the initial quantity: Determine the starting value P0.
- Convert the decay rate: Express the decay rate as a negative decimal or a half-life.
- Formulate the equation: Use P(t) = P0 * e-rt.
- Plug in the variables: Insert time and decay rate into the equation.
- Calculate: Determine P(t) at the specific time.
💡 Note: Remember that decay rates are often given in terms of half-life. To convert from half-life (T1⁄2) to rate constant (k), use the formula k = ln(2) / T1⁄2.
Examples of Exponential Growth and Decay

Here are two illustrative examples:
Example 1: Population Growth

Consider a bacterial culture with an initial population of 100 cells and a growth rate of 10% per hour. How many cells will there be after 5 hours?
- P0 = 100 cells
- r = 0.10 per hour
- t = 5 hours
The equation becomes:
- P(5) = 100 * e(0.10 * 5)
After calculating, we find:
- P(5) ≈ 164.87 cells
Example 2: Radioactive Decay

If the half-life of a radioactive isotope is 3 days and we start with 500 grams, how much will be left after 9 days?
- P0 = 500 grams
- T1⁄2 = 3 days
- k = ln(2) / 3 ≈ 0.231 days-1
- t = 9 days
The decay equation then becomes:
- P(9) = 500 * e-(0.231 * 9)
Calculating this, we find:
- P(9) ≈ 62.5 grams
Exponential growth and decay equations are powerful tools for predicting how quantities change over time. By grasping these principles, we equip ourselves with the ability to make informed predictions and analyses, from understanding biological systems to optimizing financial portfolios. Here's a quick reference table for the basic equations:
| Scenario | Formula |
|---|---|
| Exponential Growth | P(t) = P0 * ert |
| Exponential Decay | P(t) = P0 * e-rt |

The journey to understanding exponential models is not just about memorizing equations but about developing an intuition for how quantities evolve over time. The fascinating aspect of exponential functions is their ability to describe phenomena that are seemingly complex with surprising simplicity.
What is the difference between linear and exponential growth?

+
Linear growth increases at a constant rate, whereas exponential growth increases at a rate proportional to its current value, leading to much faster growth over time.
How do you know if an equation models growth or decay?

+
An equation with a positive exponent or growth rate r indicates growth, while a negative exponent or decay rate indicates decay.
Can exponential growth or decay continue indefinitely?

+
No, in reality, both growth and decay have natural limits. Growth often encounters limitations due to resources, and decay can reach a point where further reduction is negligible or impossible.