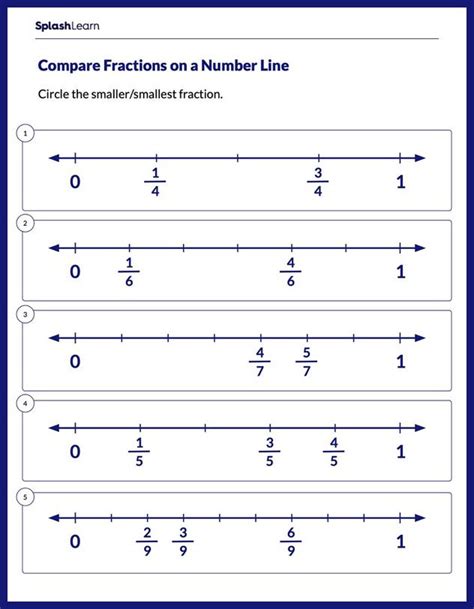
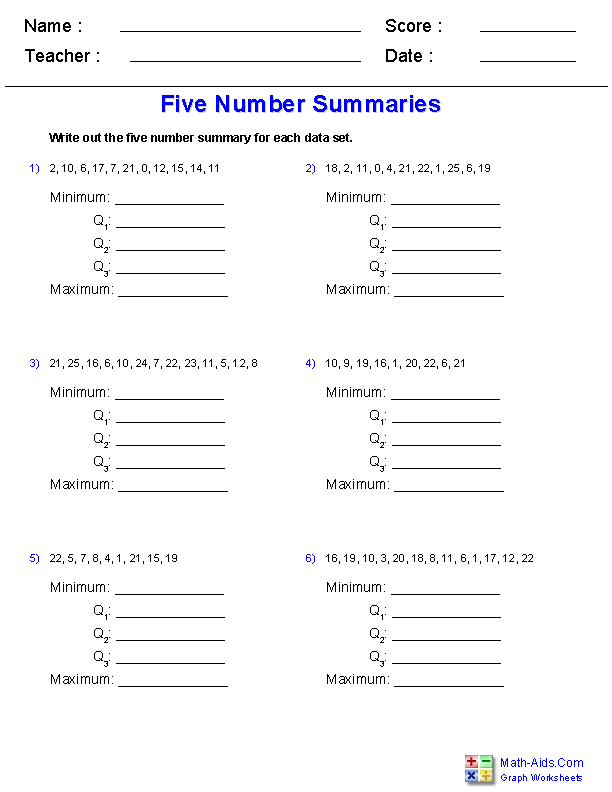
Five Number Summary Worksheet: Answer Key Revealed

Statistics, especially descriptive statistics, provide us with tools to summarize and understand datasets at a glance. One popular method for describing the distribution of data is the Five Number Summary. This method encapsulates the minimum, first quartile (Q1), median (second quartile or Q2), third quartile (Q3), and the maximum of a dataset. Here's a deeper dive into creating a Five Number Summary worksheet and revealing its answer key:
Understanding the Five Number Summary
The Five Number Summary serves as a robust way to:
- Summarize the central tendency, spread, and skewness of the data.
- Identify outliers if any.
- Provide insights into the distribution of data without complex calculations.
How to Create a Five Number Summary

Step 1: Organize the Data
First, you need to ensure your data is sorted in ascending order. This step is crucial because finding the median, quartiles, and other values is much easier when data is arranged systematically.
Step 2: Finding the Minimum and Maximum

Identify the lowest and highest values in your dataset. These are straightforward to find and are critical to understanding the full range of your data.
Step 3: Determining the Median

The median (Q2) is the middle value of your sorted dataset. If your dataset has an even number of observations, the median is the average of the two middle numbers.
Step 4: Calculating the First and Third Quartiles

The quartiles divide your dataset into four parts, each containing 25% of the data:
- Q1 (First Quartile): The median of the lower half of the dataset.
- Q3 (Third Quartile): The median of the upper half of the dataset.
📝 Note: Use methods like the "Tukey" or "M & M" to calculate quartiles accurately. Ensure you understand your method's nuances, especially for datasets with an odd number of observations.
Example with Answer Key

Consider this dataset of exam scores:
| Scores | 45, 55, 60, 65, 70, 75, 80, 85, 90, 95, 100 |
|---|

Solution:

- Minimum: 45
- Q1: (60 + 65) / 2 = 62.5
- Median (Q2): 75
- Q3: (85 + 90) / 2 = 87.5
- Maximum: 100
Interpreting the Five Number Summary

Now that we've calculated our summary, here's what it tells us:
- The range of scores in this class varies widely from 45 to 100.
- The middle 50% of the data (from Q1 to Q3) is between 62.5 and 87.5, indicating a relatively broad spread around the median.
- The median being 75 shows that half the students scored 75 or above, which could indicate a positive skew.
📝 Note: The Five Number Summary can be extended to create a box-and-whisker plot for a visual representation of data distribution.
Additional Considerations
While the Five Number Summary is a powerful tool, there are some additional points to consider:
- Outlier Detection: You can identify potential outliers using the Interquartile Range (IQR) method, where anything below Q1 - 1.5 * IQR or above Q3 + 1.5 * IQR might be considered an outlier.
- Data Size: Smaller datasets might not yield a precise Five Number Summary. For datasets with fewer than 10 observations, consider using more robust methods.
- Dealing with Skewness: Skewed data can mislead the interpretation of quartiles. In such cases, a median absolute deviation might provide more insight.
In the pursuit of understanding data distributions, the Five Number Summary worksheet serves as an invaluable tool, offering a clear, concise snapshot of the dataset's characteristics. This method, alongside other statistical tools, provides educators, students, and data analysts with the means to make informed decisions based on the data at hand. Whether you're analyzing exam scores, financial data, or any other kind of numerical data, the Five Number Summary offers a robust approach to understanding the data's behavior, identifying potential outliers, and drawing valuable insights. Remember that while this summary is straightforward, it's the interpretation and application of these statistics that truly enhance our understanding of the dataset's story.
What is the importance of calculating Q1 and Q3 in a Five Number Summary?

+
The first (Q1) and third (Q3) quartiles divide the data into four equal parts, providing insights into the spread of the central half of your dataset. This helps in understanding the dispersion of data and detecting any potential skewness or outliers.
How do I handle an odd number of observations in a dataset?

+
For an odd number of observations, the median (Q2) will be the middle number. For Q1 and Q3, exclude the median and then find the medians of the remaining lower and upper halves.
Can the Five Number Summary detect outliers?

+
Yes, by using the Interquartile Range (IQR), where any data points below Q1 - 1.5 * IQR or above Q3 + 1.5 * IQR could be considered as outliers. This method is particularly useful for identifying extreme values that might skew the data distribution.