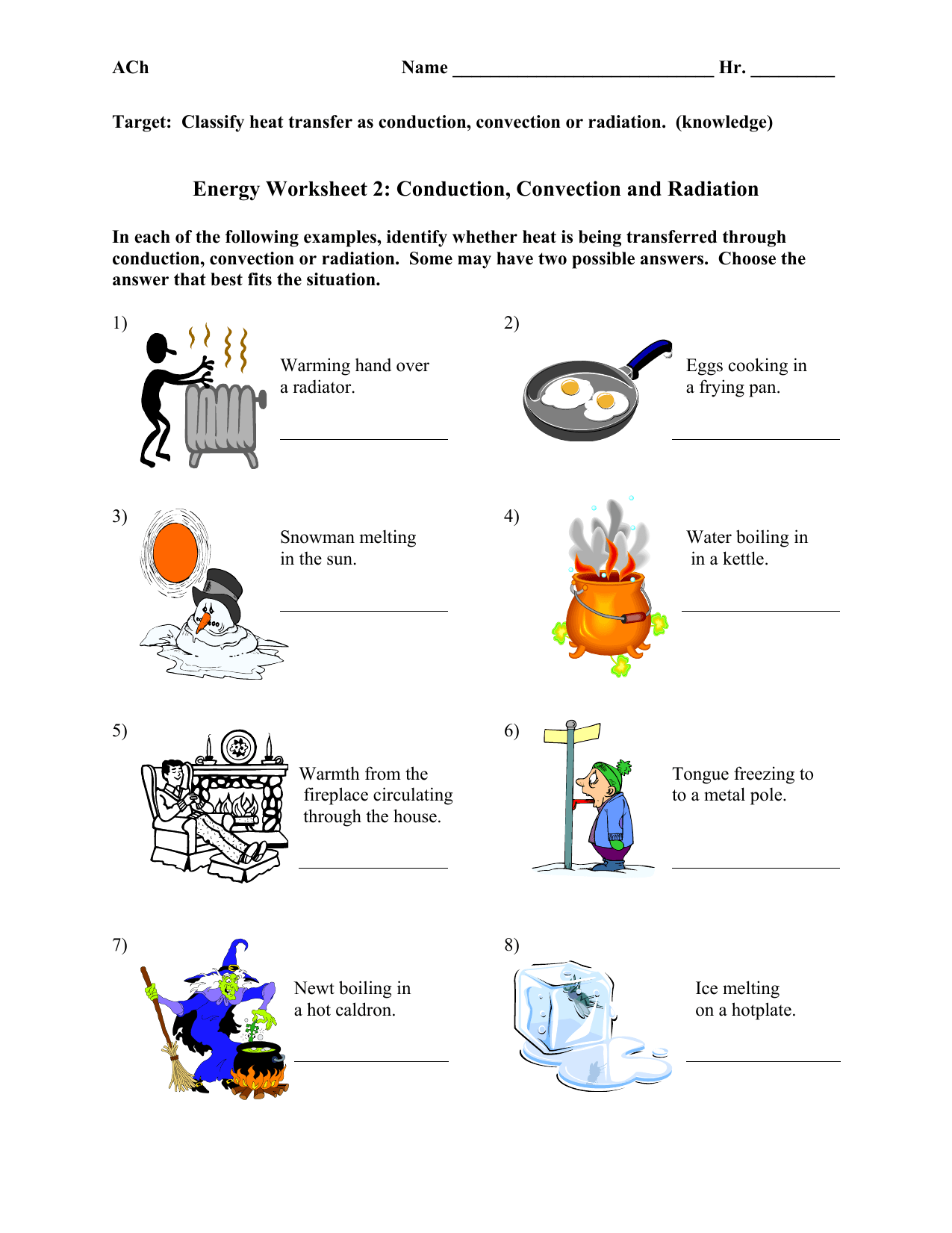
Finding Slope From Table: Easy Worksheet Guide

Understanding Slope: Basics for Students
When it comes to learning about slope in mathematics, especially in algebra or linear functions, one of the foundational skills is determining the slope of a line from given data points or a table. This skill is crucial because slope is a measure of how steep a line is, and understanding it helps in various applications from engineering to economics. In this guide, we'll explore how to find slope from a table of values, which can be a straightforward approach to learning this concept.
What is Slope?

Before diving into the method of finding slope from a table, let's briefly understand what slope is:
- Definition: Slope, often represented as 'm', is the ratio of the vertical change (rise) to the horizontal change (run) between any two points on a line.
- Formula: The slope formula is m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are points on the line.
How to Find Slope From a Table

Let's walk through the steps needed to find the slope from a table:
Step 1: Identify Points on the Table

Find at least two points from the table. A typical table in math might look like this:
| X | Y |
|---|---|
| 0 | 3 |
| 1 | 5 |
| 2 | 7 |

From this table, we can select any two points, but let's choose the first and the last for clarity:
- First Point: (0, 3)
- Last Point: (2, 7)
📘 Note: Choosing points further apart usually gives a clearer calculation of slope, but any two will work.
Step 2: Apply the Slope Formula

Use the slope formula with the points you've identified:
- Let (x1, y1) be (0, 3) and (x2, y2) be (2, 7).
- Insert these into the formula: m = (y2 - y1) / (x2 - x1) = (7 - 3) / (2 - 0) = 4 / 2 = 2.
The slope of the line represented by the data in the table is 2, indicating that for every unit increase in x, y increases by 2 units.
Step 3: Check Consistency
Ensure that the slope is consistent throughout the table if applicable. For our example:
- If we choose points (1, 5) and (2, 7), the slope calculation should give the same result.
- m = (7 - 5) / (2 - 1) = 2 / 1 = 2, confirming our previous calculation.
Common Mistakes to Avoid

- Using Subtraction Wrongly: Always subtract y2 - y1 and x2 - x1 in that order. Mismatching the order can lead to incorrect results.
- Not Simplifying Fractions: If the result of your slope calculation is a fraction, simplify it to get the true slope.
- Ignoring Zero Points: Points where either x or y is zero are valuable. They often simplify calculations, especially when using zero as x1.
💡 Note: When dealing with large numbers or fractions, check your work to ensure you've not made arithmetic errors.
Why This Matters?

Understanding how to calculate slope from a table is vital for several reasons:
- Real-World Applications: Slope is used in fields like physics to determine velocity, in architecture to design ramps or stairs, and in economics to analyze cost or revenue changes.
- Predictive Modeling: With slope, one can predict future values by extending the line’s trend.
- Graphing: Slope directly influences the shape of graphs, helping visualize data relationships.
In this guide, we’ve covered how to find the slope of a line from a table, why it’s important, and common pitfalls to avoid. Mastery of this technique not only aids in understanding linear equations but also enriches the ability to analyze data in real-world scenarios.
What if my table has more than two points?

+
You can use any two points from the table to calculate slope. However, ensure that the slope remains consistent for all pairs of points to confirm linearity. If slopes vary, it might suggest a non-linear relationship or errors in data.
Can the slope ever be negative?

+
Yes, if y decreases as x increases, the slope will be negative. This means the line slopes downward from left to right on a graph.
How do I interpret a slope of zero?

+
A slope of zero means the line is horizontal. It indicates no change in y for any change in x, which means the function is constant.