5 Proven Methods to Solve Slope Worksheet Quickly

Understanding Slope Worksheets

When you’re faced with slope worksheets, your main task is to determine the slope of a line, which is essentially how steep it is, from given points, equations, or graphs. Here’s a fundamental understanding:
- Slope can be derived from the formula slope = (y2 - y1)/(x2 - x1).
- Worksheets often present this in various forms requiring different calculations or visualizations.
Mastering slope calculation can greatly enhance your understanding of algebra, geometry, and even calculus. Here are five proven methods to solve these problems efficiently:
1. Use the Slope Formula Directly

The most straightforward method is to apply the slope formula directly:
- Identify two points on the line or graph.
- Plug those points into the formula: (y2 - y1)/(x2 - x1).
- Simplify to find the slope.

This method is particularly effective for linear equations where you have clear coordinates.
📝 Note: Always double-check your calculations, especially if the slope results in a fraction or negative number.
2. Recognize Common Slopes

Some slopes are so common that recognizing them can speed up your work:
- Horizontal lines have a slope of zero.
- Vertical lines are undefined in slope.
- Diagonal lines often have slopes like 1, -1, or simple fractions like 1⁄2 or 2⁄1.
Here’s a quick reference table:
| Type of Line | Slope |
|---|---|
| Horizontal | 0 |
| Vertical | Undefined |
| Diagonal 45° | 1 or -1 |

🌟 Note: Knowing these common slopes can save time on multiple-choice questions where these are often options.
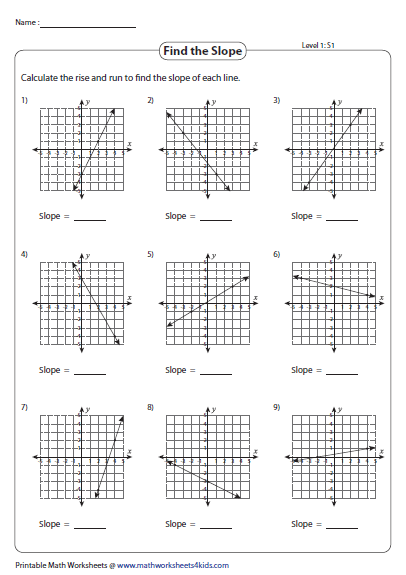
3. Graph and Estimate

If you are provided with a graph:
- Plot the points given or the line provided on the graph.
- Estimate the slope by looking at the line's steepness.

This method is useful when you’re dealing with less precise or more visual math problems.
👀 Note: Estimation is not exact, so be cautious when relying solely on this method for precise calculations.
4. Convert to Slope-Intercept Form

If you’re given a linear equation, convert it to slope-intercept form:
- The slope-intercept form is y = mx + b, where m is the slope.
- Rearrange the equation to isolate y, and the coefficient of x is your slope.
For example:
- 3x - 6y = 12 becomes -6y = -3x + 12, then y = 0.5x - 2. Here, the slope m is 0.5.
🔧 Note: This method is particularly useful when dealing with equations involving fractions or negatives.
5. Use Parallel and Perpendicular Relationships

Sometimes, understanding how slopes relate:
- Parallel lines have the same slope.
- Perpendicular lines have slopes that are negative reciprocals of each other.
For instance, if one line has a slope of 3, any line parallel to it will also have a slope of 3, and a line perpendicular to it will have a slope of -1⁄3.

🔗 Note: Remember, this doesn't apply to vertical and horizontal lines as their slopes are 0 and undefined respectively.
The last step in solving slope worksheets efficiently is wrapping up your understanding:
- Utilizing the slope formula directly is a fundamental approach for straightforward calculations.
- Recognizing common slopes can speed up your work in multiple-choice scenarios.
- Estimating from graphs helps when dealing with visual or imprecise math problems.
- Converting equations to slope-intercept form provides a quick slope identification.
- Using properties of parallel and perpendicular lines can solve problems without needing actual coordinates or equations.
These methods not only make slope calculations more manageable but also deepen your understanding of the underlying principles. Whether you’re a student tackling homework or preparing for an exam, these strategies will ensure you approach slope problems with confidence and efficiency.
Can slopes be negative?

+
Yes, slopes can definitely be negative. A negative slope indicates that the line decreases as it moves from left to right.
What does an undefined slope mean?

+
An undefined slope means the line is vertical, and there’s no horizontal change to calculate the slope from.
Is there a way to calculate slope without plotting points?
+
Yes, if you have the equation in slope-intercept form (y = mx + b), you can directly find the slope m without plotting points.