5 Steps to Master Dilated Coordinates Worksheets

Engaging with dilated coordinates worksheets is an excellent way to deepen your understanding of geometric transformations, particularly dilation. This process involves resizing a figure proportionally, either by enlarging or reducing it, while keeping its shape intact. In this comprehensive guide, we'll explore the steps necessary to master dilated coordinates worksheets, making sure you're equipped with the knowledge and skills for both academic success and practical application.
Understanding Dilation in Coordinates
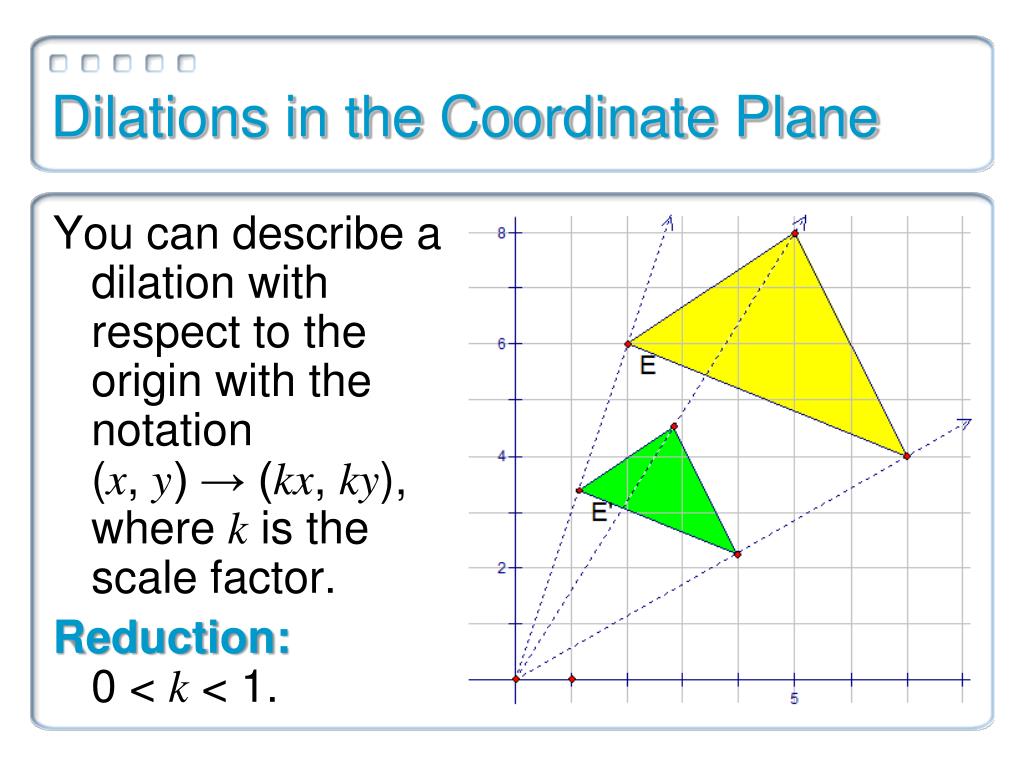
Before diving into the specifics of the worksheets, let's clarify what dilation means in the context of coordinate geometry. Dilation involves:
- Expanding or shrinking a figure from a fixed center point, known as the center of dilation.
- Maintaining the shape of the figure through scaling by a given factor, called the dilation factor or scale factor.
💡 Note: Remember that the center of dilation does not change its position during the transformation.
Step 1: Identify the Center and Scale Factor

To begin working with dilated coordinates worksheets, you need to:
- Find the center of dilation, which is typically given or can be deduced from the diagram.
- Identify the scale factor. This can be either greater than 1 (enlarging) or between 0 and 1 (reducing).
Consider the following example:
| Point | Original Coordinates | Scale Factor | New Coordinates |
|---|---|---|---|
| A | (2, 3) | 2 | (4, 6) |
| B | (1, 1) | 2 | (2, 2) |
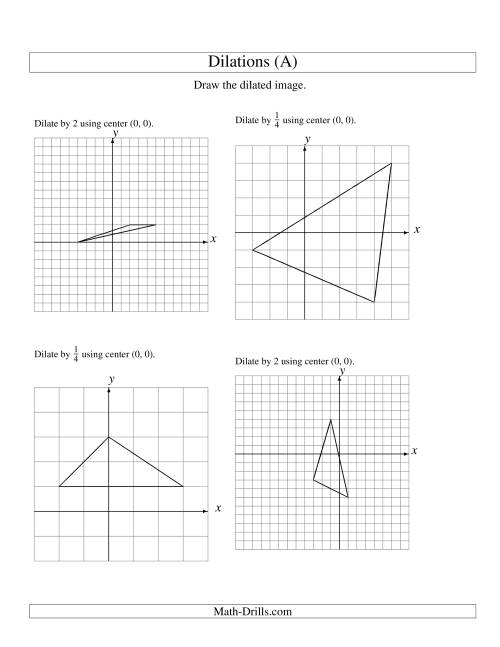
Here, the center of dilation is at the origin (0, 0), and the scale factor is 2, meaning each coordinate is multiplied by 2 to find the new coordinates.
Step 2: Apply the Dilation Formula

Once you have the center and scale factor, apply the dilation formula:
[ (x, y) \rightarrow (kx + c_x - kc_x, ky + c_y - kc_y) ]
Where:
- (x, y) are the original coordinates
- k is the scale factor
- (c_x, c_y) are the coordinates of the center of dilation
In the case of dilation from the origin (0, 0), the formula simplifies to:
[ (x, y) \rightarrow (kx, ky) ]
🧐 Note: If the center of dilation is not the origin, adjust the formula accordingly.
Step 3: Plot the Dilated Figure

With the new coordinates calculated, plot them on the worksheet or coordinate plane. Ensure that:
- All points are accurately reflected according to the new coordinates.
- The shape remains proportional, just at a different size.
Remember that while plotting:
- Maintain the shape of the figure to preserve its geometric properties.
- Check for any scaling errors by visually comparing the original to the dilated figure.
Step 4: Analyze the Transformation

Analysis is key to mastering dilated coordinates:
- Observe how distances change; distances from the center of dilation to each vertex are scaled by the dilation factor.
- Understand how angles and parallel lines remain unchanged due to similarity in figures.
- Consider the area change, which is proportional to the square of the scale factor.
Step 5: Practice with Real Examples

To solidify your mastery over dilated coordinates worksheets, engage in:
- Practice problems from textbooks or online resources.
- Creating your own dilation problems and solving them.
- Applying dilation to real-life scenarios like map scaling or photography.
Using dilation in practical applications can enhance both your understanding and application of the concept:
- Architects use dilation to scale down their blueprints for presentation.
- Photographers apply dilation to zoom in or out of scenes without distorting the subject.
💼 Note: Dilation is a powerful tool in various fields, not just mathematics.
To summarize, mastering dilated coordinates worksheets requires understanding dilation, identifying the necessary components, applying the correct formula, plotting the transformed figure, analyzing the transformation, and practicing with real examples. By following these steps, you'll not only excel in your worksheets but also gain a valuable skillset in geometric transformations.
Why do we need to know dilation in geometry?
+
Dilation is essential in understanding how shapes and figures change size proportionally while retaining their shape. It has practical applications in fields like engineering, architecture, and art.
How does the center of dilation affect the transformation?

+
The center of dilation is the fixed point from which all measurements are scaled. If the center is not the origin, the transformation formula adjusts to account for this shift in position.
Can the scale factor be a fraction or decimal?

+
Yes, the scale factor can be any positive number, including fractions or decimals, to either enlarge or reduce the figure.