5 Ways to Master Factoring With GCF Worksheets

In mathematics, one of the essential skills every student should master is factoring. Among various factoring techniques, using the Greatest Common Factor (GCF) stands out as a fundamental approach that not only simplifies expressions but also paves the way for understanding more complex algebraic concepts. This blog will delve into five effective ways to master factoring using GCF worksheets, providing tools, insights, and step-by-step guidance for educators and students alike.
Understanding the Basics of GCF


Before diving into specific strategies, it's crucial to understand what the Greatest Common Factor (GCF) is:
- The GCF of two or more numbers is the largest factor that divides them both without leaving a remainder.
- For example, the GCF of 12 and 18 is 6 since 6 divides both numbers and is the largest factor that does so.
Strategy 1: Utilize Interactive GCF Worksheets
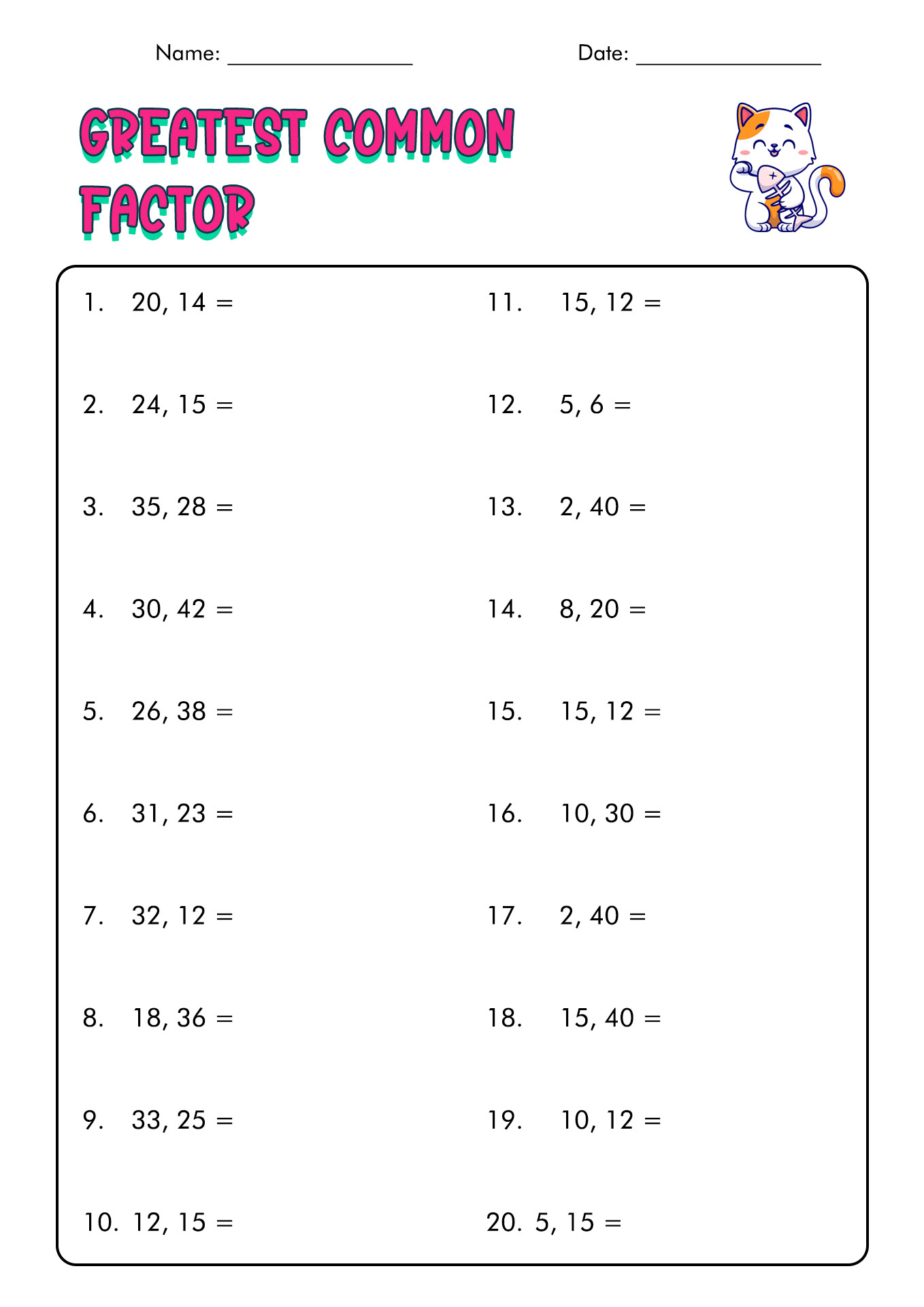
Interactive worksheets provide a dynamic way for students to engage with GCF. Here's how you can maximize their benefits:
- Online Platforms: Use platforms that offer digital, interactive GCF worksheets. These often include games or interactive puzzles which can make learning fun.
- Practice with Variety: Choose worksheets that introduce GCF in different contexts, such as algebraic expressions, word problems, or visual problems using geometric shapes.
Strategy 2: Gradual Complexity Increase


Factoring should be taught in a structured manner, where complexity increases over time:
- Start with basic number problems to ensure understanding of the GCF concept.
- Move to factoring numbers with a larger common factor.
- Introduce algebraic expressions where the GCF involves variables.
This approach helps students build confidence and competence progressively.
Strategy 3: Real-Life Applications of GCF

Connecting mathematical concepts to real-world applications can enhance understanding:
- Geometry: Calculating areas of rectangular fields where GCF helps in finding common dimensions.
- Home Economics: Dividing resources equally among family members using GCF to determine servings or portions.
💡 Note: Always ensure the real-life examples are relatable to the student's age group and interests for maximum engagement.
Strategy 4: Peer Learning and Group Activities
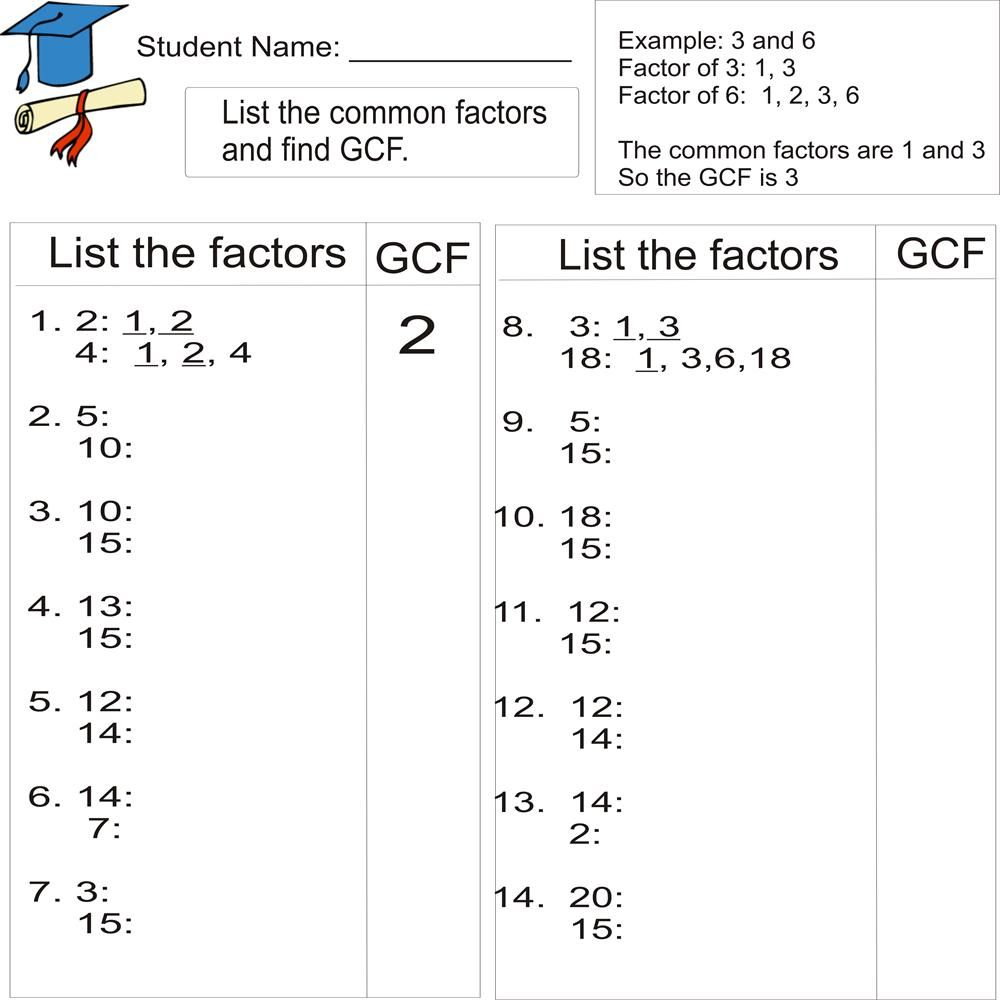

Peer learning fosters:
- Cooperative problem-solving.
- Explanation of concepts through different perspectives.
- Promoting teamwork and communication skills.
Group activities like GCF relay races, where teams solve factoring problems to "win" pieces of a puzzle, can turn learning into an exciting game.
Strategy 5: Error Analysis and Feedback
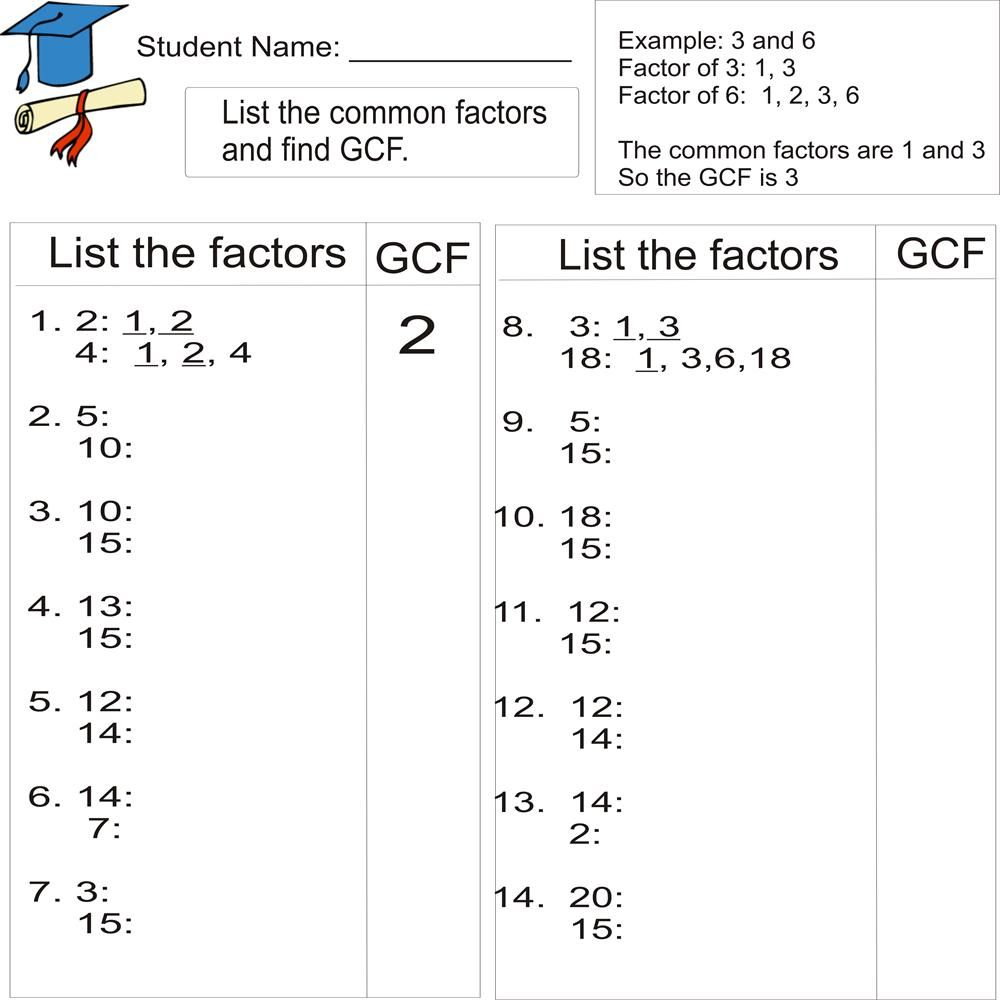
One of the most overlooked yet effective strategies is:
- Error Identification: Present students with incorrect solutions to factoring problems and ask them to identify and correct the errors.
- Feedback Sessions: Regular sessions where students discuss their solutions with peers or the teacher can clarify misconceptions and strengthen understanding.
📌 Note: Ensure that feedback is constructive, focusing on both what was done correctly and areas for improvement.
To conclude, mastering GCF through worksheets involves a blend of practical exercises, understanding the core concept, and applying it in various scenarios. By progressively introducing complexity, using interactive tools, relating math to real life, encouraging teamwork, and focusing on error correction, students can gain a deep understanding of factoring. This multifaceted approach ensures that students not only solve mathematical problems efficiently but also appreciate the broader applications of GCF in their daily lives.
Why is GCF important in factoring?

+
The GCF is crucial in factoring because it allows us to simplify expressions by extracting common factors, making subsequent steps in problem-solving easier.
How can I find GCF worksheets?

+
Many educational websites offer free GCF worksheets. Also, check out your school’s math resources or ask your math teacher for worksheets or references.
What if students find GCF challenging?

+
Start with the basics, use visual aids, relate problems to real-life scenarios, and encourage practice with patience. Group work and error analysis can also provide peer support and insight.
Can GCF be used in subjects other than math?

+
Yes, concepts like GCF can be applied in subjects like physics (when simplifying formulas) or economics (when dealing with quantities or divisions of resources).



