5 Easy Steps for Factoring Quadratic Equations

Factoring quadratic equations is a fundamental skill in algebra, used frequently in both high school math classes and in higher-level mathematics. Understanding how to factor these equations not only simplifies solving them but also gives insight into the underlying polynomial structure. Here, we'll walk through five straightforward steps to factor quadratic equations effectively.
1. Understanding the Quadratic Equation

A quadratic equation has the standard form ax² + bx + c = 0. Here:
- a is the coefficient of x²,
- b is the coefficient of x,
- c is the constant term.

💡 Note: Quadratic equations can have different forms, but the standard form is used for factoring purposes.
2. Identifying if Factoring is Possible

Not all quadratic equations can be easily factored. Here are conditions to check:
- If a is not 1, factoring can be more complex but still possible.
- If the discriminant (b² - 4ac) is a perfect square, factoring is generally possible.
3. Applying the AC Method

The AC method is useful for factorizing when a ≠ 1:
- Calculate ac.
- Find two numbers that multiply to give ac and add to give b.
- Rewrite the middle term bx using these two numbers.
- Factor by grouping.
| Step | Example |
|---|---|
| Calculate ac | For 2x² + 5x + 3, ac = 2*3 = 6 |
| Find two numbers | 6 = 6*1 or 3*2; 6+1 = 7, 3+2 = 5 |
| Rewrite | 2x² + 5x + 3 = 2x² + 3x + 2x + 3 |
| Factor by grouping | = x(2x + 3) + 1(2x + 3) = (x+1)(2x+3) |

📝 Note: The AC method is particularly useful when the leading coefficient a is not 1.
4. Using the Difference of Squares

If your quadratic equation has a squared term and the constant term is negative, you might use the difference of squares:
- If a = 1, and c is negative, you can use x² - c = (x - √c)(x + √c).
5. Factor Completely

After factoring by any of the methods above, always check if you can factor further. Some polynomials might have factors that can be reduced or simplified further.
🔍 Note: Ensure all factors are simplified completely for a fully factored form.
By following these steps, you will gain a firm grasp on factoring quadratic equations, making it easier to solve and understand their properties. Factoring isn’t just about finding solutions; it’s about mastering a critical aspect of polynomial manipulation.
Understanding how to factor quadratics not only aids in solving equations but also in manipulating and simplifying algebraic expressions. This skill is invaluable in fields such as engineering, physics, computer science, and finance where quadratic equations often appear in real-world applications.
What if a quadratic equation cannot be factored?

+
If a quadratic equation does not factor nicely into integers, you might use the quadratic formula (x = (-b ± √(b² - 4ac)) / 2a) to solve it. However, this does not always yield integers or even rational solutions.
Can I use factoring to solve all quadratic equations?

+
No, not all quadratic equations can be factored using integers or rational numbers. However, factoring is an essential step in simplifying or transforming the equation before possibly resorting to other methods like completing the square or using the quadratic formula.
How does understanding factoring help in real-world problems?
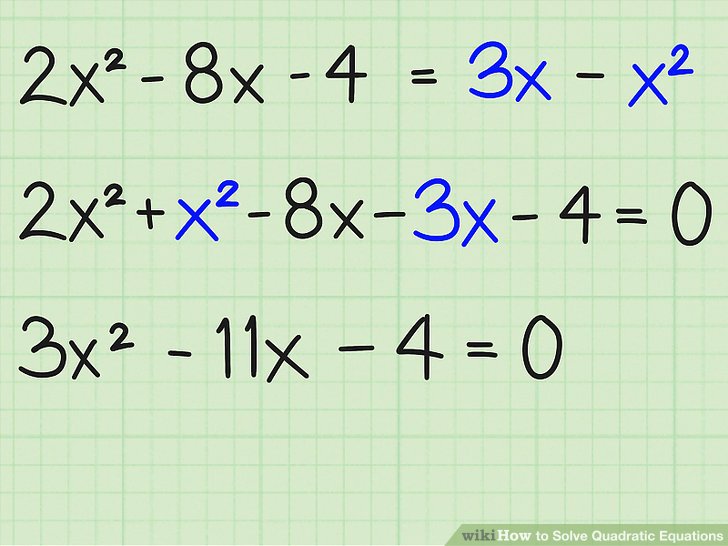
+
Factoring helps in breaking down complex problems into more manageable parts. For instance, in physics, you might use it to understand projectile motion or in finance to calculate break-even points for investments.