7 Ways to Master Logarithm Expansion Quickly

If you've ever struggled with mathematics, particularly with the concept of logarithms, you're not alone. Logarithms can seem daunting at first, but with the right strategies, you can master them quickly. This guide will delve into logarithm expansion methods that can simplify your learning journey and make logarithm problems feel more manageable.
Understanding Logarithms

Before diving into expansion techniques, let’s solidify your understanding of logarithms:
- The logarithm is the power to which a base must be raised to produce a given number. For instance,
logₐ(x) = bmeansaᵇ = x. - The base of a logarithm is crucial; common ones include base 10 (common logarithms) and base e (natural logarithms).

Expand Logarithms Using Properties

The power of logarithms lies in their properties which make expansion straightforward:
- Product Property:
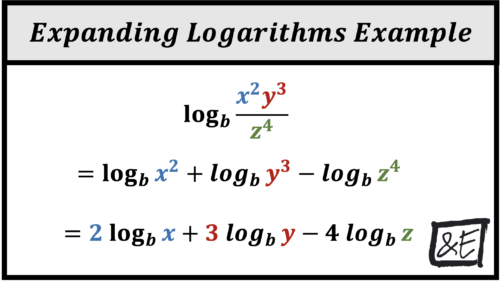
logₐ(xy) = logₐ(x) + logₐ(y) - Quotient Property:
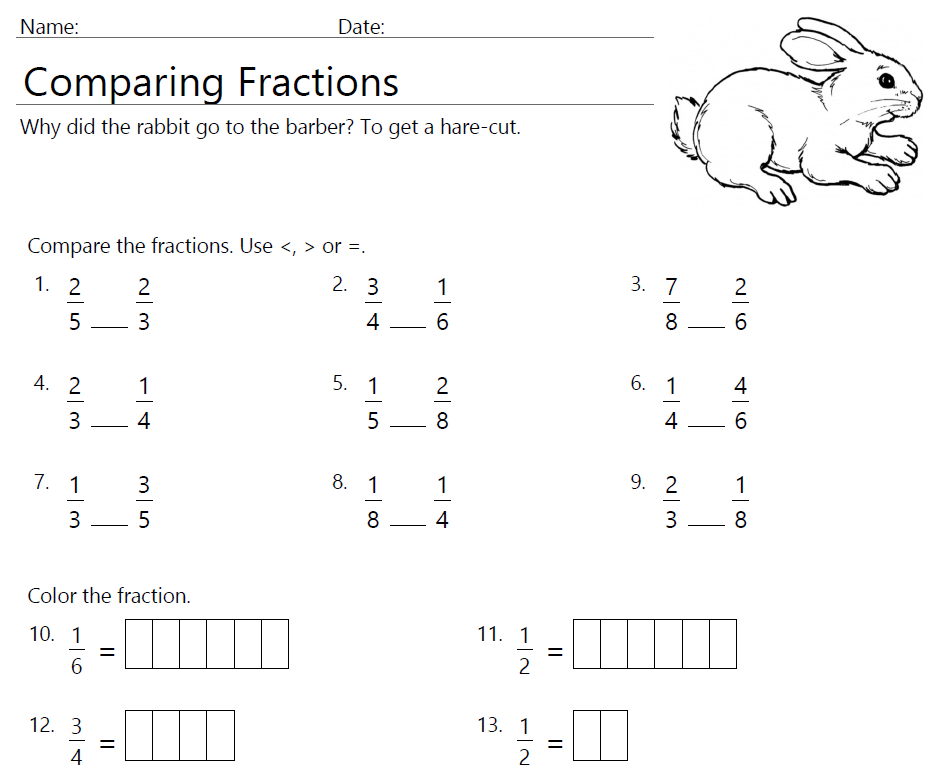
logₐ(x/y) = logₐ(x) - logₐ(y) - Power Property:
logₐ(xʸ) = y * logₐ(x)
Examples:
Here are some examples to illustrate these properties:
- Product Property:
log(2 * 4) = log(2) + log(4) = 1 + 0.602 = 1.602 - Quotient Property:
log(16⁄4) = log(16) - log(4) = 1.204 - 0.602 = 0.602 - Power Property:
log(25^2) = 2 * log(25) = 2 * 1.398 = 2.796
Key Steps in Expanding Logarithms

To expand logarithms effectively, follow these steps:
- Identify whether the logarithm is a product, quotient, or power.
- Apply the appropriate property to expand.
- Keep track of signs when dealing with the quotient property.
- Remember that the base must be the same for expansion to work.

✍️ Note: When expanding, always check if the bases match; otherwise, proceed with caution or use change of base formula.
Logarithm Expansion in Real-world Problems

Logarithms aren’t just theoretical; they’re used in fields like:
- Science (pH calculations)
- Engineering (signal processing)
- Economics (growth rates)
Advanced Logarithm Expansion Techniques

Mastering logarithms can also involve more complex situations:
Combining Properties

Expand log(a * b / (c * d)) using:
- First apply the quotient property:
log(a * b) - log(c * d) - Then apply the product property to each log:
(log(a) + log(b)) - (log© + log(d))
Why Expand Logarithms?

Logarithm expansion simplifies:
- The manipulation of complex expressions
- Calculation and comparison of logarithms
- Graphing logarithmic functions
To wrap up our discussion on logarithm expansion, we've explored the fundamental properties that allow for quick manipulation of logarithms. Understanding these properties and how to apply them efficiently can streamline your problem-solving in mathematics and beyond. Practice expanding different logarithmic expressions to boost your familiarity, and remember the real-world applications where logarithm skills are invaluable.
What is the purpose of expanding logarithms?

+
Expanding logarithms simplifies complex expressions, making it easier to solve equations, compare values, or understand logarithmic functions graphically.
Can logarithms with different bases be expanded together?

+
No, the bases must be the same for direct expansion. Use the change of base formula if needed to convert logarithms to a common base.
How do I handle logarithms with exponents?

+
Use the power property of logarithms to bring the exponent down in front of the logarithm, allowing for further expansion if necessary.