Master Distributive Property with Our Equation Worksheet

Whether you're a student grappling with algebra or a teacher looking to scaffold the learning process, understanding the distributive property is fundamental. This key algebraic principle not only simplifies equations but also serves as a stepping stone to more complex mathematical operations. In this post, we will delve into the intricacies of the distributive property, providing examples, exercises, and tips to help you master this concept through our unique Distributive Property Equation Worksheet.
What is the Distributive Property?

At its core, the distributive property states that you multiply a sum by multiplying each term of the sum individually, then adding the results. The classic formula is:
a(b + c) = ab + ac
- a is the term outside the parentheses
- b and c are the terms inside the parentheses
This property is vital for simplifying expressions, solving equations, and understanding how numbers interact in algebra.
Why Learn the Distributive Property?

Mastery of the distributive property:
- Helps in simplifying complex equations
- Provides the foundation for factoring polynomials
- Is essential for advanced algebraic operations like FOIL (First, Outside, Inside, Last) for binomials
- Improves mental arithmetic skills by allowing you to break down larger numbers into smaller, manageable parts
Using Our Distributive Property Equation Worksheet
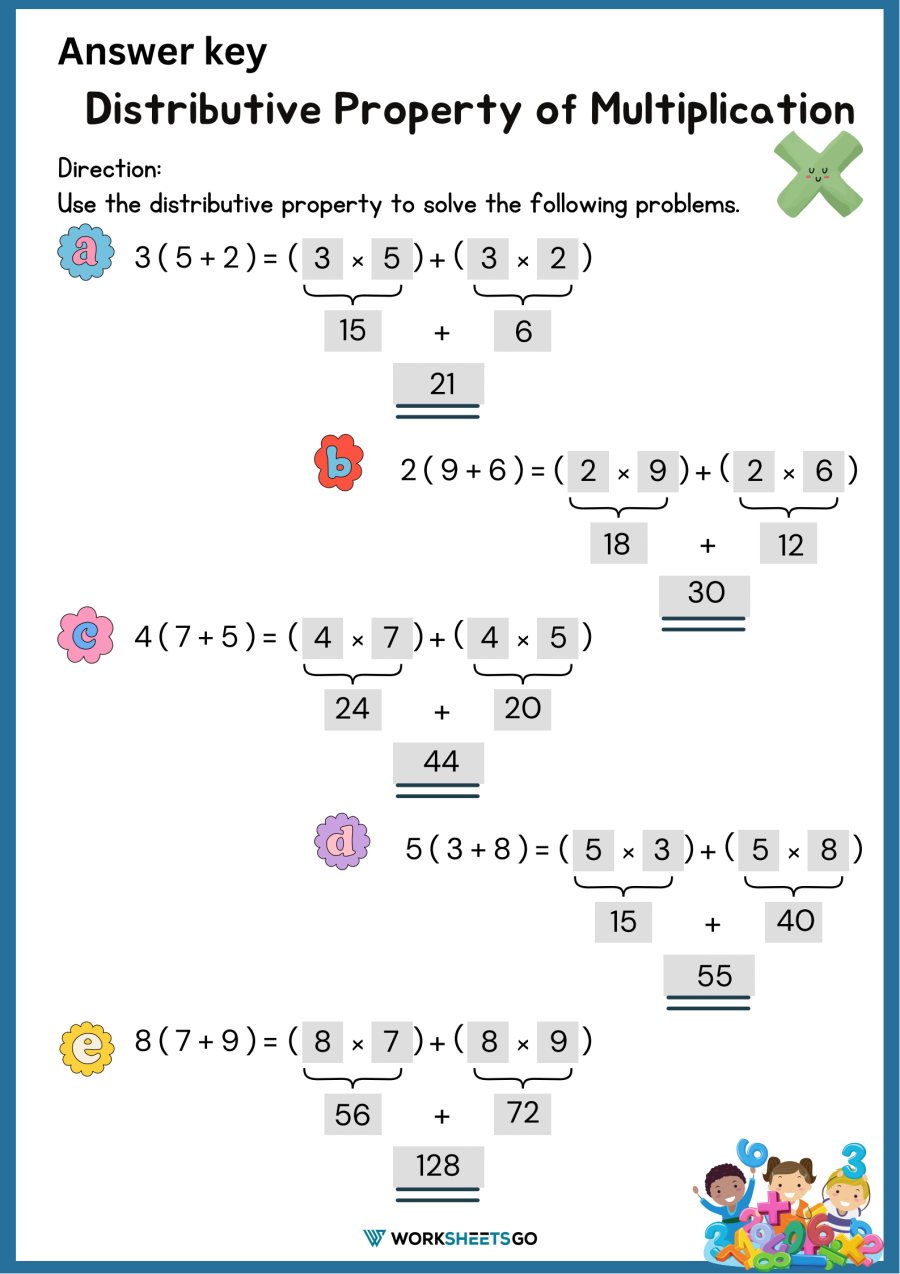
Our worksheet is designed to guide you through various scenarios where the distributive property can be applied. Here’s how to use it effectively:
Basic Exercises

Start with simple problems that involve distributing over two terms. For example:
- 2(3 + 4)
- 5(x - 2)
These exercises reinforce the basic application of the property and help students become comfortable with the concept.
Expanding to More Terms

Once comfortable, students can apply the property to expressions with more than two terms:
- 4(2x + y + z)
- -3(x + 2y - z + 3)
This helps in understanding how the property extends to multiple variables and constants.
Factoring as Reverse Distribution

We include exercises where students learn to factor expressions by pulling out a common factor:
- 6x + 9 = 3(2x + 3)
- 15ab + 10a = 5a(3b + 2)
This step is crucial for equation solving and simplifying complex expressions.
Real-World Applications
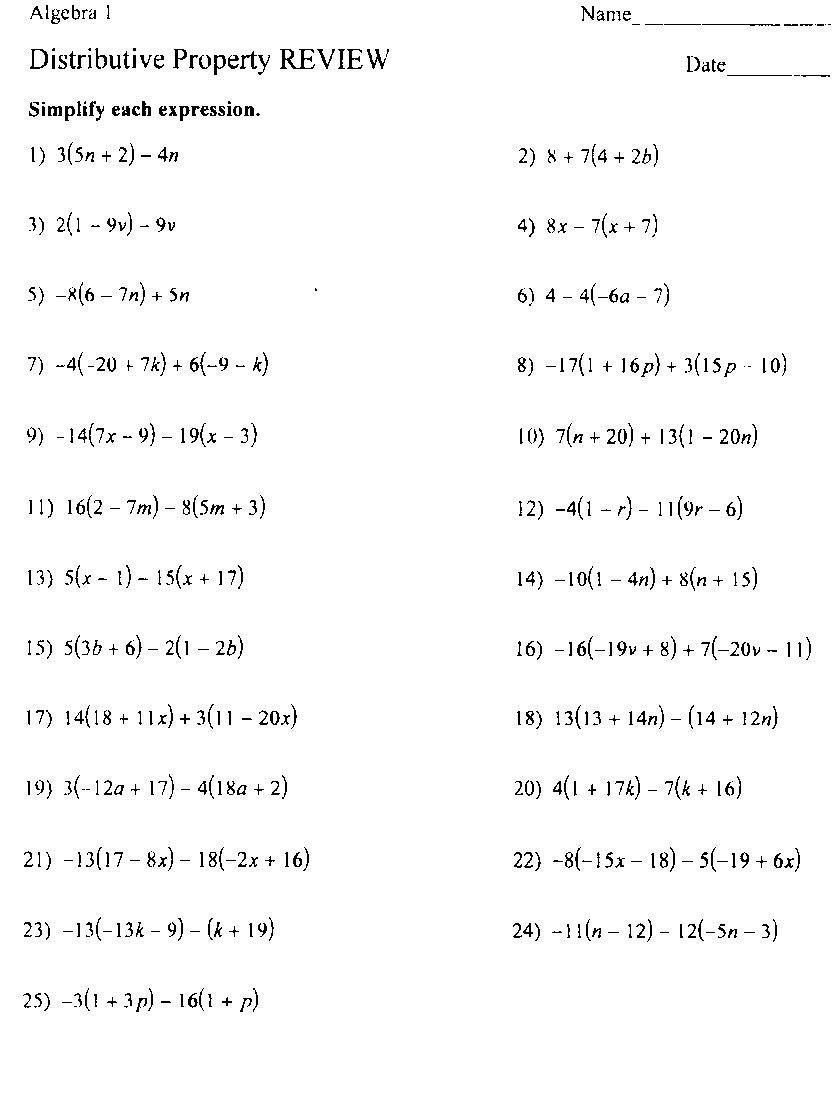
To make learning more tangible, our worksheet also includes:
- Word problems where students must translate a real-world scenario into a mathematical expression and solve it using the distributive property
- Visual exercises where students can see how the property works with geometric shapes, like rectangles
⚠️ Note: Make sure to show the steps clearly. For example, when factoring, show the common factor first, then the terms inside the parentheses.
Practical Tips for Mastering the Distributive Property

- Memorize the Basics: Understand the multiplication of basic numbers and variables.
- Practice Distribution and Factoring: Use the worksheet regularly to reinforce both distributing and factoring skills.
- Visualize: Use diagrams or physical objects to represent distributions, especially for visual learners.
- Keep Organized: Maintain clean and structured work, ensuring each step of distribution or factoring is clear.
- Check Work: After distributing or factoring, reverse the process to verify the results.
As we reach the end of our journey through the distributive property, it’s clear that this fundamental algebraic tool not only helps in solving equations but also in building a robust mathematical foundation. By using our Distributive Property Equation Worksheet, you've seen how this principle can be applied across a spectrum of algebraic expressions, enhancing your ability to solve, simplify, and understand mathematics at a deeper level. Remember, the key to mastering this property lies in consistent practice, clear visualization, and understanding its applications in both numerical and real-world scenarios.
What are some common mistakes when using the distributive property?

+
One common mistake is not distributing over all terms within the parentheses. For instance, 3(x + 2y) should result in 3x + 6y, not just 3x + 2y. Another frequent error is forgetting to distribute negative numbers, like -1(x + 2) should be -x - 2, not x + 2.
How do you practice factoring using the distributive property?

+
Start by identifying the common factor among the terms. Then, divide each term by this common factor to get the terms inside the parentheses. For example, for the expression 12x + 8y, you can factor out the common factor of 4: 4(3x + 2y). Practice this by reversing the distribution process regularly.
Can the distributive property be used in higher math?

+
Yes, the distributive property underpins many advanced algebraic operations. It is used in matrix multiplication, polynomial expansion, solving systems of linear equations, and even in calculus when dealing with derivatives and integrals. Understanding this property early on prepares you for these more complex applications.