Division Exponents Worksheet: Master Math Today

Exponents are a fundamental part of algebra, allowing us to write and solve problems involving repeated multiplication in a simplified manner. Understanding how to work with exponents is crucial for progressing in higher levels of mathematics, physics, chemistry, and various real-world applications. In this comprehensive guide, we'll explore the different types of exponentiation, particularly focusing on division with exponents, and provide you with an exponents worksheet to hone your skills.
What are Exponents?

An exponent is a number that indicates how many times a base number should be multiplied by itself. For instance, in the expression (2^3), the base is 2, and the exponent is 3, meaning (2^3 = 2 \times 2 \times 2 = 8). Here’s what you need to know about exponents:
- Base: The number being multiplied.
- Exponent or Power: The number of times to multiply the base.
- Formula: (a^n) where ‘a’ is the base and ‘n’ is the exponent.
The rules of exponents help us simplify and solve complex expressions. Today, our focus will be on the division of exponents, which is an essential operation.
Exponent Rules for Division

When dividing exponents, several rules come into play:
- Quotient of Powers: ( \frac{a^m}{a^n} = a^{m-n} )
- Power of a Quotient: ( \left( \frac{a}{b} \right)^n = \frac{a^n}{b^n} )
- Negative Exponents: ( a^{-n} = \frac{1}{a^n} )
Quotient of Powers

The quotient of powers rule states that when dividing two expressions with the same base, you subtract the exponents. Let’s illustrate this with an example:
- If ( x^5 \div x^2 = x^{5-2} = x^3 )
⚠️ Note: This rule applies only when the bases are the same.
Power of a Quotient

When the exponent applies to the whole fraction, you raise both the numerator and the denominator to that power:
- ( \left( \frac{3}{4} \right)^2 = \frac{3^2}{4^2} = \frac{9}{16} )
Negative Exponents

Negative exponents turn the expression into its reciprocal with a positive exponent:
- ( 5^{-2} = \frac{1}{5^2} = \frac{1}{25} )
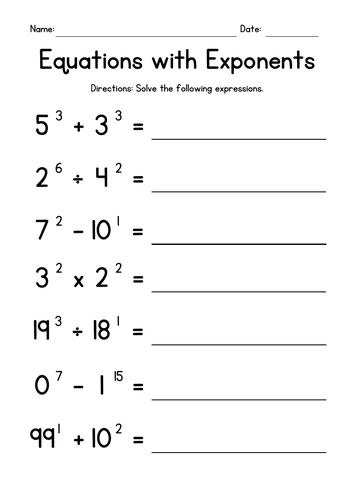
Dividing Exponents Worksheet

To become proficient in dividing exponents, practice is essential. Here’s an exponents worksheet tailored to help you master this skill:
| Problem | Answer |
|---|---|
| \frac{x^5}{x^2} | x^3 |
| \left( \frac{2}{3} \right)^3 | \frac{8}{27} |
| \frac{7^4}{7^2} | 7^2 = 49 |
| \frac{10^{-3}}{10^2} | 10^{-5} = \frac{1}{100000} |
| \left( \frac{1}{5} \right)^{-2} | 5^2 = 25 |

Steps to Solve Division of Exponents

- Identify the bases: Make sure the bases of the exponents are the same.
- Subtract the exponents: Apply the quotient of powers rule by subtracting the exponent in the denominator from the exponent in the numerator.
- Simplify: If possible, further simplify the expression according to other exponent rules.
- Negative Exponents: If the result has a negative exponent, rewrite it as a fraction.
- Check: Always double-check your work, especially when dealing with negative exponents or fractions.
🔍 Note: For complex expressions, it might be helpful to break down the problem into simpler steps before applying the rules.
Real-World Applications of Division of Exponents
Here are some scenarios where division with exponents plays a crucial role:
- Physics and Engineering: To understand compound interest, population growth, and decay.
- Chemistry: For solving problems involving concentration, rates of reactions, and equilibrium constants.
- Computer Science: When dealing with algorithms that involve logarithms or powers of numbers.
- Economics: In analyzing economic indicators where exponential growth or decay models are used.
By mastering the division of exponents, you not only strengthen your mathematical skills but also open up various fields of study and application. The ability to simplify and solve these mathematical operations quickly can significantly enhance your problem-solving capabilities in both academic and professional settings.
Now, let’s recap the key elements of our discussion:
Throughout this guide, we've covered the rules for dividing exponents, provided examples and a worksheet to help you practice, and highlighted the importance of understanding these concepts in various disciplines. Remember, proficiency comes with practice, and consistent application of these rules will make complex problems second nature.
What are the rules for dividing exponents with the same base?

+
When dividing exponents with the same base, use the quotient of powers rule: ( \frac{a^m}{a^n} = a^{m-n} ), where you subtract the exponent of the denominator from the exponent of the numerator.
How do you handle negative exponents when dividing?

+
A negative exponent turns the expression into its reciprocal with a positive exponent: ( a^{-n} = \frac{1}{a^n} ). Thus, when dividing, you can deal with negative exponents by moving them to the denominator to make them positive.
Why is it important to understand exponent rules?

+
Exponent rules are fundamental in algebra, simplifying expressions, solving equations, and are crucial in fields like physics, engineering, finance, and more where exponential growth or decay models are common.