Order of Operations Worksheets With Answers for 7th Grade
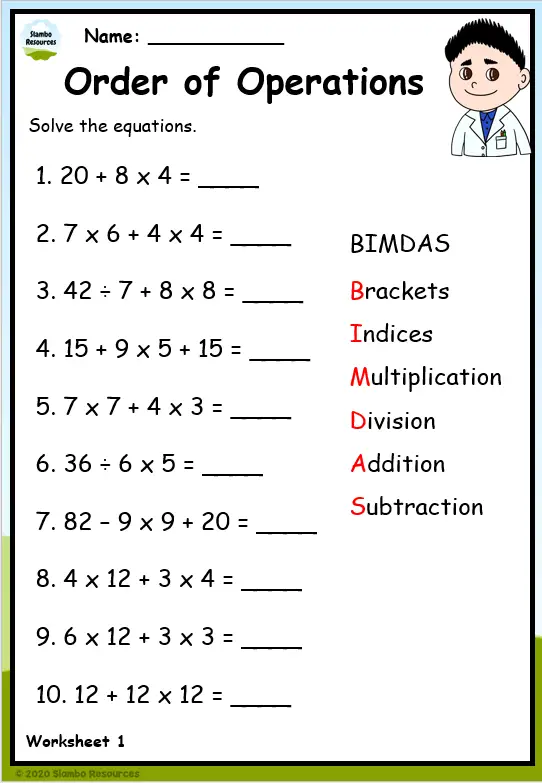
In the world of mathematics, understanding the order in which operations are performed is crucial. For seventh graders, this concept, known as the order of operations, ensures that mathematical expressions are evaluated uniformly. This blog post will guide you through creating order of operations worksheets tailored for 7th-grade students, complete with answers to facilitate learning.
Why the Order of Operations Matters
The order of operations, often remembered by the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right)), dictates how calculations are carried out. Here are some reasons why this is fundamental:
- Consistency: Ensures that everyone gets the same result when solving an expression.
- Clarity: Reduces the chances of misinterpretation in mathematical expressions.
- Foundation for Higher Math: Provides a basis for more advanced mathematical concepts in algebra and beyond.

Designing Your Worksheets

When crafting your order of operations worksheets for 7th graders, consider these points:
- Use relatable contexts: Make problems relevant to their daily life to keep engagement high.
- Increase complexity gradually: Start with basic PEMDAS exercises and then integrate more challenging operations.
- Provide answers: This allows students to self-assess their understanding and progress.
Steps to Create Effective Worksheets
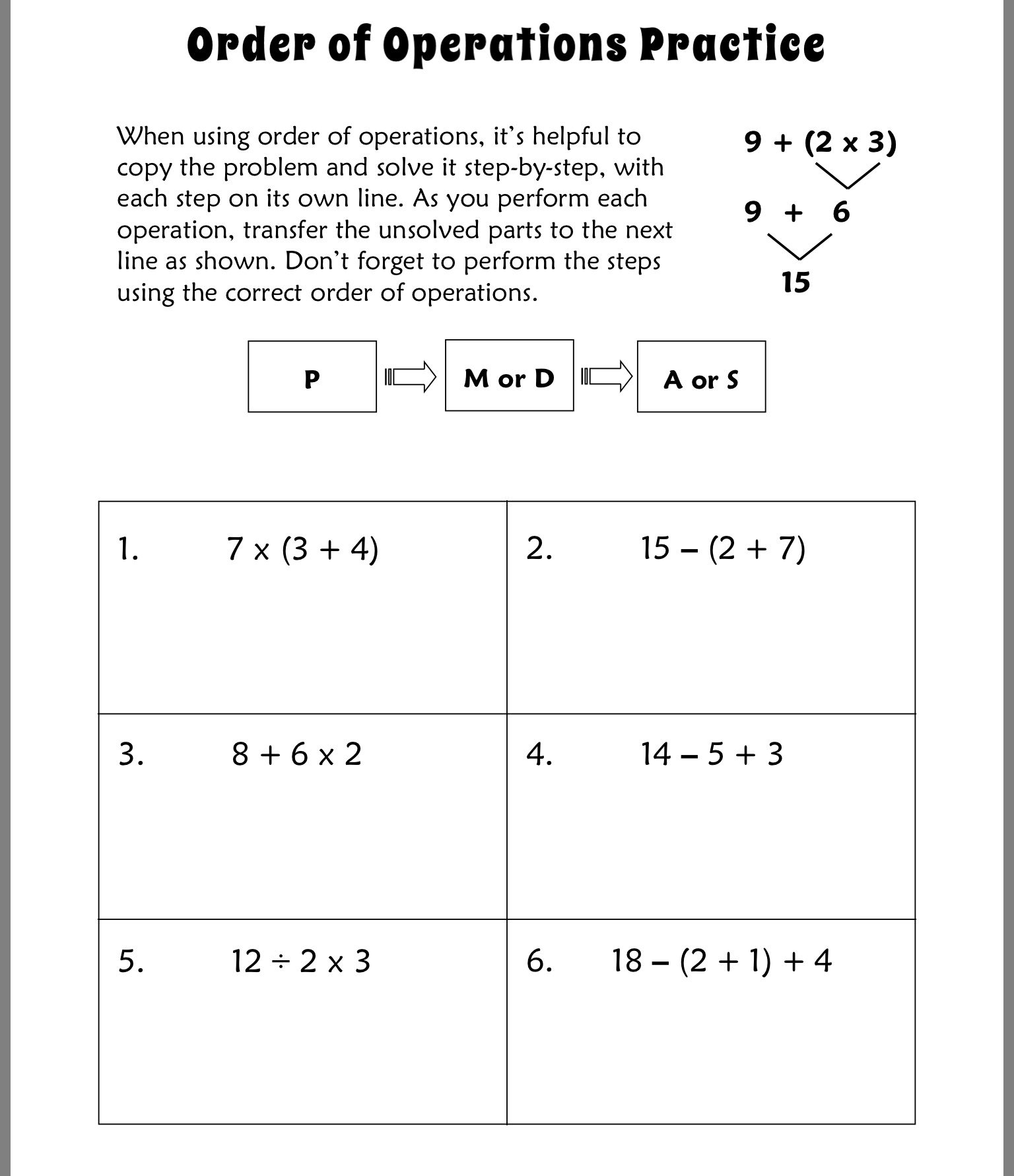
Here is how to design worksheets that both teach and test the order of operations:
- Identify the Learning Objectives: Clarify what aspects of PEMDAS you want to focus on.
- Create a Variety of Problems:
- Basic problems using all operations (PEMDAS)
- Problems with parentheses, brackets, or braces
- Expressions with exponents or roots
- Include Word Problems: Help students understand how to apply PEMDAS in real-world scenarios.
- Provide Step-by-Step Solutions: Showing detailed steps is beneficial for visual learners.
| Problem | Step-by-Step Solution | Final Answer |
|---|---|---|
| 2 + 3 * 4 | Step 1: 3 * 4 = 12 Step 2: 2 + 12 = 14 |
14 |
| (6 - 2)^2 / 4 | Step 1: 6 - 2 = 4 Step 2: 4^2 = 16 Step 3: 16 / 4 = 4 |
4 |

🖊️ Note: Ensure problems match the learning stage of your 7th graders, avoiding overly complex expressions at the beginning.
Word Problems and Real-Life Application

Word problems are a great way to engage students by placing mathematical concepts in scenarios they can relate to:
- Buying items at a store with discounts
- Calculating distances on a trip considering speed and time
- Problems involving recipe adjustments
Sample Word Problem

Sam wants to buy a shirt on sale at a 25% discount. The shirt originally costs 50. How much will the shirt cost after the discount?</p> <ul> <li>Step 1: Calculate the discount amount: 50 * 0.25 = 12.50
Enhancing Engagement and Learning
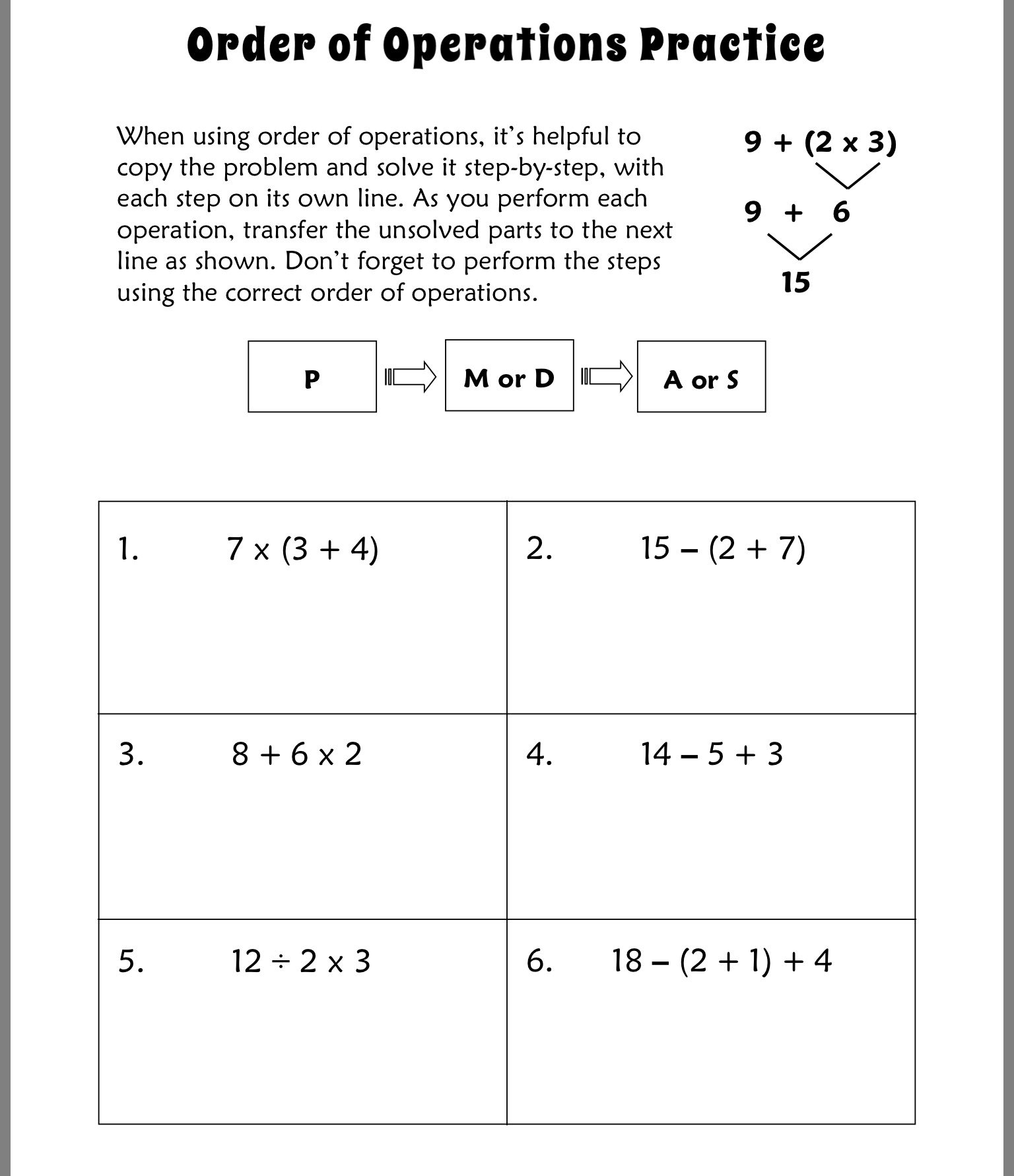
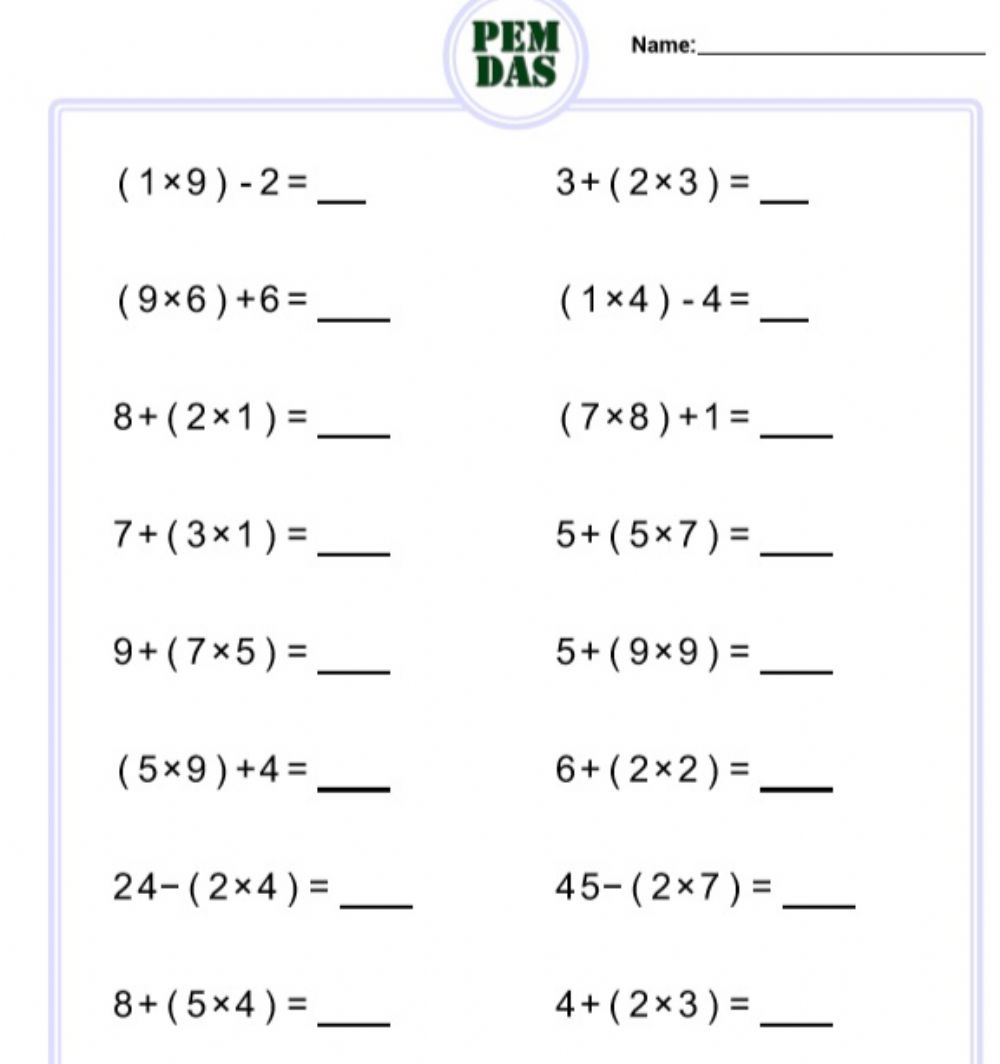
Creating interactive or fun elements can boost learning:
- Create challenges or competitions
- Incorporate puzzles or riddles
- Use colorful diagrams or charts to illustrate the concept
🎓 Note: Interactive learning tools can help students remember PEMDAS better than rote memorization alone.
To wrap up, creating order of operations worksheets with answers for 7th-grade students not only reinforces the basic principles of PEMDAS but also prepares them for more advanced mathematical tasks. It encourages consistency, clarity, and a deep understanding of how operations interact, setting a strong foundation for future learning. Remember to keep your worksheets engaging and varied, always showing the steps involved in solving problems to demystify the process for students.
Why is the order of operations important in real life?

+
The order of operations is essential in real life for ensuring that calculations involving multiple operations yield consistent and correct results. It’s used in fields like finance, engineering, and everyday tasks such as cooking or budgeting.
Can the order of operations change?

+
The order of operations, as defined by PEMDAS, does not change. However, the way we interpret and use it in higher math or specific contexts might involve additional rules or conventions.
What are some common mistakes students make with the order of operations?

+
Common errors include:
- Not performing multiplication or division before addition or subtraction from left to right
- Misinterpreting the brackets (parentheses, brackets, braces)
- Mistaking the precedence of operations like roots and exponents