5 Simple Steps to Master Dividing Rational Numbers

Dividing rational numbers might seem daunting at first, but with the right steps and understanding, it can become a simple task. Here are five steps to help you master this mathematical operation, ensuring your calculations are both accurate and efficient.
1. Convert the Division into Multiplication
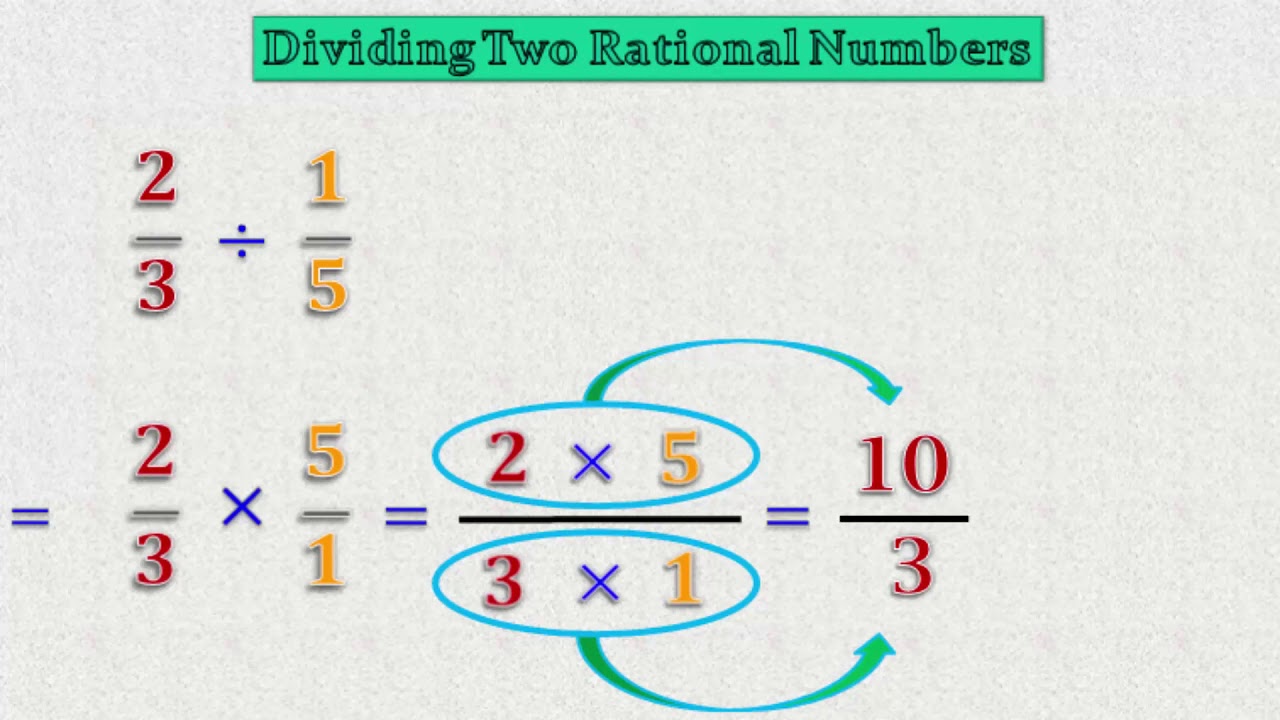
Remember that dividing by a number is the same as multiplying by its reciprocal. This is the foundational step in dividing rational numbers:
- Identify the divisor.
- Find its reciprocal. For example, if you’re dividing by ( \frac{3}{4} ), the reciprocal is ( \frac{4}{3} ).
- Instead of dividing, you will multiply the dividend by this reciprocal.
💡 Note: The reciprocal is obtained by swapping the numerator and the denominator.
2. Handle Mixed Numbers if Applicable

Mixed numbers can complicate the process unless they are converted into improper fractions. Here’s how you do it:
- Multiply the whole number part by the denominator of the fractional part.
- Add the numerator of the fractional part to this result.
- Place this sum over the original denominator.
3. Perform the Multiplication
Now that you have two fractions or improper fractions:
- Multiply the numerators together to get the new numerator.
- Multiply the denominators together to get the new denominator.
🔎 Note: Simplify this new fraction if possible, by dividing both the numerator and the denominator by their greatest common divisor.
4. Simplify the Result

To ensure your answer is in its simplest form:
- Factorize both the numerator and the denominator.
- Cancel out any common factors.
| Numerator | Denominator |
|---|---|
| 30 | 60 |
| 30 ÷ 30 = 1 | 60 ÷ 30 = 2 |

In this case, dividing both by their common factor of 30, \frac{30}{60} simplifies to \frac{1}{2} .
5. Convert Back to a Mixed Number or Express in Decimal Form
If your result was an improper fraction and you need a whole number or mixed number:
- Divide the numerator by the denominator to find the whole number part.
- The remainder becomes the new numerator, with the original denominator staying the same.
🧮 Note: When converting to a decimal, remember to keep as many decimal places as needed for accuracy or round appropriately.
Throughout this process, understanding the properties of rational numbers, like the commutative property of multiplication or the concept of inverse operations, can greatly enhance your speed and accuracy. Keep practicing these steps, and soon, dividing rational numbers will feel as natural as basic arithmetic.
Why is it important to convert division into multiplication when dividing fractions?

+
Dividing by a number is equivalent to multiplying by its reciprocal due to the fundamental property of fractions. This makes division easier because you’re performing a simpler multiplication operation.
Can I skip the step of simplifying fractions during the division process?
+
You can, but simplifying at the end or at intermediate steps can make the numbers you deal with smaller and the process less error-prone. However, it’s not strictly necessary if accuracy is your only goal.
How do I handle negative fractions when dividing?

+
Treat the negative sign as you would in regular arithmetic: the result will be negative if both the numerator and the denominator of one fraction are negative or if one is negative and one is positive. When dividing, change the division to multiplication by the reciprocal, and then follow the rules for signs in multiplication.