Decimal to Fraction Conversion Made Easy

Understanding Decimal to Fraction Conversion

Decimal to fraction conversion is a fundamental concept in mathematics that involves converting a decimal number into a fraction. This process is essential in various mathematical operations, such as adding, subtracting, multiplying, and dividing fractions. In this article, we will explore the step-by-step process of converting decimals to fractions, along with examples and tips to make the process easier.

Why Convert Decimals to Fractions?

Converting decimals to fractions is necessary for several reasons:

• Simplifying calculations: Fractions can be easier to work with than decimals, especially when performing complex calculations.
• Accurate representation: Fractions can provide a more accurate representation of certain values, such as pi (π) or e.
• Mathematical operations: Fractions are required for certain mathematical operations, like finding the greatest common divisor (GCD) or least common multiple (LCM).

Step-by-Step Process of Decimal to Fraction Conversion

Converting decimals to fractions involves a simple step-by-step process:

1. Write the decimal as a fraction: Start by writing the decimal as a fraction with the decimal number as the numerator and 1 as the denominator.
2. Multiply by a power of 10: Multiply the numerator and denominator by a power of 10 to eliminate the decimal point.
3. Simplify the fraction: Simplify the fraction by dividing the numerator and denominator by their greatest common divisor (GCD).

📝 Note: The power of 10 used in step 2 will depend on the number of decimal places in the original decimal number.

Examples of Decimal to Fraction Conversion

Let’s consider a few examples to illustrate the process:

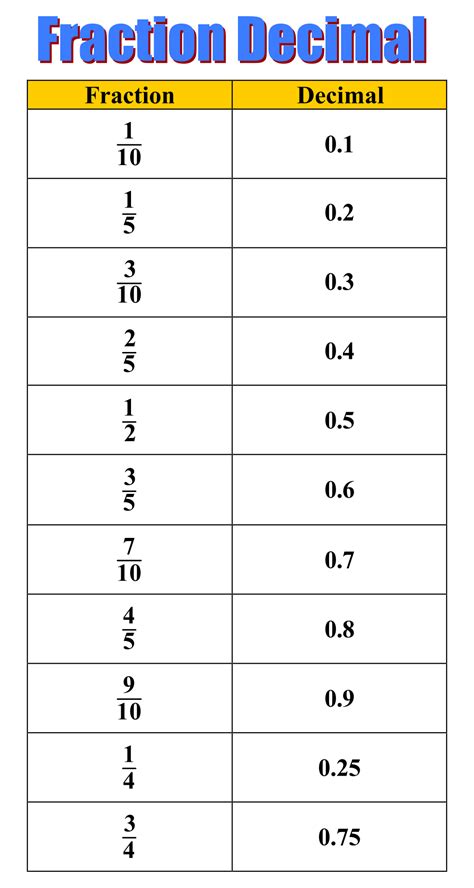
• Example 1: Convert 0.5 to a fraction
  • Write the decimal as a fraction: 0.⁵⁄₁
  • Multiply by a power of 10: ⁵⁄₁₀
  • Simplify the fraction: ¹⁄₂
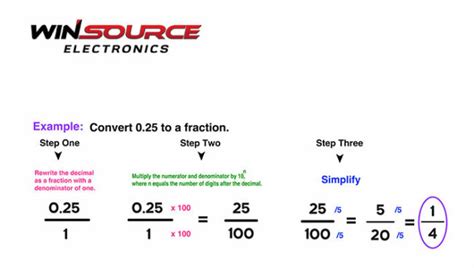
• Example 2: Convert 0.25 to a fraction
  • Write the decimal as a fraction: 0.²⁵⁄₁
  • Multiply by a power of 10: ²⁵⁄₁₀₀
  • Simplify the fraction: ¹⁄₄
• Example 3: Convert 0.125 to a fraction
  • Write the decimal as a fraction: 0.¹²⁵⁄₁
  • Multiply by a power of 10: ¹²⁵⁄₁₀₀₀
  • Simplify the fraction: ¹⁄₈

Tips and Tricks for Decimal to Fraction Conversion

Here are some tips and tricks to make decimal to fraction conversion easier:

• Use a calculator: Use a calculator to perform the calculations and simplify the fraction.
• Look for patterns: Look for patterns in the decimal number to determine the equivalent fraction.
• Use mental math: Use mental math to estimate the equivalent fraction and then simplify it.

Common Mistakes to Avoid

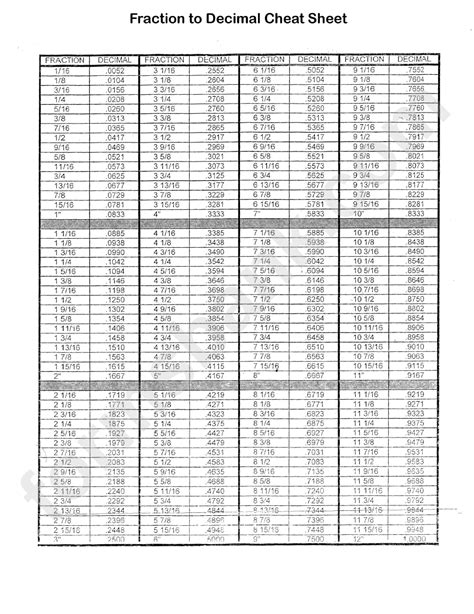
Here are some common mistakes to avoid when converting decimals to fractions:

• Not multiplying by a power of 10: Failing to multiply the numerator and denominator by a power of 10 can result in an incorrect fraction.
• Not simplifying the fraction: Failing to simplify the fraction can result in a complex fraction that is difficult to work with.
• Rounding errors: Rounding errors can occur when converting decimals to fractions, so it’s essential to be precise.

Conclusion

Decimal to fraction conversion is a fundamental concept in mathematics that involves converting a decimal number into a fraction. By following the step-by-step process and using tips and tricks, you can easily convert decimals to fractions. Remember to avoid common mistakes and use mental math to estimate the equivalent fraction.

What is the purpose of decimal to fraction conversion?

+

The purpose of decimal to fraction conversion is to simplify calculations, provide accurate representation, and enable mathematical operations that require fractions.

How do I convert a decimal to a fraction?

+

To convert a decimal to a fraction, write the decimal as a fraction with the decimal number as the numerator and 1 as the denominator, multiply by a power of 10, and simplify the fraction.

What are some common mistakes to avoid when converting decimals to fractions?

+

Related Terms:

• Decimal to fraction worksheet pdf