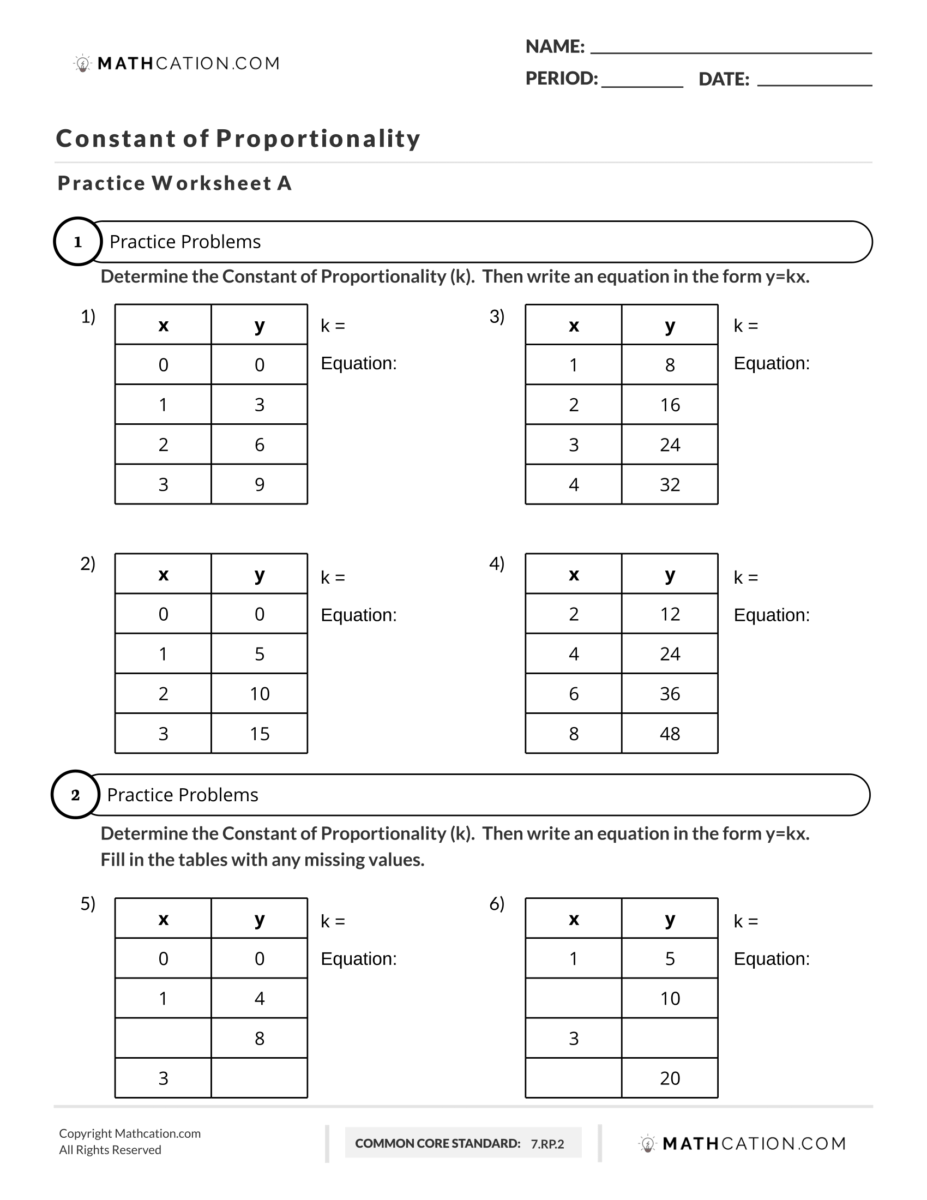
5 Essential Tips for Mastering CPCTC Proofs Worksheets

Mastering CPCTC proofs, or "Corresponding Parts of Congruent Triangles are Congruent," is an integral part of geometry. This mathematical concept is pivotal for students who are delving deeper into the subject, as it provides the groundwork for proving that parts of congruent triangles are congruent. Understanding and applying CPCTC in proofs not only enhances logical reasoning but also boosts problem-solving skills. Here are five essential tips to help you excel in using CPCTC proofs worksheets effectively:
1. Understand the Basics of Congruent Triangles


Before you can effectively use CPCTC in proofs, you must first understand how to identify congruent triangles. Here are the key points:
- SSS (Side-Side-Side): If all three sides of one triangle are congruent to all three sides of another triangle, the triangles are congruent.
- SAS (Side-Angle-Side): If two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, the triangles are congruent.
- ASA (Angle-Side-Angle): If two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, the triangles are congruent.
- AAS (Angle-Angle-Side): If two angles and a non-included side of one triangle are congruent to two angles and the corresponding side of another triangle, the triangles are congruent.
- HL (Hypotenuse-Leg): Applicable to right triangles, if the hypotenuse and one leg are congruent to the hypotenuse and one leg of another triangle, the triangles are congruent.
💡 Note: Remember that CPCTC is used after proving triangles are congruent, not to prove the congruence itself.
2. Utilize Visual Aids and Diagrams


Visual aids are invaluable for CPCTC proofs:
- Draw clear and accurate diagrams for the given problem.
- Label all the relevant parts of the triangles to visualize the congruence.
- Use different colors or line styles to distinguish between what is given and what needs to be proved.
- Remember that a picture is worth a thousand words when it comes to understanding geometric relationships.
3. Start with a Strategy

Approaching a CPCTC proof without a strategy can lead to confusion:
- Identify the triangles involved in the proof.
- List all known information, marking the diagram accordingly.
- Determine which congruence postulate (SSS, SAS, ASA, AAS, HL) applies to prove the triangles are congruent.
- Once congruence is established, use CPCTC to prove the remaining parts of the triangles congruent.
4. Practice Structured Proof Writing
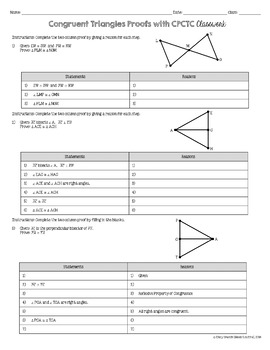
Writing a CPCTC proof in a structured manner is crucial:
- Begin with the Given and Prove statements.
- Outline each step logically:
- State any given information.
- List any geometric theorems or postulates you use.
- Write out the statements and reasons side by side for clarity.
- Make sure to write “CPCTC” as the reason when proving that corresponding parts are congruent after establishing triangle congruence.
✍️ Note: Practice writing proofs out on worksheets, focusing on clear, concise statements and accurate reasoning.
5. Apply Logical Reasoning Consistently

Logical reasoning is the backbone of CPCTC proofs:
- Be clear on what you are given and what you need to prove.
- Use theorems and postulates logically and in sequence.
- When you reach CPCTC, ensure you’ve established triangle congruence.
- Do not skip steps; every statement should have a corresponding reason.
CPCTC proofs are not just about proving that triangles are congruent but also about understanding the logical flow of mathematics. With practice and these tips, you can navigate through CPCTC proofs with greater ease.
By mastering these techniques, students will find themselves not only better prepared for their geometry coursework but also equipped with enhanced analytical skills applicable in various academic and real-world scenarios. CPCTC is more than just a proof technique; it's a gateway to logical reasoning and problem-solving in the realm of geometry.
What does CPCTC stand for?

+
CPCTC stands for “Corresponding Parts of Congruent Triangles are Congruent.” It’s used after proving two triangles congruent to state that their corresponding parts are congruent as well.
Can CPCTC be used as a standalone method to prove triangle congruence?

+
No, CPCTC is not used to prove triangle congruence; instead, it’s applied after proving congruence by one of the postulates (SSS, SAS, ASA, etc.).
Why is drawing diagrams important for CPCTC proofs?

+
Diagrams visually represent geometric relationships, making it easier to identify congruent parts, angles, and sides, which helps in structuring and understanding the proof.