7 Essential Tips for Complex Numbers in Algebra 2

Algebra 2 introduces students to a fascinating realm of mathematics through the study of complex numbers. These entities, which are neither real nor integers, expand the horizon of what we can understand in algebra and beyond. Grasping complex numbers is not just an academic exercise; they are foundational in numerous fields like electrical engineering, quantum physics, and signal processing. Here, we will delve into seven essential tips to master complex numbers, ensuring you not only understand them but can apply them confidently in your studies.
1. Understand the Basics of Complex Numbers


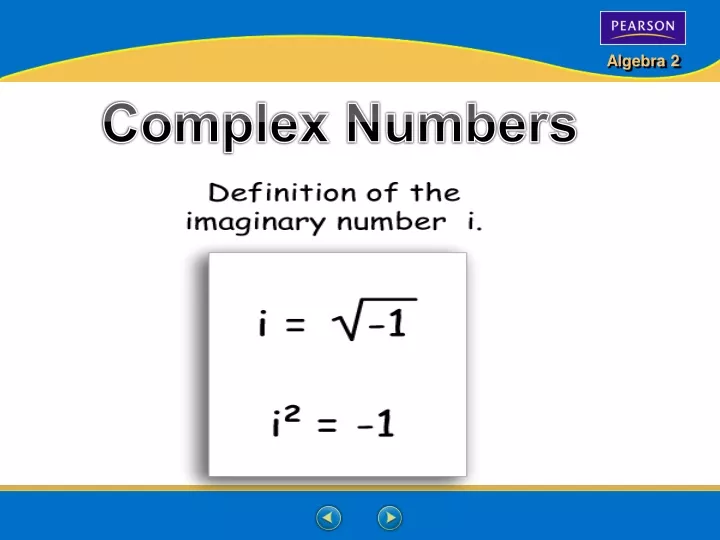
Before diving deep, it’s crucial to understand what a complex number is. A complex number is expressed as:
a + bi
- a represents the real part.
- b represents the imaginary part.
- i is the imaginary unit, where i2 = -1.
Complex numbers extend the real number line into a two-dimensional plane, where the x-axis represents the real part, and the y-axis the imaginary part. Getting a firm grip on this concept sets the foundation for all subsequent operations.
2. Master the Addition and Subtraction of Complex Numbers

| Example | Operation | Result |
|---|---|---|
| (5 + 3i) + (2 - 6i) | Addition | (5 + 2) + (3 - 6)i = 7 - 3i |
| (5 + 3i) - (2 - 6i) | Subtraction | (5 - 2) + (3 + 6)i = 3 + 9i |
Here’s how you perform these operations:
- Add the real parts together.
- Add the imaginary parts together.
- The same principle applies to subtraction.
3. Learn Multiplication of Complex Numbers
When multiplying complex numbers, you use the FOIL method:
- First: Multiply the first terms.
- Outer: Multiply the outer terms.
- Inner: Multiply the inner terms.
- Last: Multiply the last terms.
Remember to handle the imaginary part correctly:
(a + bi) × (c + di) = ac + adi + bci + bdi²
Since i2 = -1:
= (ac - bd) + (ad + bc)i
💡 Note: Complex number multiplication is not the same as multiplying real numbers; you must account for the interplay between the real and imaginary parts.
4. Understand Conjugation and Its Applications


The conjugate of a complex number a + bi is a - bi. Here’s why it’s essential:
- Division: Conjugation allows you to perform division through rationalization.
- Roots: Conjugation helps in finding roots of polynomial equations.
Conjugation transforms the complex plane, flipping signs of the imaginary part, which can simplify many operations.
5. Work with Complex Plane

Visualize complex numbers on the Argand diagram, where:
- The horizontal axis represents the real part.
- The vertical axis represents the imaginary part.
This visualization can help understand:
- Magnitudes or Moduli.
- Arguments or Phases.
🧭 Note: The magnitude of a complex number z = a + bi is |z| = √(a² + b²), which is the distance from the origin in the complex plane.
6. Use Complex Numbers in Polynomial Equations

Complex numbers unlock new solutions to polynomial equations. Here’s how:
- The Fundamental Theorem of Algebra states every non-constant single-variable polynomial with complex coefficients has at least one complex root.
- Knowing how to find these roots expands the potential solutions to equations, especially those with no real solutions.
Example: If z = 2 + 3i is a root, then 2 - 3i must also be a root due to the Conjugate Root Theorem.
7. Apply Complex Numbers in Real-world Scenarios

Complex numbers aren’t just theoretical constructs; they have practical applications:
- In electrical engineering, to model alternating currents.
- In quantum mechanics, where complex numbers help to describe the state of particles.
- In signal processing, where Fourier Transforms use complex numbers to analyze and manipulate signals.
Understanding these applications can make learning complex numbers more exciting and relevant.
In our exploration of complex numbers, we've covered the core operations, theoretical underpinnings, and real-world applications. This knowledge isn't just for solving academic problems; it's a gateway to understanding advanced mathematical concepts and their practical uses. Remember, the more you engage with complex numbers, the more intuitive they become, unveiling a richer, more complete understanding of mathematics. Whether you're tackling complex equations, visualizing in the complex plane, or solving real-world problems, these tips are your allies. Embrace them, practice, and watch as complex numbers become a familiar, invaluable tool in your mathematical toolkit.
What are the main components of a complex number?

+
Complex numbers consist of two parts: the real part and the imaginary part. These are denoted as a and bi respectively, where a and b are real numbers, and i is the imaginary unit with the property that i2 = -1.
How do you find the magnitude of a complex number?

+
The magnitude or modulus of a complex number z = a + bi is calculated as |z| = √(a² + b²), which is the distance from the origin on the complex plane.
Why are complex numbers important in engineering?

+
Complex numbers are crucial in engineering for tasks like analyzing electrical circuits, designing control systems, and in signal processing where they facilitate the use of Fourier transforms to analyze signal components.