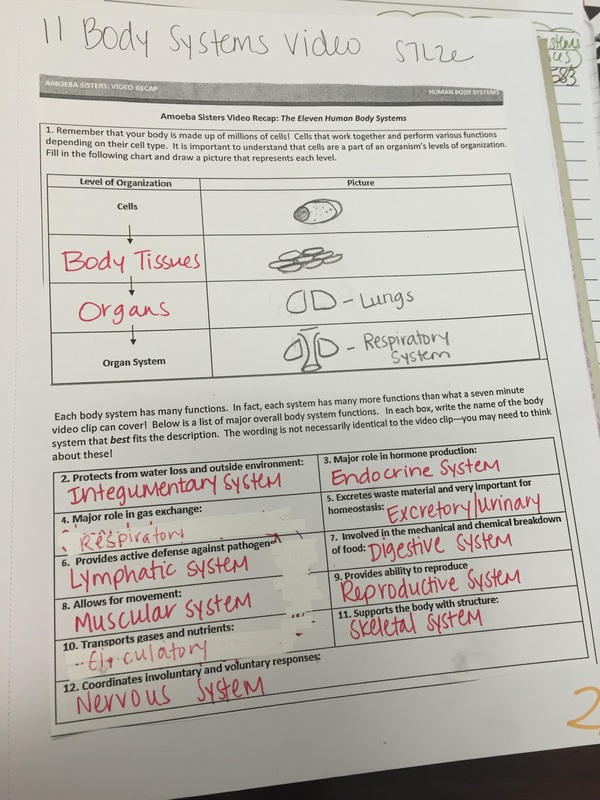
5 Ways to Master Combining Like Terms in Algebra 1

Mastering the art of combining like terms in Algebra 1 is not just a fundamental skill; it's a gateway to understanding higher mathematical concepts. For students stepping into the realm of algebra, the ability to simplify expressions by combining like terms is crucial. This technique reduces complexity, allowing for easier problem-solving and manipulation of equations. Let's dive into five effective methods to excel in this foundational aspect of algebra.
Understanding Like Terms

Before we combine terms, it's essential to understand what like terms are. Like terms in algebra refer to expressions that have identical variables raised to the same power. Here’s what to look for:
- Variable consistency: Terms like 3x and 7x are like terms because they both contain the variable x to the first power.
- Coefficient changes: The numerical multiplier in front of the variable can vary, but it does not change the nature of the term.

⚠️ Note: Remember, terms with different exponents or variables are not like terms. For example, 2x^2 and 5x are not like terms because the exponents are different.
Method 1: The Box Method

The box method provides a visual approach to combining like terms:
- Draw a large box.
- Divide the box into compartments, one for each unique variable in the expression.
- List all the terms with that variable in their respective compartments.
- Combine the coefficients of like terms within each compartment.
| Variable | Terms | Combined Coefficient |
|---|---|---|
| x | 3x, -2x, 5x | 6x |
| y | 4y, -y | 3y |
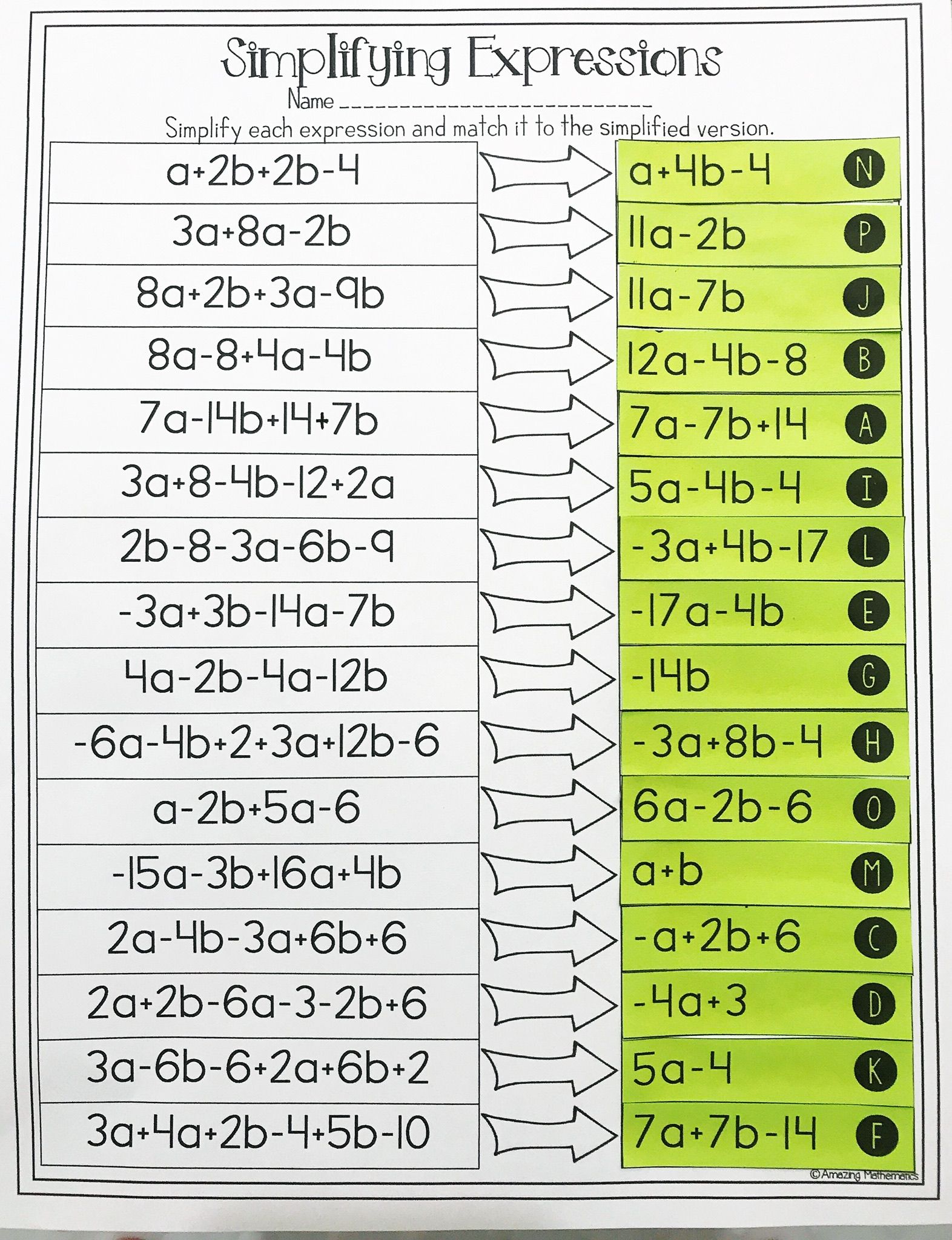
📝 Note: This method can be particularly helpful for visual learners as it organizes the expression into manageable parts.
Method 2: Color Coding

Another useful technique is color coding:
- Assign a unique color to each variable in your expression.
- Write out the expression in colors to easily see which terms belong together.
- Group and combine the like terms with the same color.
Here's an example:
- 3x
- -2x
- 4y
- -y
Combining the red terms gives x, and the green terms give 3y. The result is x + 3y.
Method 3: Substitution
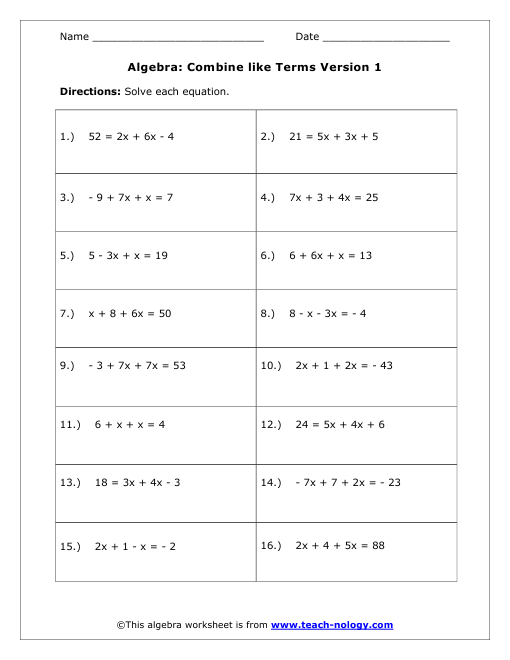
Substitution can simplify expressions:
- Introduce new variables to represent groups of like terms.
- Combine these new variables and then back-substitute to find the original expression.
Let's say you have 3x + 5x - 2y + 4y:
- Let a = 3x + 5x and b = -2y + 4y.
- Combine to get a + b = 8x + 2y.
Method 4: Distribution

The distribution property helps when terms are within brackets:
- Distribute the coefficient across the terms inside the parentheses.
- Then, combine like terms as usual.
Example: 3(2x - y) + 4x - 2y
- Distribute: 6x - 3y + 4x - 2y
- Combine like terms: 10x - 5y
Method 5: Identify Patterns

Recognizing patterns can make combining like terms faster:
- Identify common structures in expressions, like binomial expansion or difference of squares.
- Quickly combine terms by understanding how patterns behave under algebraic manipulation.
For instance, recognizing x^2 - y^2 = (x + y)(x - y) can simplify combining terms significantly.
💡 Note: Practicing pattern recognition enhances your ability to combine like terms rapidly.
By mastering these five methods, you not only improve your skill in combining like terms but also develop a deeper understanding of algebraic manipulation. Whether you prefer visual techniques like the box method or color coding, or more abstract methods like substitution and pattern recognition, each approach offers unique advantages. Remember, the key to fluency in algebra lies in consistent practice and the ability to choose the right method for the problem at hand. With time, these techniques will become second nature, paving the way for you to tackle more complex algebraic challenges with confidence.
What are like terms in algebra?

+
Like terms are expressions that have identical variables raised to the same power. For example, (3x) and (7x) are like terms because both have (x) to the first power.
Why is it important to combine like terms?

+
Combining like terms simplifies algebraic expressions, making them easier to work with and understand. It’s crucial for solving equations, evaluating expressions, and manipulating formulas in algebra.
Can you combine terms with different variables?

+
No, terms with different variables or different exponents of the same variable cannot be combined. They are unlike terms and must remain separate in an expression.
How does pattern recognition help in algebra?

+
Recognizing patterns allows for quicker manipulation of expressions, as common algebraic identities can be applied directly to simplify or expand terms, making problem-solving more efficient.
What can be done if there are no like terms to combine?
+
If there are no like terms, the expression is already in its simplest form. Further simplification might involve other algebraic techniques like factoring or applying identities.