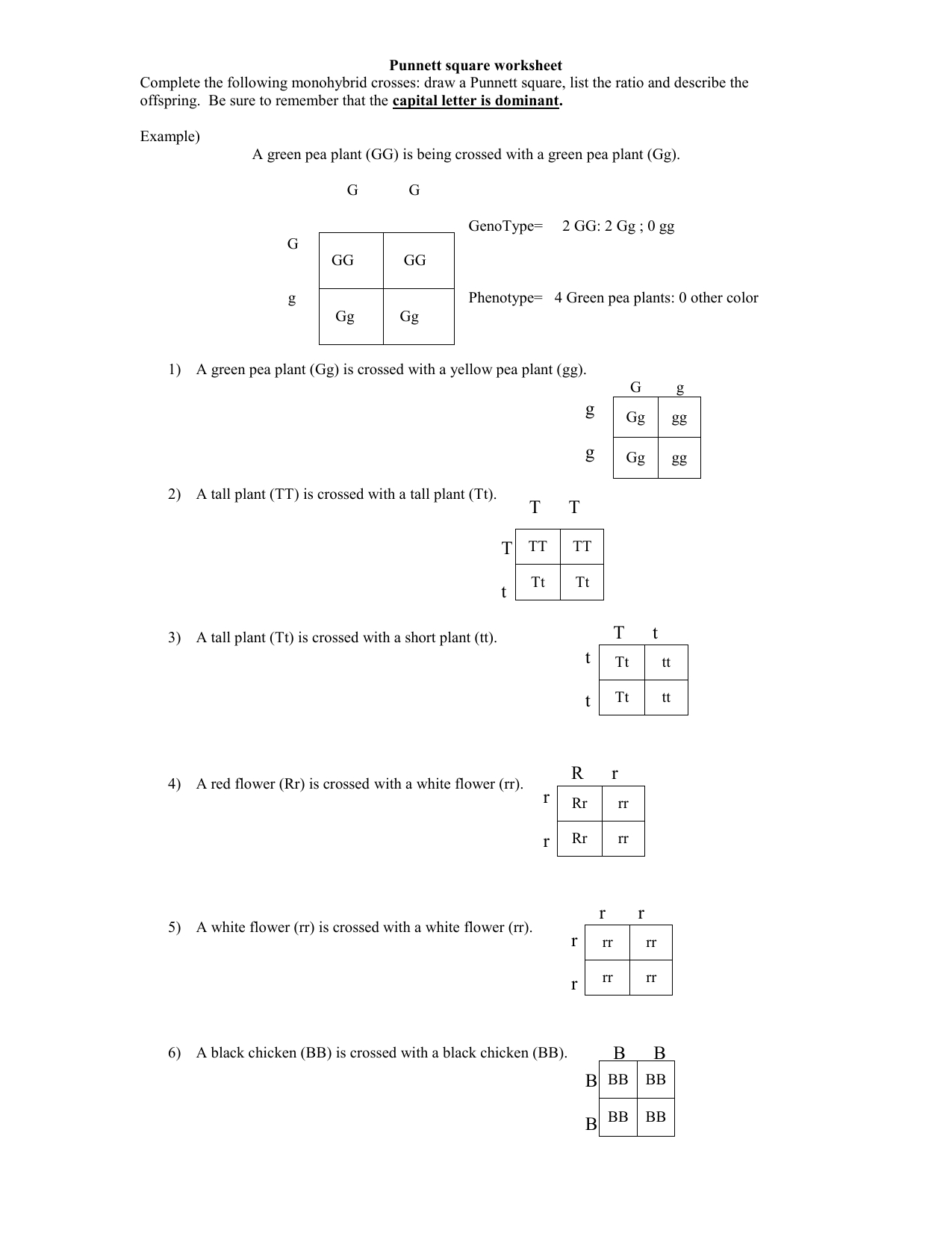
Charles Law Worksheet: Simplified Gas Law Explanation

In the world of physics and chemistry, Charles' Law stands as a cornerstone, particularly when we discuss the behavior of gases. Named after the French scientist Jacques Charles, this law outlines the relationship between the volume of a gas and its temperature, when the pressure and the amount of gas remain constant. Here, we explore an in-depth Charles Law Worksheet aimed at simplifying this fundamental gas law for students and enthusiasts alike.
Understanding Charles’ Law


Charles’ Law can be summarized with the formula:
V1/T1 = V2/T2
Where:
- V1 is the initial volume of the gas
- T1 is the initial temperature (in Kelvin)
- V2 is the final volume after temperature change
- T2 is the final temperature (in Kelvin)
This means that as the temperature of a gas increases, so does its volume, and vice versa, assuming the pressure is held constant. Here’s how it applies:
Real-World Applications

- Balloon Inflation: A balloon expands when placed in hot water as the temperature of the air inside increases.
- Weather Balloons: They rise as they ascend into colder regions of the atmosphere, expanding and eventually bursting.
- Hot Air Balloons: The pilot controls the lift by heating the air inside the balloon, thereby increasing the volume of the hot air.
Charles’ Law Worksheet

Let’s dive into some practical examples using Charles’ Law:
Example Problem

A gas occupies a volume of 150 mL at a temperature of 27°C. If the temperature is increased to 127°C at the same pressure, what will be the new volume?
| Step | Process |
|---|---|
| 1 | Convert temperatures to Kelvin: V1 = 150 mL T1 = 27 + 273.15 = 300.15 K T2 = 127 + 273.15 = 400.15 K |
| 2 | Set up the equation: V2 = (V1 * T2)/T1 |
| 3 | Calculate the final volume: V2 = (150 mL * 400.15 K) / 300.15 K ≈ 200.15 mL |

📢 Note: The volume of the gas increases because the temperature increased while the pressure remained constant.
Another Example

If the volume of a gas at 25°C is 2.5 liters, what would be its volume at 100°C?
- V1 = 2.5 L
- T1 = 25 + 273.15 = 298.15 K
- T2 = 100 + 273.15 = 373.15 K
To find V2:
V2 = (V1 * T2)/T1 = (2.5 L * 373.15 K)/298.15 K ≈ 3.14 L
Key Points to Remember

- Kelvin Temperature: All temperatures must be converted to Kelvin scale to use Charles’ Law.
- Pressure Constancy: The law assumes constant pressure; any change in volume is due to temperature change.
- Inverse Relationship: If temperature decreases, volume also decreases, and vice versa.
- Proportional Change: The ratio of volume to temperature remains constant at any point during the process.
⚠️ Note: Never forget to convert temperatures to Kelvin when solving Charles' Law problems to ensure accurate calculations.
In summary, Charles' Law provides a straightforward way to understand the relationship between the temperature and volume of gases. It's instrumental in various scientific fields, from chemistry labs to everyday phenomena like the behavior of balloons. The worksheets and examples provided here aim to deepen your understanding and make this scientific principle more accessible. By applying this law, you not only gain insight into how gases behave under temperature changes but also enhance your problem-solving skills in science.
What happens if pressure changes while applying Charles’ Law?

+
Charles’ Law assumes constant pressure. If pressure changes, you’ll need to use the Combined Gas Law to account for all variables.
How does Charles’ Law apply in everyday life?

+
Examples include the expansion and contraction of helium balloons in different temperatures, the rising of hot air balloons, and the design of certain temperature-regulating materials.
Why is it important to use Kelvin in Charles’ Law calculations?

+
Kelvin is the absolute temperature scale where 0 K is absolute zero. Using Kelvin ensures that the temperature ratio is always positive, which reflects the physical reality that gases never contract below zero volume.