Arithmetic Sequence Worksheet With Answers: Master Number Patterns

Arithmetic sequences are fundamental in understanding mathematical patterns, often forming the backbone of algebra, calculus, and more advanced mathematical disciplines. If you're looking to master arithmetic sequences, a dedicated worksheet can transform your learning experience, offering both practical application and theoretical understanding. This post will guide you through everything from basic arithmetic sequence properties to creating your own worksheet with answers, ensuring you grasp this essential mathematical concept fully.
Understanding Arithmetic Sequences
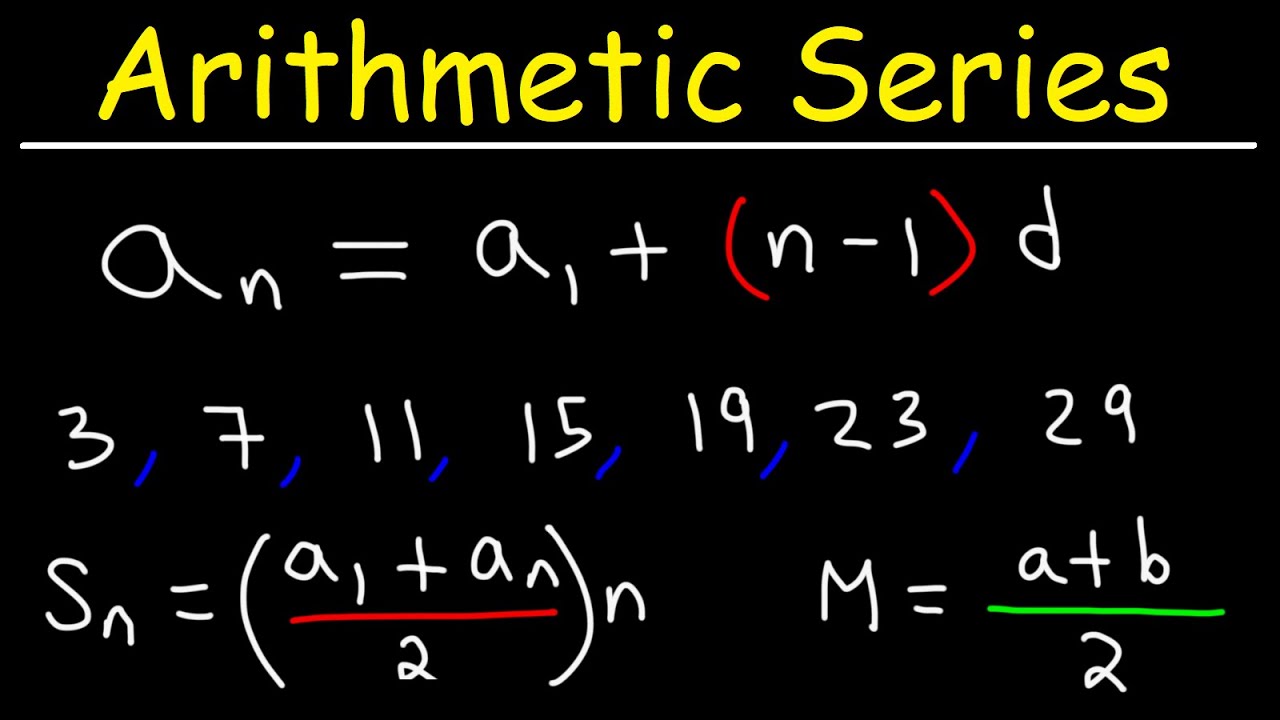
Definition: An arithmetic sequence is a list of numbers where the difference between consecutive terms is constant. This common difference is denoted as d.
Formula: The general term, or the nth term, of an arithmetic sequence can be found using:
\[ a_n = a_1 + (n-1)d \]- a1 - The first term of the sequence.
- d - The common difference.
- n - The term number in the sequence.
Sum Formula: To find the sum of the first n terms:
\[ S_n = \frac{n}{2} \times (a_1 + a_n) \]Creating Your Arithmetic Sequence Worksheet

To construct an effective worksheet for mastering arithmetic sequences, consider the following steps:
Step 1: Setting Objectives

Identify what you want students to learn:
- Identifying common differences.
- Calculating terms in the sequence.
- Determining the sum of sequences.
- Finding missing terms or the common difference from a given set of terms.
Step 2: Designing Problem Sets

| Problem Type | Example | Answers |
|---|---|---|
| Identify d | Given: 2, 5, 8, 11, … | 3 |
| Find the 5th term | Sequence: 7, 14, 21, 28, ... | 35 |
| Sum of first 10 terms | a1 = 3, d = 5 | 145 |
| Missing term | _, 12, 18, 24, … | 6 |

🖊️ Note: Ensure variety in problem types to cover all aspects of arithmetic sequences, making your worksheet comprehensive.
Step 3: Providing Examples

Offering worked examples alongside problems aids understanding:
- Calculate the 6th term of the sequence 2, 4, 6, 8, …
Solution: a6 = 2 + (6-1)2 = 12
Step 4: Incremental Difficulty

Start with simple sequences and increase complexity by introducing negative terms, fractional differences, or higher term calculations.
Step 5: Answers Section

Include a section for answers at the end of the worksheet. This provides immediate feedback, essential for learning:
- Problem: Identify the common difference in the sequence 5, 8, 11, 14.
Answer: 3
Effective Use of Worksheets

Using arithmetic sequence worksheets effectively involves:
- Practice: Regular and focused practice to solidify understanding.
- Feedback: Immediate feedback from the answers section or from a teacher.
- Application: Real-world applications or problems to show the relevance of arithmetic sequences.
📚 Note: Encourage students to check their work with the provided answers to foster self-assessment and correction.
Final Thoughts

In mastering arithmetic sequences, a well-designed worksheet acts as a roadmap, guiding learners from basic understanding to advanced problem-solving skills. Through structured practice, students not only comprehend the sequence properties but also learn to apply these principles to new situations, enhancing their mathematical intuition. With such tools, arithmetic sequences become more than just numbers—they are patterns that reveal the underlying structure of our numerical world.
What is the significance of the common difference in an arithmetic sequence?

+
The common difference, d, is the key factor that differentiates each term in an arithmetic sequence from the preceding one. It allows for predicting future terms and understanding the pattern, making sequences predictable and manageable.
Can arithmetic sequences have a common difference of zero?

+
Arithmetic sequences inherently require a common difference that is not zero because, by definition, an arithmetic sequence needs to show a pattern of consistent change between terms. If d is zero, the sequence would simply be a constant sequence, not arithmetic.
How do you know when a sequence is arithmetic?

+
A sequence is arithmetic if the difference between any two consecutive terms remains constant. This common difference can be positive, negative, or even a fraction but must be the same for the entire sequence.
Is there any practical application of arithmetic sequences in real life?

+
Arithmetic sequences find applications in finance for interest rate calculations, in physics for constant acceleration problems, in computer science for sorting algorithms, and in various scheduling and time-based problems.



