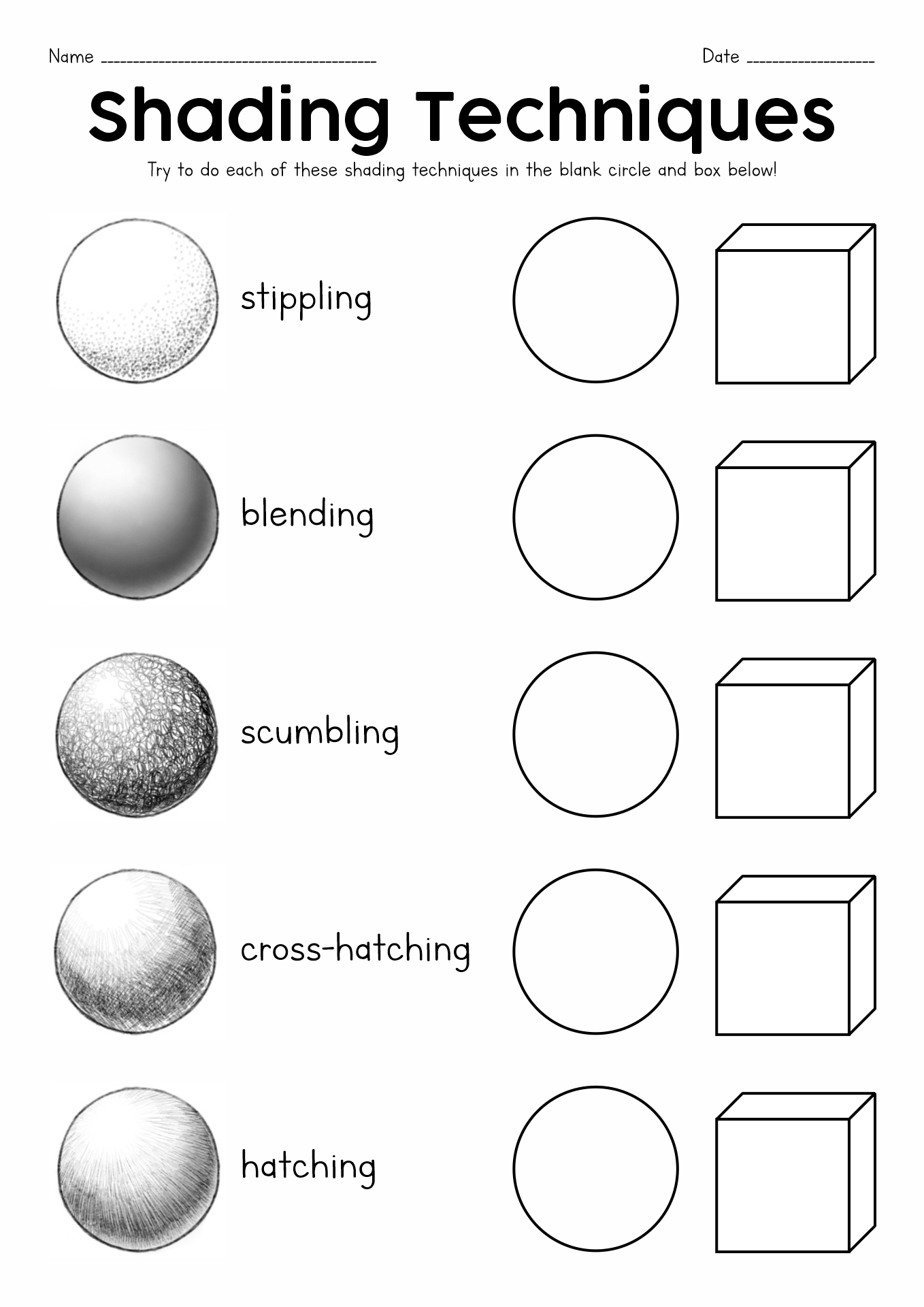
Master the Basics: Area of Square & Rectangle Worksheet

The journey of learning mathematics is often filled with fundamental concepts that build the foundation for more complex calculations and understanding. In this educational journey, understanding area calculations for basic shapes like squares and rectangles is paramount. Not only do these concepts appear frequently in everyday life, but they are also critical for higher-level math and various fields like architecture, engineering, and design. This blog post is tailored to provide you with an in-depth guide on calculating the area of squares and rectangles, complete with practical examples, helpful tips, and an interactive worksheet to cement your learning.
Understanding Area: A Mathematical Overview

Area is a measurement of two-dimensional space, essentially quantifying the size of a flat surface. Before diving into specific shapes, it’s crucial to grasp:
- The definition of area.
- Units of measurement like square centimeters (cm²), square meters (m²), square feet (ft²), and square inches (in²).
📌 Note: Remember, area is always calculated in square units.
Area of a Square

A square, one of the simplest geometric shapes, has all four sides of equal length. The formula to calculate its area is straightforward:
Area of a Square = Side × Side

Here’s how you can apply this formula:
- If a square has sides of 5 meters, the area will be:
5 × 5 = 25 square meters.
🔎 Note: The side length in the formula is written twice, often expressed as Side².
Area of a Rectangle
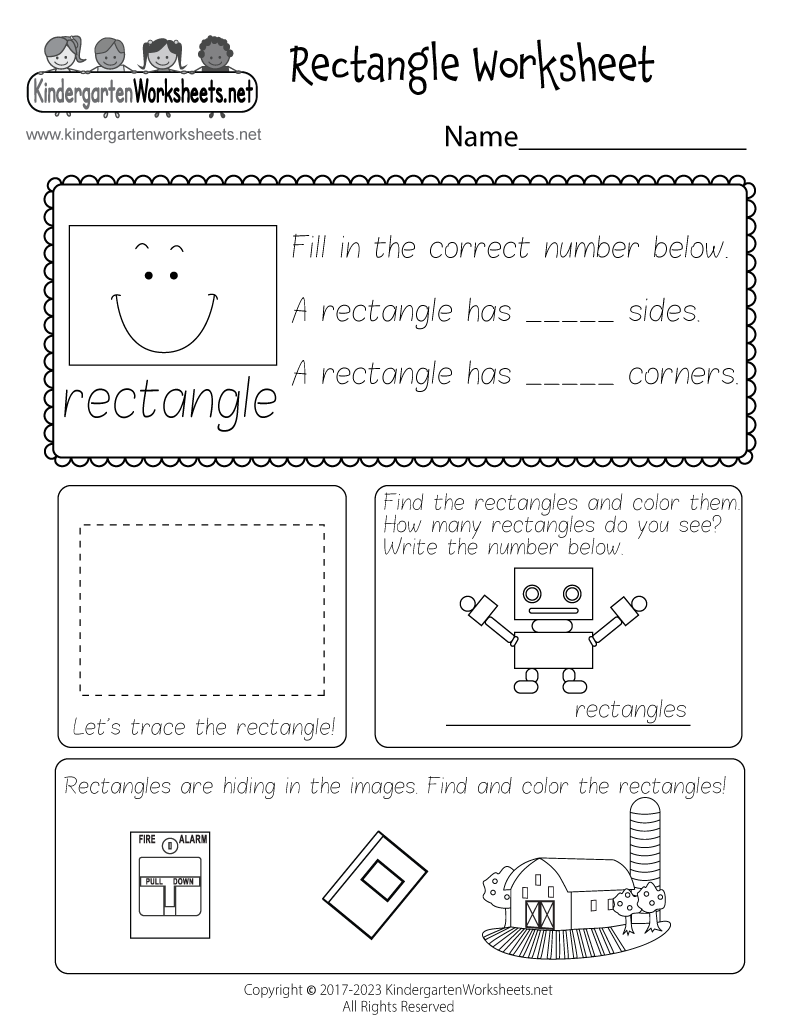
Rectangles have two pairs of equal sides, where adjacent sides are different in length. The formula for finding the area of a rectangle is:
Area of a Rectangle = Length × Width

Here are some steps to find the area:
- Measure the length of the rectangle.
- Measure the width of the rectangle.
- Multiply these two measurements together:
- For example, if a rectangle has a length of 8 inches and a width of 4 inches:
8 × 4 = 32 square inches.
🌟 Note: Unlike squares, rectangles have two distinct measurements for sides.
Practical Worksheet for Area Calculation

To make the learning process more interactive, let’s dive into a practical worksheet. This will help you practice what you’ve learned about calculating the area of squares and rectangles.
| Shape | Dimensions | Area |
|---|---|---|
| Square | Side: 3 cm | |
| Rectangle | Length: 7 cm, Width: 5 cm | |
| Square | Side: 6 inches | |
| Rectangle | Length: 12 feet, Width: 9 feet |

💡 Note: Fill in the Area column with your calculations for each shape.
Advanced Tips for Area Calculation

Here are some advanced tips to enhance your understanding and application of area calculations:
- Convert Units: Sometimes, you might need to convert from one unit of measurement to another. Remember, conversion must be consistent across all measurements.
- Use Geometry Properties: For complex shapes, break them down into simpler forms or use geometric properties like the Pythagorean theorem.
- Accuracy Matters: Ensure your measurements are precise. A small error in length or width can significantly affect the area.
As we wrap up our exploration into calculating the area of squares and rectangles, we reflect on the fundamental yet versatile nature of these shapes. Not only do they serve as basic building blocks in geometry, but their principles are also deeply ingrained in practical applications from determining the amount of paint needed for a wall to optimizing land use in urban planning. By mastering these calculations, you're not only enhancing your mathematical skills but also equipping yourself with the tools to tackle real-world problems with confidence. This foundational knowledge sets the stage for more complex geometric explorations and mathematical endeavors, ensuring you have the skills needed to navigate the world of shapes and spaces with precision.
How can I calculate the area if the measurements are in different units?

+
First, convert all measurements to the same unit before calculating the area. For instance, if one side is given in feet and the other in inches, convert either all to inches or all to feet. Then apply the area formula.
What’s the difference between area and perimeter?

+
Area measures the space inside a shape (2D), while perimeter measures the total length of its outer edges (1D).
Can I find the area of irregular shapes?

+
Yes, by breaking the shape into simpler, measurable shapes like squares, rectangles, or triangles, or by using techniques like the grid method for estimation.