Literal Equations Worksheet Answers: Your Ultimate Guide

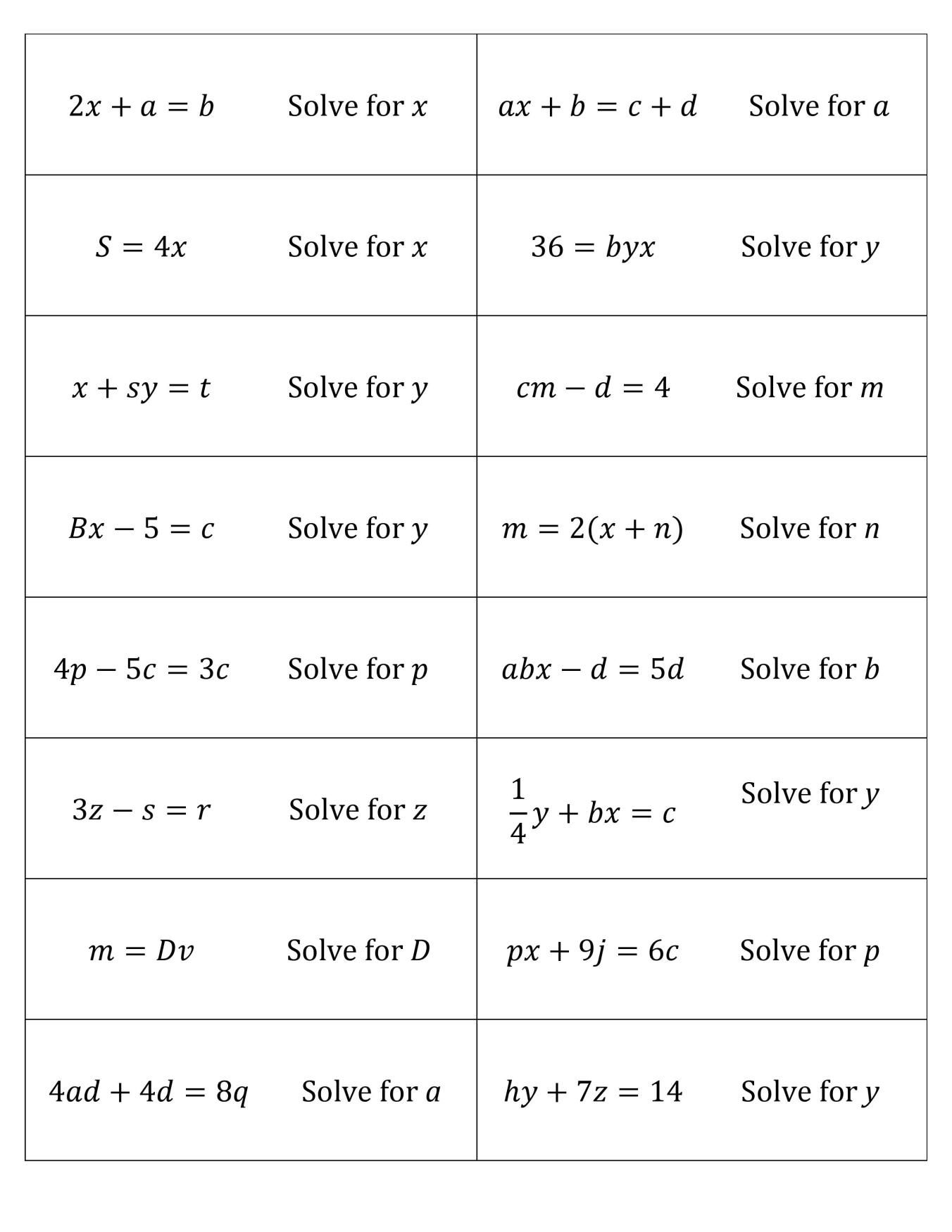
Literal equations, also known as formulas, are mathematical expressions where variables represent values in an equation. Understanding how to solve and manipulate these equations is crucial for students learning algebra and beyond. This comprehensive guide will delve into the process of solving literal equations, provide practical examples, and offer insights on typical mistakes and how to avoid them.

The Basics of Literal Equations

Literal equations are essentially formulas where letters or symbols replace numbers. Common examples include:

• Distance formula: d = rt
• Area of a rectangle: A = lw
• The formula for circumference: C = 2πr

When solving literal equations, the goal is often to isolate one variable in terms of the others. Here’s how you can start:

• Identify the variable you wish to solve for.
• Follow the basic algebraic principles of:
• Adding or subtracting terms to isolate the variable
• Multiplying or dividing both sides by constants or variables
• Ensure the final equation is simplified, with the solved variable on one side.

Solving Literal Equations: Step-by-Step Examples

Let’s walk through some typical examples of solving literal equations:

Example 1: Solving for d in d = rt

Here’s how to solve for distance:

1. Original equation: d = rt
2. If solving for r, divide both sides by t: r = d
3. Simplify: r = d/t

🔍 Note: When dividing by a variable, remember that you are assuming the variable is not zero, otherwise, division by zero would occur.

Example 2: Isolating v in F = ma

Consider Newton’s Second Law where force equals mass times acceleration:

1. Original equation: F = ma
2. To solve for m, divide both sides by a: m = F/a

The resulting equation simplifies to m = F/a, where mass is isolated.

Example 3: Rearranging A = πr²

Here’s how to solve for r:

1. Original equation: A = πr²
2. Divide both sides by π: A/π = r²
3. Take the square root of both sides: r = √(A/π)

The equation can now be used to find the radius given the area of a circle.

Common Mistakes and How to Avoid Them

Here are some common pitfalls when dealing with literal equations:

• Failing to Undo All Operations: Students often forget to perform all the necessary algebraic steps. For example, not squaring both sides when dealing with squared variables.
• Division by Zero: Be cautious when dividing both sides of an equation by a variable or an expression that could be zero.
• Forgetting to Simplify: After isolating the variable, always simplify the expression to make it as straightforward as possible.

Advanced Techniques and Tips

Here are some tips for tackling more complex literal equations:

• Cross-Multiplication: Use cross-multiplication when solving equations with fractions to avoid complex operations.
• Combining Like Terms: If there are variables on both sides of the equation, combine like terms to streamline the process.
• Using Completing the Square: For quadratic equations or those with quadratic terms, completing the square can be a helpful approach.

⚠️ Note: Always verify your solution by substituting back into the original equation to ensure the variables were manipulated correctly.

In wrapping up, solving literal equations is an essential skill for algebra students. It involves a systematic approach to isolating variables while ensuring no mathematical principles are violated. This guide has provided you with a solid foundation to tackle these equations, from basic principles to advanced techniques. Remember, practice and persistence are key to mastering these algebraic concepts.

Why do we need to isolate one variable in a literal equation?

+

Isolating one variable allows us to understand the relationship between the variables more clearly and to solve for the value of that variable given other values.

Can you always solve a literal equation for any variable?

+

Not always. Sometimes, certain variables might be dependent on others in ways that make solving for them impossible without additional information.

What should I do if I get stuck while solving a literal equation?

+

Review each step taken, check for arithmetic errors, and consider alternative methods like substituting known values or using algebraic identities to simplify the equation.