5 Essential Linear Functions Worksheets for Algebra Practice
Mastering linear functions is fundamental to understanding algebra. These mathematical expressions allow us to model real-world phenomena, from simple motion problems to more complex economic models. If you're looking to improve your algebra skills or help others learn, utilizing the right practice worksheets can make all the difference. Here, we delve into five essential linear functions worksheets designed to aid in effective algebra practice.
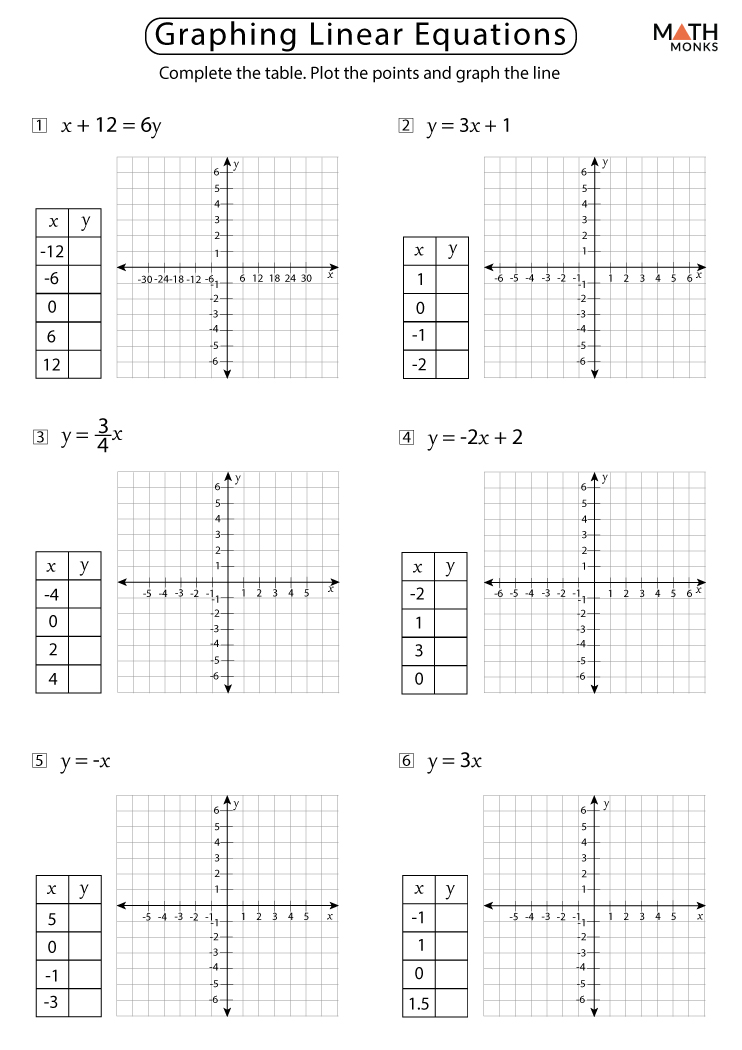
1. Graphing Linear Functions

Graphing is one of the most visual and intuitive ways to understand linear functions. This worksheet focuses on:
- Plotting points on a coordinate plane
- Finding the slope
- Determining the y-intercept
- Using the slope-intercept form of a line: y = mx + b
| Exercise | Description |
|---|---|
| Graphing From Slope and Y-Intercept | Students are given the slope and y-intercept and must graph the line. |
| From Slope and a Point | Given a point and the slope, students graph the line through the point with the specified slope. |
| Identifying Line Equations | Students must determine the equation of the line when given a graph. |

📊 Note: Ensure students have access to graph paper or use a digital graphing tool for precision.
2. Standard Form and Slope-Intercept Form

This worksheet helps students transition between the standard form (Ax + By = C) and the slope-intercept form (y = mx + b):
- Converting from standard form to slope-intercept
- Converting from slope-intercept to standard
- Applying real-life problems to both forms
📌 Note: Highlighting the importance of isolating y in slope-intercept form can aid in understanding.
3. Point-Slope Form

The point-slope form, y - y1 = m(x - x1), is another crucial linear function representation. This worksheet includes:
- Converting point-slope form to slope-intercept form
- Determining line equations using two points
- Finding the equation of a line when given a point and slope
4. Slope and Rate of Change

Understanding slope as the rate of change in various contexts is essential. This worksheet involves:
- Relating slope to real-world scenarios
- Calculating the slope given two points or a graph
- Comparing slopes to analyze different rates of change
📈 Note: Encourage students to think about slope in terms of increase/decrease per unit, making the concept more tangible.
5. Systems of Linear Equations

Combining linear functions through systems of equations helps students:
- Solve systems using substitution
- Use the elimination method
- Interpret the solutions graphically
Each worksheet provides ample practice in manipulating, solving, and understanding linear functions in various forms, enabling students to see the flexibility and utility of these algebraic tools.
Throughout the learning process, it’s worth noting that:
- Mastering linear functions is a stepping stone to higher-level math.
- Application to real-world problems deepens understanding.
- Consistent practice with these worksheets can lead to better algebraic proficiency.
To conclude, these five worksheets are not just exercises but a structured path to algebra mastery. By engaging with these problems, students can develop a comprehensive understanding of linear functions, which forms the backbone of mathematical modeling in various fields. This journey from graphing basic lines to solving systems of equations prepares students for complex algebraic challenges, ensuring they have the foundational skills to tackle mathematics with confidence.
Why are linear functions important in algebra?

+
Linear functions serve as a fundamental building block in algebra. They model numerous real-world phenomena, from calculating growth rates to understanding linear motion, and provide the basis for solving more complex equations and understanding higher levels of math.
How can students practice different forms of linear functions?
+
By working through exercises like converting between slope-intercept and standard form, or using point-slope form, students can gain a deeper understanding. Worksheets tailored to these conversions, graphing, and solving systems of equations are particularly effective.
What’s the best way to understand the concept of slope?

+
Slope is best understood through real-world scenarios where change per unit is measured. Practicing with graphs, calculating rise over run, and interpreting slope as the rate of change in different contexts can solidify understanding.