5 Tips for Mastering Triangle Angles Worksheet

Understanding geometry, particularly triangle angles, is essential in various fields such as architecture, engineering, and even art. Triangles are fundamental shapes in many mathematical and practical applications, making the grasp of their properties, especially their angles, crucial. One of the best ways to get a deep understanding of triangle angles is by practicing with worksheets. Here are five effective tips to help you master your triangle angles worksheet proficiency.
1. Understand the Basic Principles of Triangle Angles

- The sum of the internal angles of any triangle is always 180°.
- Equilateral triangles have all angles equal to 60°.
- Isosceles triangles have two equal sides and therefore two equal angles.
- Scalene triangles have all sides and angles different.
📏 Note: Knowing these foundational facts helps in solving triangle angle problems quickly.
2. Practice Recognizing Triangle Types

- Right triangles have one angle that measures exactly 90°.
- Obtuse triangles have one angle that measures more than 90°.
- Acute triangles have all angles less than 90°.
🔺 Note: Identifying the type of triangle can often provide clues on how to approach angle calculations.
3. Use Trigonometry When Necessary

While triangle angles worksheets might not explicitly ask for trigonometry, having a basic understanding can be beneficial:
- Sine, Cosine, and Tangent functions can help in calculating angles or sides when you have enough information.
- Remember the SOHCAHTOA mnemonic to recall these functions.
🔢 Note: Trigonometry is particularly useful for right triangles but can also apply to others with careful application.
4. Apply Theorems and Properties
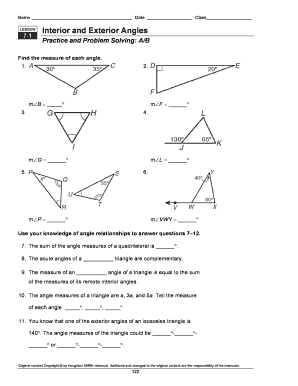
- Angle Sum Property: The sum of the interior angles is always 180°.
- Exterior Angle Theorem: An exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles.
- Isosceles Triangle Theorem: Angles opposite the equal sides are equal.
- Triangle Inequality Theorem: The sum of any two sides of a triangle is greater than the third side.
⚖️ Note: Applying these theorems can simplify problem-solving, especially when working with incomplete information.
5. Regularly Review and Practice

Consistent practice is key to mastering triangle angles:
- Set aside time each day to work through different types of triangle angle problems.
- Use online resources, textbooks, or dedicated geometry workbooks for additional practice.
- Time yourself solving problems to improve speed and efficiency.
⏰ Note: Regular review reinforces concepts, making them second nature for complex problem-solving.
In summary, mastering triangle angles worksheets involves understanding basic principles, recognizing triangle types, applying trigonometry where necessary, using theorems effectively, and maintaining consistent practice. By implementing these tips, you'll not only improve your worksheet performance but also your overall geometry skills. Whether for academic purposes or practical application in fields requiring geometric knowledge, these tips will help you navigate and excel in the world of triangle angles.
How can I quickly tell if a triangle is equilateral?

+
You can tell a triangle is equilateral if all its angles are equal to 60° or if all its sides are of the same length.
What if the angles aren’t given in a worksheet?
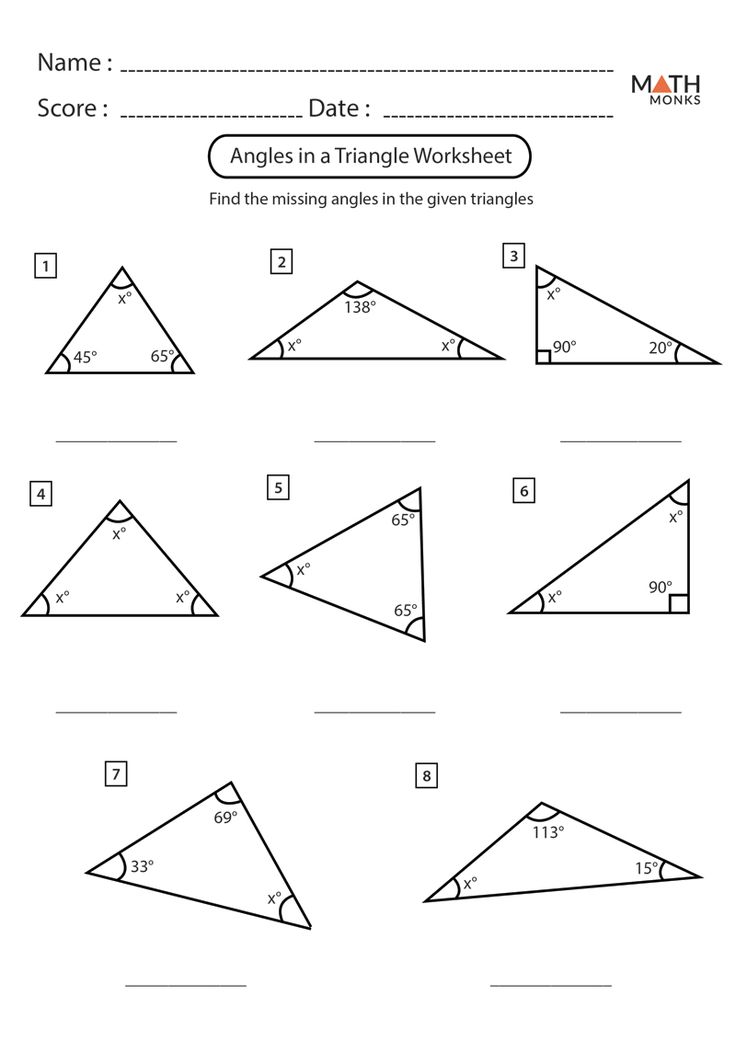
+
If angles aren’t provided, use the information about sides or look for patterns that suggest specific triangle types. Use theorems and properties to deduce angles.
Is trigonometry necessary for all triangle angle problems?

+
While trigonometry isn’t always necessary, it can be extremely helpful in calculating unknown angles or sides, particularly in right triangles.
What’s the best way to remember all these theorems?

+
Regular practice and application of theorems help in retention. Create flashcards or mnemonic devices to aid memory. Also, understand the why behind each theorem for deeper comprehension.



