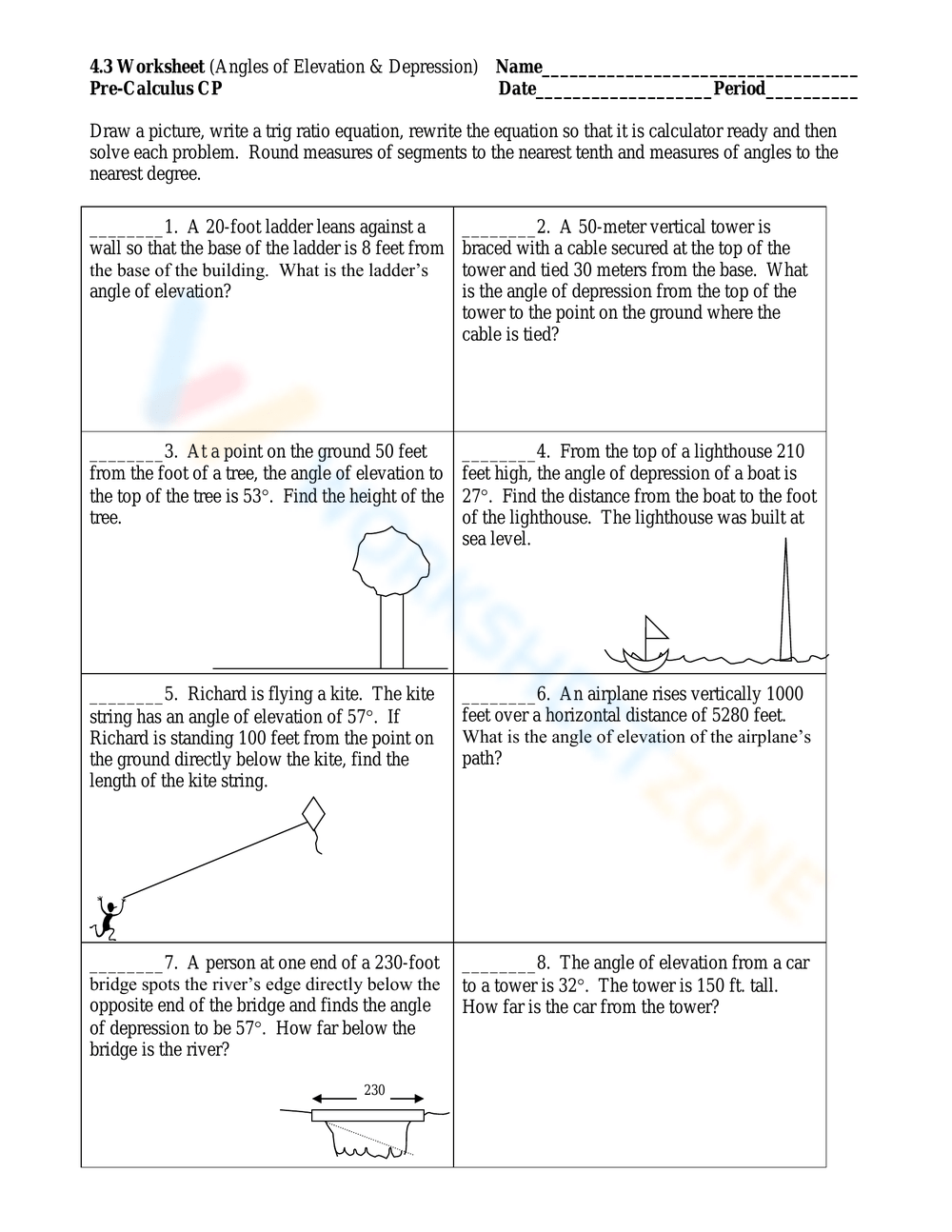
Angle of Elevation and Depression Worksheet Solutions

In the field of geometry and trigonometry, understanding the concepts of angle of elevation and angle of depression is pivotal. These angles are used to solve problems involving indirect measurement, often in fields like surveying, navigation, and even everyday life when looking at tall buildings or flying aircraft. This comprehensive guide will delve into detailed explanations, practical applications, and step-by-step solutions to various problems related to these angles. By the end, readers will not only understand the theoretical aspects but also be equipped to solve real-world problems involving these angles.
Understanding the Basics

Before diving into complex problems, let’s ensure we grasp the fundamental concepts:
- Angle of Elevation: This is the angle above the horizontal line that an observer would need to look up in order to see an object above them. For instance, if you look up at an airplane flying overhead, the angle your line of sight makes with the ground is the angle of elevation.
- Angle of Depression: Conversely, the angle of depression is the angle below the horizontal line that an observer needs to look down to see an object below them. For example, if you’re at the top of a building looking down at the street, the angle between your downward gaze and the horizontal is the angle of depression.
Practical Applications

The applications of these angles are numerous:
- Surveying: Surveyors use these angles to determine the height of landmarks, distances between points, and the slope of terrain.
- Navigation: In navigation, pilots and sailors use these angles to locate themselves relative to known points, calculate bearings, or estimate distances.
- Architecture and Construction: Builders might need to determine the height of a building or the angle at which it needs to be stabilized.
- Ecology: Scientists might use these angles to measure the height of trees or the distance to water bodies without disturbing the environment.
Solving Problems with Angles of Elevation and Depression

Let’s work through some examples to solidify our understanding:
Example 1: Height of a Building

Suppose an observer standing 50 meters from the base of a building looks up at an angle of elevation of 30 degrees. How high is the building?
Here’s how we solve this:
- Identify the knowns: Distance from the building = 50 m, Angle of elevation = 30 degrees.
- Set up the trigonometric relation: Since we have the base (adjacent) and the angle, we can use tangent: [ \tan(\theta) = \frac{\text{opposite}}{\text{adjacent}} ] [ \tan(30^\circ) = \frac{h}{50} ] where ( h ) is the height we need to find.
- Calculate: [ h = 50 \times \tan(30^\circ) \approx 50 \times 0.577 = 28.85 \text{ meters} ]
🎨 Note: In real-world scenarios, factors like the height of the observer’s eyes from the ground can affect calculations.
Example 2: Distance to an Airport

Imagine an airplane flying at an altitude of 3000 meters. From the ground, the angle of elevation to the plane is 45 degrees. How far is the airport from the observer?
- Identify the knowns: Altitude of plane = 3000 m, Angle of elevation = 45 degrees.
- Set up the relation: Here, tangent would be useful: [ \tan(45^\circ) = 1 = \frac{3000}{d} ] where ( d ) is the horizontal distance.
- Calculate: [ d = \frac{3000}{\tan(45^\circ)} = 3000 \text{ meters} ]
Table of Example Problems and Solutions

| Problem | Solution |
|---|---|
| Find the height of a 60-meter tall tree from a point 20 meters away (angle of elevation = 75°). | Using ( \sin(75^\circ) = \frac{60}{h} ), solving for ( h ). |
| A ship at sea is 10 km from a lighthouse. The lighthouse keeper measures the angle of depression to the ship as 20°. | Using ( \tan(20^\circ) = \frac{h}{10} ), solve for ( h ) to find lighthouse height. |

Wrapping Up

Understanding and applying angles of elevation and depression enriches our ability to analyze and interpret spatial relationships in our environment. Whether it’s to estimate the height of a skyscraper or the distance to a destination, these concepts empower us with practical skills for everyday and professional challenges. By mastering the use of trigonometry in these situations, we not only enhance our problem-solving abilities but also deepen our appreciation for how mathematics underpins the world around us.
What is the relationship between angle of elevation and depression?

+
The angle of elevation from one point is the angle of depression from the opposite point, assuming the horizontal lines are parallel and the points are vertically aligned.
How do I know when to use the angle of elevation or depression?

+
Use angle of elevation when looking up at something from a lower position, and use angle of depression when looking down from a higher position.
Are there practical tools to measure these angles accurately?
+
Yes, tools like clinometers, theodolites, and sextants are commonly used for precise measurements in fields like surveying and navigation.
Can these angles be used for navigation?

+
Absolutely, both pilots and sailors use angles of elevation and depression for dead reckoning, estimating distances, and calculating bearings.