Algebraic Addition and Subtraction Worksheet Mastery Guide

Mastering algebraic addition and subtraction is a crucial skill for anyone stepping into the world of algebra. It forms the backbone of algebraic manipulation, setting the stage for more advanced mathematical concepts. This guide dives deep into the practice of working with algebra, focusing on the subtleties of adding and subtracting algebraic terms. Here, we'll cover not only the basics but also strategies for mastering these operations, enabling you to tackle even the most challenging algebraic worksheets with confidence.
Understanding the Basics
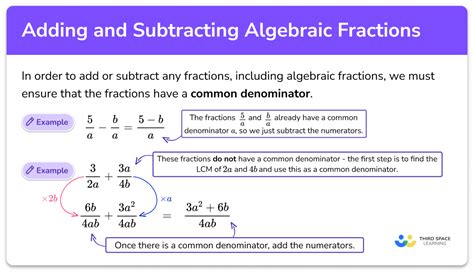
Algebraic expressions involve variables, coefficients, and constants. Addition and subtraction of these expressions require a solid grasp of these elements:
- Variable: A symbol that represents an unknown quantity (e.g., x, y, z).
- Coefficient: The numerical factor of a term that includes a variable (e.g., the “3” in 3x).
- Constant: A term without a variable (e.g., 5 in the expression 3x + 5).
Key Rules for Adding and Subtracting Algebraic Terms

Before diving into complex problems, here are the fundamental rules you should commit to memory:
- Like Terms: Only terms with the same variables raised to the same power can be combined. For example, 3x and 5x can be added to get 8x.
- Distributive Property: When adding or subtracting within parentheses, distribute the sign to every term inside. For instance, - (3x - 4) becomes -3x + 4.
- Change in Sign: When subtracting, every term within the expression being subtracted changes sign.
Techniques for Mastering Algebraic Addition

Here are some strategies to help you excel in adding algebraic expressions:
- Identify and Combine Like Terms: Begin by grouping and combining like terms, which simplifies the problem and minimizes errors.
- Visualize: If possible, sketch or visualize the terms as areas of a rectangle or parts of a diagram, which can help in understanding how terms combine.
- Step-by-Step Approach: Write each step out to avoid missing any sign changes or wrong coefficients combinations.
Subtracting Algebraic Expressions

Subtraction in algebra follows similar rules to addition but with added nuances:
- Subtraction as Addition of the Opposite: Think of subtraction as adding the opposite (negative) of the term you’re subtracting. For example, 5x - 2x = 5x + (-2x) = 3x.
- Change Signs Inside Parentheses: When subtracting an expression in parentheses, change the sign of every term inside it.
- Align Terms: Align terms vertically to avoid missing or misreading signs, especially in longer expressions.
Practice Exercises

The key to mastering algebra is consistent practice. Here are some exercises to hone your skills:
| Problem | Answer |
|---|---|
| 3x + 5 - 2x + 7 | x + 12 |
| (5x - 3) + (2x + 4) | 7x + 1 |
| 8y - (2y - 6) | 6y + 6 |
| 3(a + 2b - 1) - (4a - 2b - 5) | -a + 8b + 2 |

📝 Note: Remember, consistency is key when practicing algebra. Mistakes in one step can cascade into bigger errors later on, so always double-check your work.
Algebraic addition and subtraction are not just about following rules; they're about understanding how numbers and variables interact. By mastering these foundational skills, you're setting yourself up for success in all areas of algebra. Whether you're simplifying complex polynomials or solving equations, the ability to add and subtract algebraic terms confidently is indispensable. Remember to practice regularly, understand the principles deeply, and soon, these operations will become second nature, allowing you to tackle algebraic challenges with ease.
What’s the easiest way to spot like terms?

+
Like terms share the same variables with the same exponents. For instance, 2x and -3x are like terms, but 2x and 3x^2 are not because the exponents differ.
How can I avoid mistakes when subtracting algebraic terms?

+
Focus on changing signs. When subtracting, view it as adding the opposite. Also, align terms vertically if possible to keep track of every sign change.
Why is distributing the sign important in parentheses?

+
Distributing the sign ensures that you’re accounting for every term inside parentheses, preventing errors in your calculations.