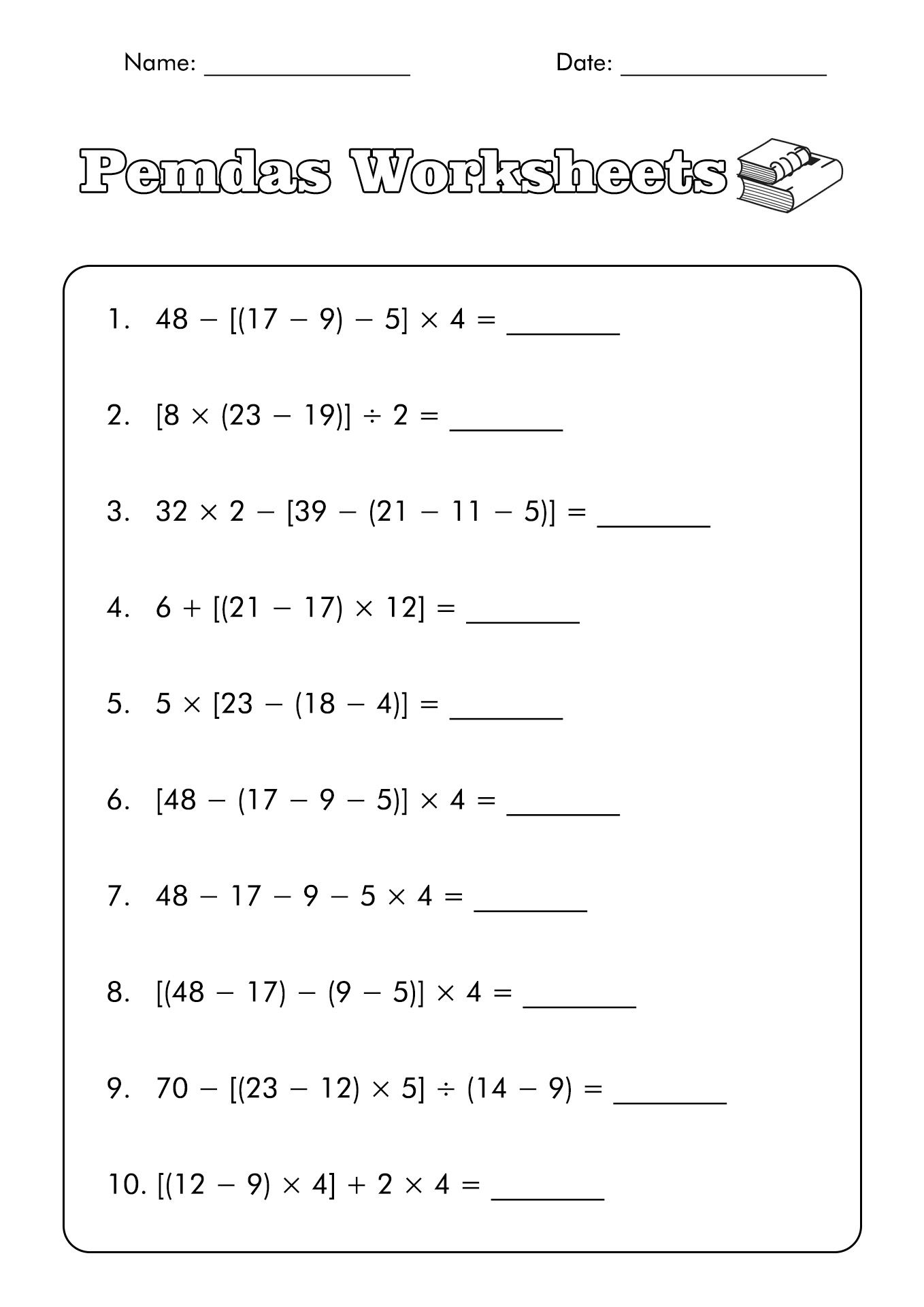
5 Ways to Master Algebra 2 Functions Easily

Algebra 2 functions can often seem daunting with their abstract concepts, but with the right approach, mastering these mathematical tools can become not just manageable, but enjoyable. Here are five strategic ways to navigate through the complexities of algebra 2 functions:
1. Understand the Basics of Functions


Before diving into complex functions, ensure you have a firm grasp of the basics:
- Definition: Understand that a function is a relation where each input has exactly one output.
- Domain and Range: Recognize the set of all possible inputs (domain) and outputs (range) of a function.
- Notation: Familiarize yourself with common notations like f(x) or g(x).
2. Learn the Types of Functions


Algebra 2 introduces a range of function types. Here's a brief overview:
| Type of Function | Description |
|---|---|
| Linear | Functions with the form f(x) = mx + b |
| Quadratic | Parabolas formed by equations like f(x) = ax^2 + bx + c |
| Polynomial | Functions with terms in powers of x, where exponents are non-negative integers. |
| Rational | Fractions where both numerator and denominator are polynomials. |
| Exponential and Logarithmic | Functions involving exponents or logarithms, like f(x) = a^x or f(x) = loga(x). |

💡 Note: Familiarity with these function types not only helps in solving problems but also in understanding real-world applications.
3. Graph Functions Effectively


To truly understand functions, visualize them:
- Plot Points: Start by plotting a few points to get an idea of the function's behavior.
- Understand Key Features: Identify intercepts, asymptotes, and any symmetry.
- Use Graphing Software: Tools like Desmos or Graphing Calculator can help visualize and experiment with functions in real-time.
4. Practice Transformation of Functions


Understanding how to transform basic functions helps in recognizing patterns:
- Vertical and Horizontal Shifts: Learn how adding or subtracting constants inside or outside the function affects its graph.
- Scaling: Understand the effect of multiplying the function by a constant.
- Reflections: Know how to reflect functions over the x or y-axis.
- Combinations: Combine transformations to predict new graphs.
📚 Note: Practice is key here. The more you transform functions, the more intuitive it becomes.
5. Solve Algebra 2 Problems Using Functions
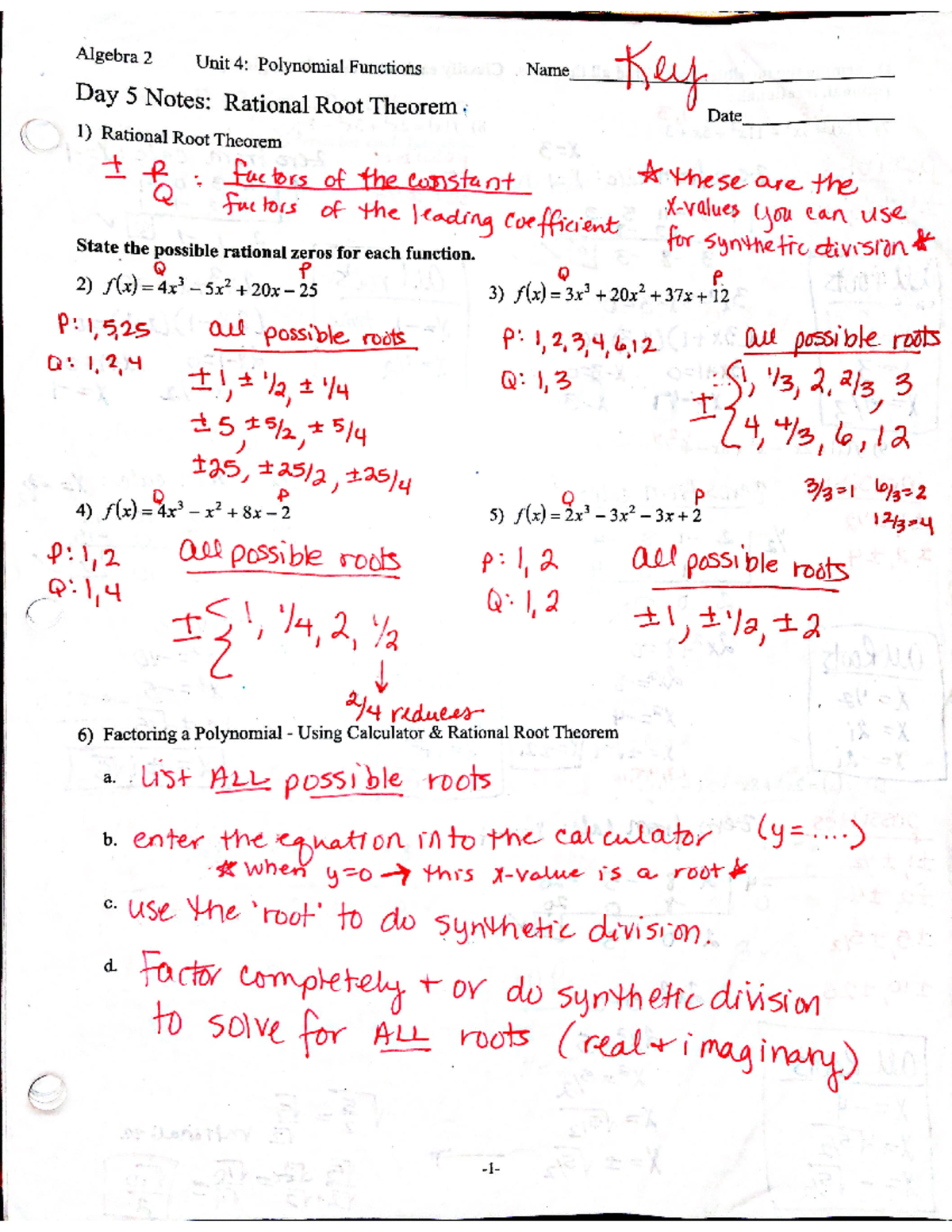

Here are methods to effectively apply your knowledge of functions:
- Modeling: Use functions to model real-world scenarios like growth of populations or decay.
- Solving Equations: Recognize when and how to apply functions to solve algebraic equations.
- Optimization: Use calculus principles to maximize or minimize functions.
- Analyze Data: Apply functions to understand trends, patterns, and make predictions in data sets.
🚀 Note: Remember that functions are not just theoretical; they are tools to solve real problems!
By employing these strategies, you'll find that algebra 2 functions become less intimidating. Understanding the fundamentals, recognizing the different types of functions, visualizing them through graphs, mastering transformations, and applying these concepts to problem-solving will significantly elevate your mathematical skills. Functions are not just abstract entities but are essential tools for analysis and prediction in numerous fields, making them a cornerstone of algebra 2 education.
What are the key differences between linear and quadratic functions?

+
Linear functions have a straight-line graph with a constant slope, while quadratic functions produce a parabola with a U or n-shaped curve, where the rate of change is not constant.
How can graphing software help in learning algebra 2 functions?
+
Graphing software allows students to visualize functions in real-time, making it easier to understand transformations, analyze behavior, and solve equations interactively.
What are some real-world applications of exponential functions?

+
Exponential functions are used in modeling population growth, compound interest calculations, radioactive decay, and even signal processing in engineering.
Can understanding functions in algebra 2 help with calculus?

+
Absolutely! Calculus is built upon the foundations of algebra 2, particularly functions, where concepts like limits, derivatives, and integrals use functions as a starting point.
Why is it important to understand function transformations?

+
Understanding function transformations allows you to predict how changes in equations affect their graphs, enabling efficient problem-solving and graphical analysis in advanced mathematics.