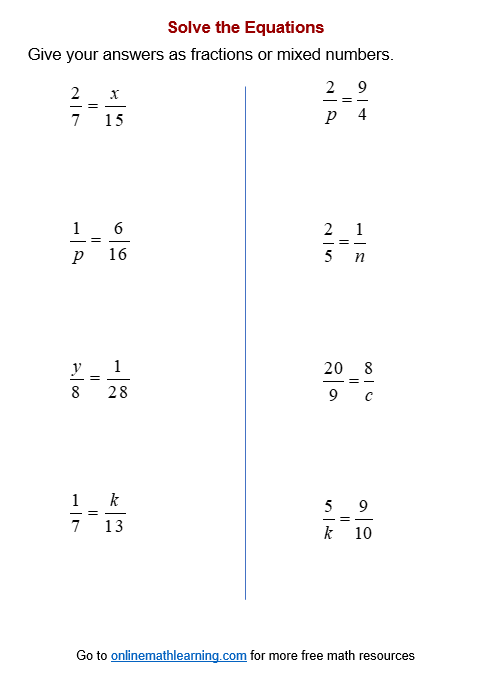
Master Adding Fractions with Unlike Denominators Easily

The process of adding fractions can seem daunting, particularly when the denominators are different. But with the right approach, adding fractions with unlike denominators can become a seamless part of your mathematical toolkit. This article dives deep into mastering this skill, breaking down each step to ensure clarity and understanding. Whether you're a student, teacher, or simply a math enthusiast, these steps will simplify the addition of fractions, making it less of a chore and more of an enjoyable challenge.
Understanding Fractions

Before we delve into adding fractions, it’s essential to grasp the basics:
- Numerator: The top number, which represents the number of parts you have.
- Denominator: The bottom number, indicating the total number of parts the whole is divided into.
When adding fractions, our goal is to make the denominators identical, enabling us to add the numerators directly.
Step-by-Step Guide to Adding Fractions with Unlike Denominators

Here's how to approach this task:
1. Identify the Least Common Denominator (LCD)

The Least Common Denominator or LCD is the smallest number that all denominators can divide into without leaving a remainder. Here’s how to find it:
- List the multiples of each denominator.
- Find the smallest common multiple.
Let’s use an example:

🔎 Note: Finding the LCD can sometimes be simplified by using the largest denominator as a starting point if other denominators are its multiples.
2. Convert Each Fraction to an Equivalent Fraction with the LCD

Once you have the LCD, convert each fraction by multiplying both the numerator and the denominator by the same value to keep the fraction's value unchanged:
Example:
| Original Fraction | LCD | Multiplier | New Fraction |
|---|---|---|---|
| 1/4 | 8 | 2 | 2/8 |
| 3/8 | 8 | 1 | 3/8 |

3. Add the Numerators

With all fractions having the same denominator, you can now add the numerators directly:
[ \frac{2}{8} + \frac{3}{8} = \frac{5}{8} ]
🔍 Note: If you are adding more than two fractions, add the numerators one by one and keep the denominator consistent.
4. Simplify the Fraction if Possible

After adding, check if the result can be simplified. The common factors of both numerator and denominator should be divided out:
Example:
- Original Sum: \frac{5}{8} is already in its simplest form.
5. Convert to a Mixed Number if Necessary

if the resulting fraction is an improper fraction (where the numerator is larger than the denominator), convert it to a mixed number:
[ \frac{10}{4} = 2\frac{2}{4} ]
Then, simplify the fraction part:
[ 2\frac{1}{2} ]
Common Mistakes and How to Avoid Them

Here are some common pitfalls and how to steer clear of them:
- Multiplying Numerators: Never multiply numerators when converting to equivalent fractions for addition.
- Incorrect LCD: Ensure the LCD you're using is the smallest possible common denominator.
- Forgetting to Simplify: Always reduce the final fraction to its simplest form.
Practice Makes Perfect

To master adding fractions, regular practice is key. Use practice problems, online tools, or create your own exercises to sharpen your skills. Here are a few tips to keep your learning on track:
- Work on different levels of complexity, from simple to challenging problems.
- Try to time yourself to increase speed and accuracy.
- Use visual aids like fraction strips or pie charts to understand the concept visually.
In conclusion, adding fractions with unlike denominators is an exercise in patience and logical thinking. By following these steps, understanding common mistakes, and practicing regularly, you'll soon be adding fractions with ease. Remember, every journey begins with a single step, and in the world of mathematics, that step can make all the difference. With this guide, you're well on your way to becoming proficient in this fundamental arithmetic operation.
Why do we need to find a common denominator when adding fractions?

+
Fractions represent parts of a whole, and adding fractions means combining these parts. To do so correctly, they must have the same whole, which is represented by having a common denominator.
Can the Least Common Denominator ever be one of the denominators?

+
Yes, if one denominator is a multiple of the other(s). However, this is not always the case, as the LCD must be the smallest common multiple of all given denominators.
What if the fractions have very large denominators?

+
In real-world scenarios, dealing with fractions with large denominators might involve using calculators or simplifying the process by reducing each fraction first, then finding a new LCD.
Is there a shortcut to finding the LCD?
+
Yes, for small numbers, the prime factorization method or listing multiples can be quick. For larger numbers, you might use the difference of factors approach or mathematical software.