6.1 Exponential Growth/Decay Worksheet Answers: Quick Guide

In the realm of mathematics and science, understanding the concepts of exponential growth and decay is crucial for students, researchers, and anyone interested in how quantities change over time. Exponential models are used to describe a wide range of phenomena, from population growth to radioactive decay. This guide aims to simplify the complexities of exponential functions, providing a comprehensive yet concise overview of the topic through worked examples, explanations, and key notes. Whether you're preparing for an exam or looking to refresh your understanding, let's dive into the essentials of exponential growth and decay.
What is Exponential Growth/Decay?

Exponential Growth refers to an increase in quantity at a constant rate per time period. The hallmark of exponential growth is that the rate of change of the quantity is proportional to its current value. Mathematically, this can be expressed as:
- P(t) = P_0 \cdot e^{rt}
Where:
- P(t) - The amount at time t
- P_0 - The initial amount at time t = 0
- r - The growth rate (which can be positive or negative)
- t - Time
Exponential Decay, conversely, represents a decrease where the rate of decline is proportional to the current value, typically seen in scenarios like depreciation of assets or the decay of radioactive substances. The formula is:
- P(t) = P_0 \cdot e^{-rt}
Worksheet Answers: Quick Guide
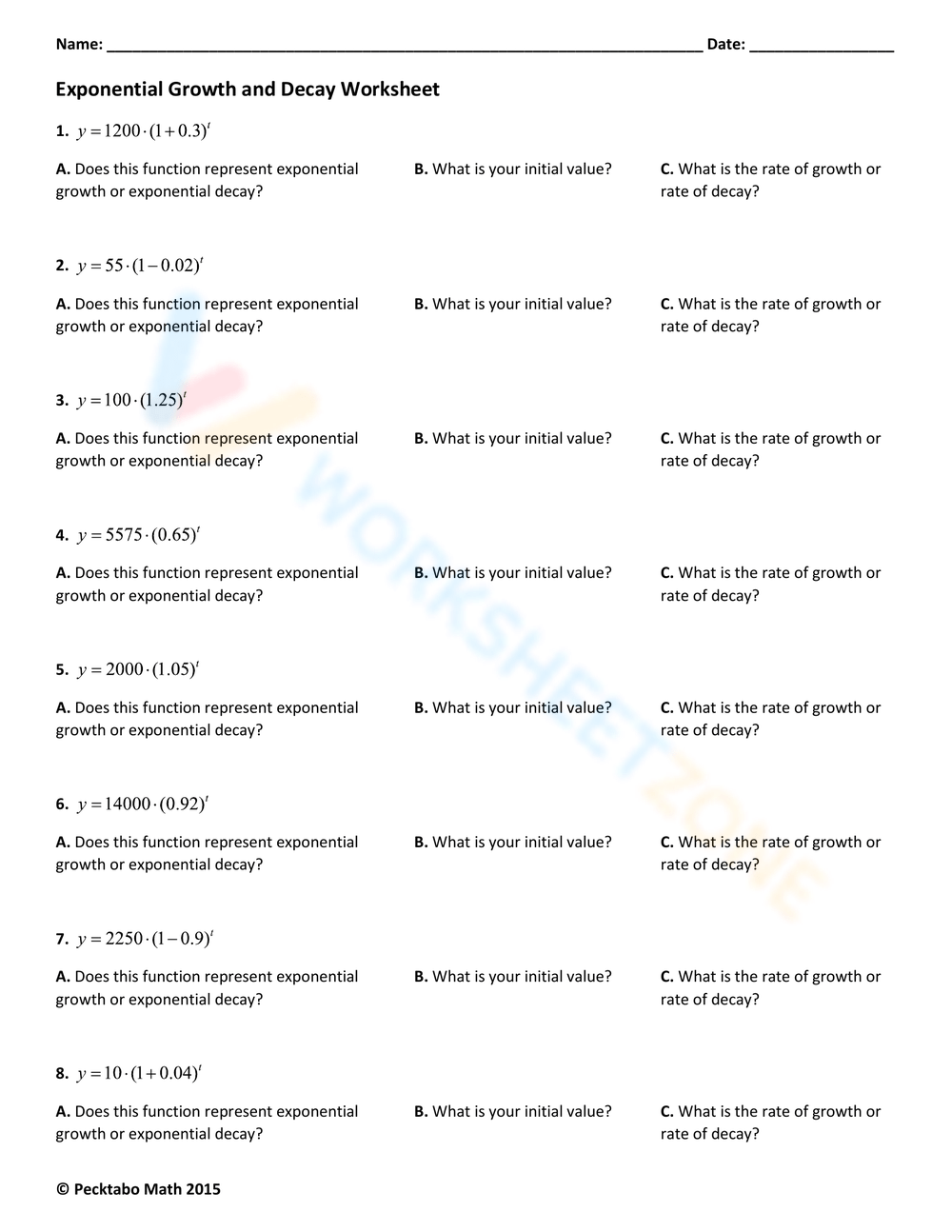
This section provides straightforward answers to common worksheet questions on exponential growth and decay, focusing on both calculation and interpretation:
Example 1: Population Growth

Given a population of 1000 people growing at 3% annually:
- Find the population after 10 years.
Using the formula:
P(t) = 1000 \cdot e^{0.03 \cdot 10}
P(t) \approx 1346.52
📚 Note: Always round to a reasonable precision as per the context or instructions given.
Example 2: Radioactive Decay
A sample initially contains 10 grams of a substance with a half-life of 5 years. What's left after 15 years?
- Find the remaining amount after 15 years.
Here, the decay constant k can be found from the half-life:
\[ k = \frac{\ln(2)}{5} \approx 0.1386 \]
Using the decay formula:
\[P(t) = 10 \cdot e^{-0.1386 \cdot 15} \approx 1.23 \text{ grams}\]
Practical Applications

The concepts of exponential growth and decay aren't just theoretical; they have real-world applications:
- Finance: Calculating compound interest or depreciation.
- Medicine: Understanding drug concentration levels over time.
- Environmental Science: Predicting the spread of invasive species or pollutants.
- Physics: Modelling the decay of radioactive materials.
Key Points for Better Understanding

- Units of Rate: The growth or decay rate (r) should be in terms of time for exponential growth or decay to be meaningful. This could be days, years, or any other unit of time.
- e: Euler's number, approximately 2.71828, is fundamental in these calculations due to its unique properties concerning the exponential function.
- Proportionality: The rate of change is always proportional to the current value, not a fixed amount, which is what makes the function exponential.
Lastly, summarizing the key insights, exponential growth and decay are fundamental concepts that help us predict and understand how various quantities change over time. From finance to physics, these models are ubiquitous. By mastering the formulas and understanding the underlying principles, one can accurately model and predict phenomena. Whether it's calculating population growth or understanding the decay of materials, the power of exponential functions lies in their simplicity and wide applicability.
What’s the difference between exponential growth and exponential decay?

+
The primary difference lies in the rate ‘r’ in the exponential formula. For growth, r is positive, indicating an increase over time. For decay, r is negative, showing a decrease.
Can exponential decay models be used for economic applications?

+
Yes, exponential decay models are widely used in economics, particularly for modelling depreciation of assets or the gradual decline in the value of currency over time.
Why is the number ‘e’ used in exponential functions?

+
Euler’s number ‘e’ arises naturally in mathematical models where growth or decay is continuous. Its derivative is itself, making it unique for exponential calculations.



