Multi-step Equations Worksheet Answers: 5 Essential Tips

In the journey of mastering Algebra, multi-step equations represent a critical milestone, pushing students to apply multiple mathematical operations to isolate the variable and find its value. Here's how you can excel in solving these equations:
1. Understanding the Basics

First, ensure you have a solid grasp of the fundamentals:
- Know the order of operations (PEMDAS: Parentheses, Exponents, Multiplication and Division, Addition and Subtraction).
- Understand the concept of the variable as an unknown quantity.
- Familiarize yourself with the rules for manipulating equations, like the addition, subtraction, multiplication, and division property of equality.
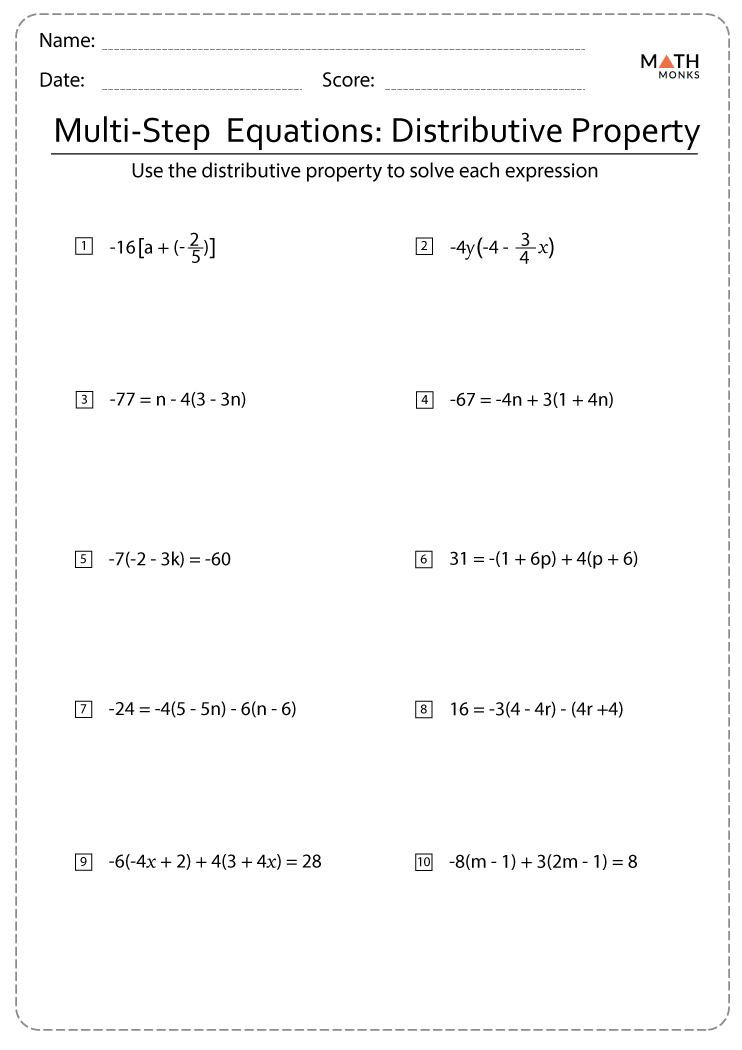
2. Simplify Your Equations
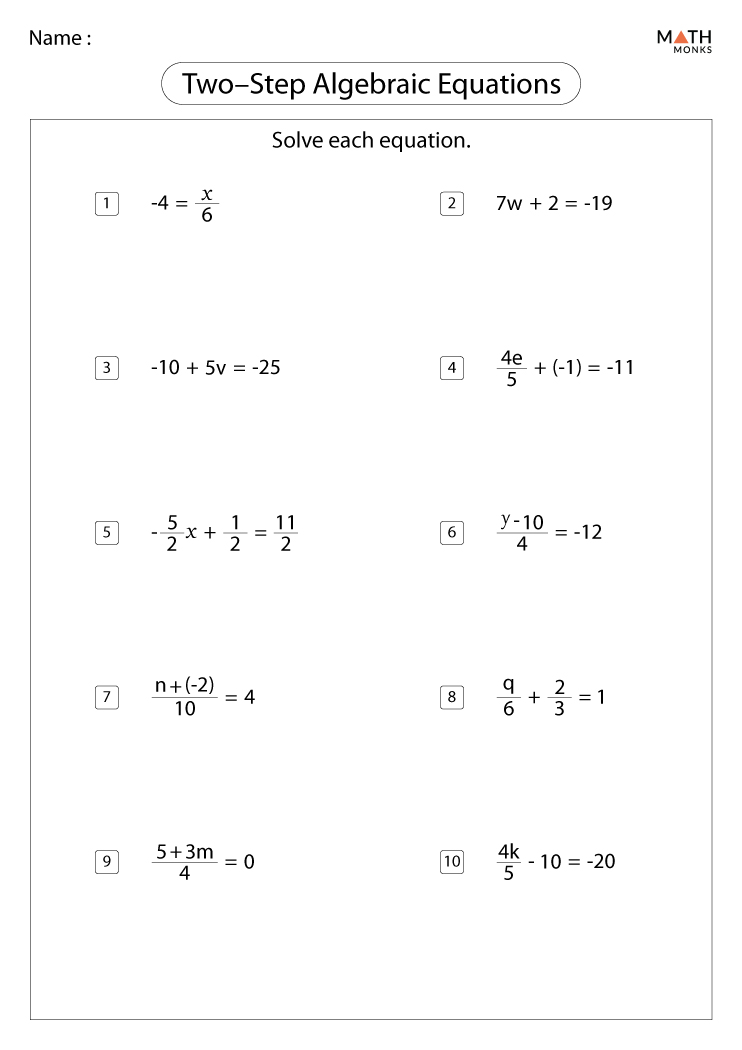
Before diving into solving multi-step equations:
- Eliminate parentheses by distributing coefficients.
- Combine like terms to reduce complexity.
3. The Art of Isolating the Variable

Your goal in solving multi-step equations is to isolate the variable on one side of the equation:
- Begin by moving constants to one side using addition or subtraction.
- Then, use multiplication or division to separate the variable from any coefficients or other variables.
🔍 Note: When you perform an operation on one side of the equation, you must do the same on the other side to keep the equation balanced.
4. Check Your Work

After solving, verification is key:
- Substitute your solution back into the original equation.
- If both sides are equal, you’ve solved it correctly.
- If not, review your steps and ensure you’ve performed each operation correctly.
5. Practice, Practice, Practice

There is no substitute for practicing. Here are some exercises you might encounter:
| Equation | Solution |
|---|---|
| 2(x + 5) + 3x = 25 | x = 5 |
| 5 - 2(x + 3) = 3 | x = 1 |
| 3x + 4(2x - 1) = 20 | x = 1 |

Engaging with these examples will enhance your fluency in handling multi-step equations.
By following these tips, you'll develop a robust approach to tackling multi-step equations. Remember, the journey from understanding basic operations to solving complex algebraic equations is a path of steady improvement. Each equation you solve sharpens your problem-solving skills, providing a deeper understanding of algebra's beautiful and logical system.
What if the equation has fractions?

+
If an equation involves fractions, multiply all terms by the least common multiple (LCM) of the denominators to eliminate them, making the equation easier to solve.
How do you deal with variables on both sides of the equation?

+
Add or subtract the same variable term to both sides to move all variable terms to one side, then solve as usual.
What should you do if you find no solution?

+
This might indicate a mistake or that the equation has no solution (often represented as ∅ or no solution). Double-check your work, or consider that the equation could be an identity with infinite solutions.
How do you ensure accuracy when solving equations?
+
Always check your work by substituting your solution back into the original equation to verify equality on both sides.



