Quadratic Equations Worksheet: Vertex and Point Examples

Understanding Quadratic Equations through Vertex and Point Examples
Quadratic equations, often found in high school algebra and beyond, are not just mathematical constructs; they're tools for describing real-world phenomena, from the flight of a ball to the path of a satellite. This blog post delves into the fascinating world of quadratic equations, with a focus on understanding them through their vertices and points.
What is a Quadratic Equation?

A quadratic equation is a second-degree polynomial equation of the form:
\[ ax^2 + bx + c = 0 \]
Here, a, b, and c are constants, and a ≠ 0. The solution to this equation is found by solving for x using methods like factoring, completing the square, or the quadratic formula.
Structure and Components

- Vertex: The vertex is the highest or lowest point on the parabola, representing the minimum or maximum value of the quadratic function.
- Axis of Symmetry: This is a vertical line passing through the vertex, where both sides of the parabola are mirror images of each other.
- Roots: The points where the parabola crosses the x-axis (if any).
📝 Note: The direction the parabola opens (up or down) depends on the sign of a. If a > 0, the parabola opens upwards; if a < 0, it opens downwards.
Vertex Form of Quadratic Equations

The vertex form of a quadratic equation allows us to easily identify the vertex of the parabola:
\[ y = a(x - h)^2 + k \]
Where (h, k) is the vertex of the parabola.
Calculating Vertex from Standard Form

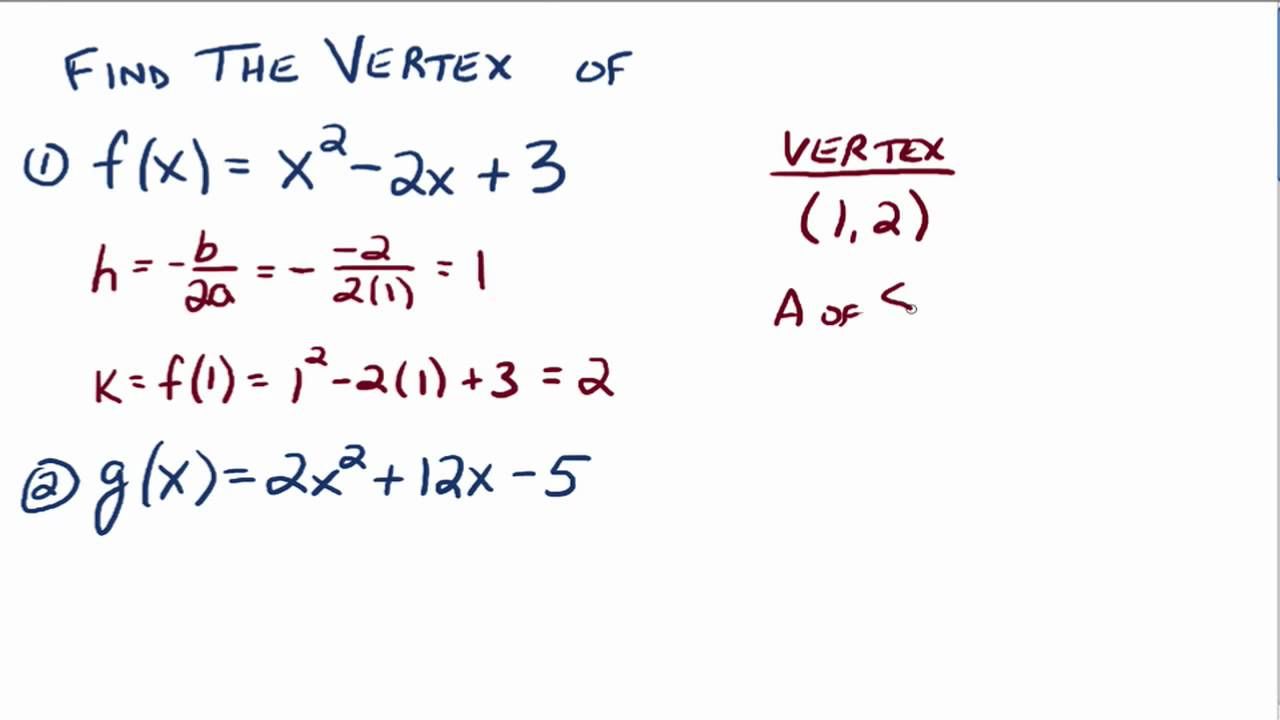
If given the standard form ax^2 + bx + c, we can find the vertex using the formulas:
- x-coordinate of vertex: h = -\frac{b}{2a}
- y-coordinate of vertex: Solve for y by substituting x = -\frac{b}{2a} into the equation.
Examples of Quadratic Equations through Vertices and Points

Example 1: Finding Vertex from Standard Form

Let's take the quadratic equation:
\[ y = 2x^2 - 8x + 7 \]
- Determine a = 2, b = -8, and c = 7.
- Calculate the x-coordinate of the vertex:
\[ h = -\frac{-8}{2 \cdot 2} = \frac{8}{4} = 2 \]
- Plug x = 2 back into the equation to find k:
\[ y = 2(2)^2 - 8(2) + 7 = -1 \]
- The vertex is at (2, -1).
Example 2: Vertex Form with Given Point

If we know the vertex and one point, we can determine the quadratic equation. Suppose the vertex is at (1, -3), and the point (3, 3) is given:
- The vertex form is y = a(x - 1)^2 - 3.
- Substitute x = 3 and y = 3:
\[ 3 = a(3 - 1)^2 - 3 \]
- Simplify and solve for a:
\[ 3 = a(2)^2 - 3 \] \[ 3 = 4a - 3 \] \[ 6 = 4a \] \[ a = 1.5 \]
- Thus, the equation in vertex form is:
\[ y = 1.5(x - 1)^2 - 3 \]
Example 3: Parabola with Multiple Points

Given three points on the parabola (like x-intercepts), we can find the quadratic equation:
| Point | x | y |
|---|---|---|
| A | -1 | 0 |
| B | 3 | 0 |
| C | 2 | 4 |

- Let's use the roots -1 and 3 to create the factored form:
\[ y = a(x + 1)(x - 3) \]
- Substitute the point (2, 4) to find a:
\[ 4 = a(2 + 1)(2 - 3) \] \[ 4 = -3a \] \[ a = -\frac{4}{3} \]
- Thus, the equation is:
\[ y = -\frac{4}{3}(x + 1)(x - 3) \]
📝 Note: Ensure to expand this equation for practical use in further calculations or graphing.
Wrapping Up

Exploring quadratic equations through their vertices and points not only enriches our understanding but also equips us with practical skills for analyzing parabolic shapes in various fields. From calculating maximum heights in physics to understanding economic models, the ability to grasp and manipulate these equations is invaluable.
What is the difference between the standard and vertex forms of quadratic equations?

+
The standard form is (ax^2 + bx + c) and is useful for various algebraic operations. The vertex form, (y = a(x - h)^2 + k), directly gives the vertex of the parabola, allowing for quick graphical analysis.
How can I find the vertex of a parabola if I only know the roots?

+
The roots give you the x-intercepts. The x-coordinate of the vertex is the average of these roots. To find (y), substitute the x-coordinate into the equation and solve for (y).
What does the coefficient (a) tell us about a quadratic equation?

+
The coefficient (a) determines the parabola’s direction and how narrow or wide it is. If (a > 0), the parabola opens upwards; if (a < 0), it opens downwards. A larger absolute value of (a) makes the parabola narrower, while a smaller one makes it wider.