5 Simple Ways to Master Polynomial Standard Form

Polynomials are fundamental elements in algebra, often representing functions or equations that can model many real-world phenomena. The standard form of a polynomial is a specific way to express it, making it easier to compare, add, subtract, or multiply polynomials. Mastering this form can significantly enhance your problem-solving capabilities in algebra and beyond. This blog post will explore five simple methods to help you master the standard form of polynomials, offering insights that can benefit students, educators, and anyone working with mathematics.
1. Understand the Concept of Standard Form
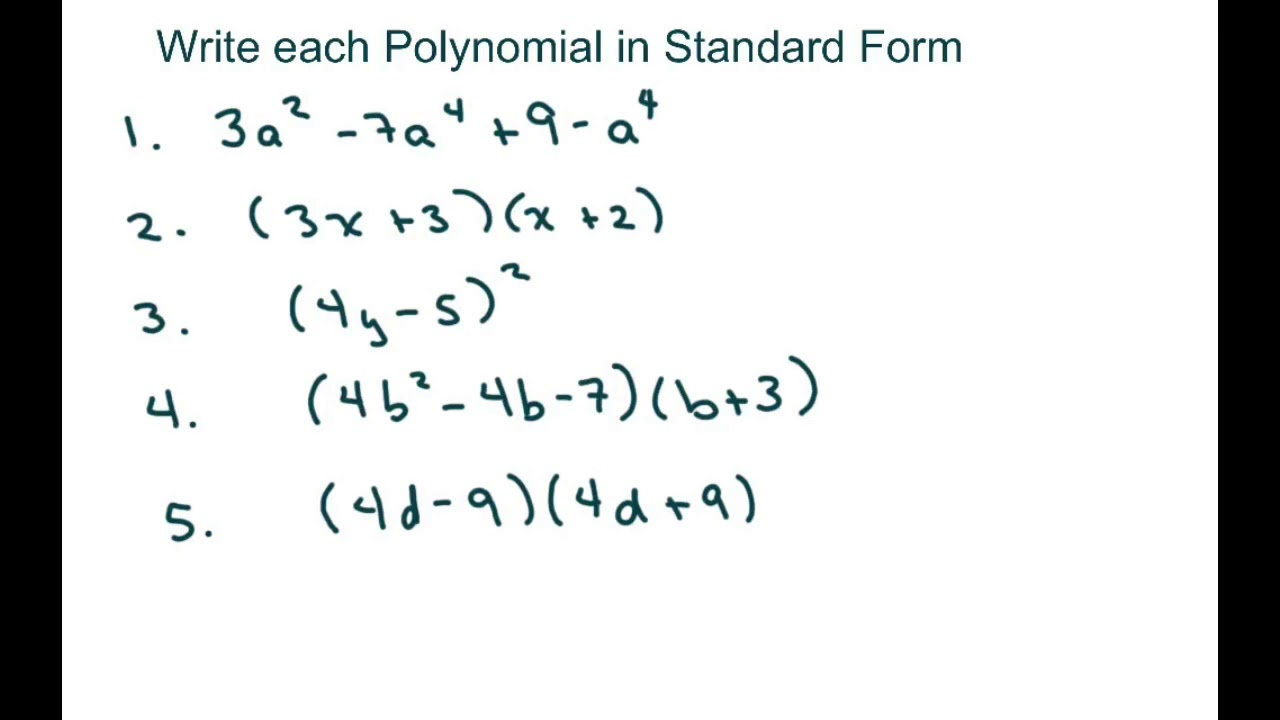
The first step towards mastering the polynomial standard form is understanding what it is:
- The polynomial is written in descending order of the degree.
- Each term consists of a variable (usually x), raised to a power, multiplied by a coefficient.
For example, the polynomial 3x² + 5x³ - 2x + 7 in its standard form would be 5x³ + 3x² - 2x + 7.
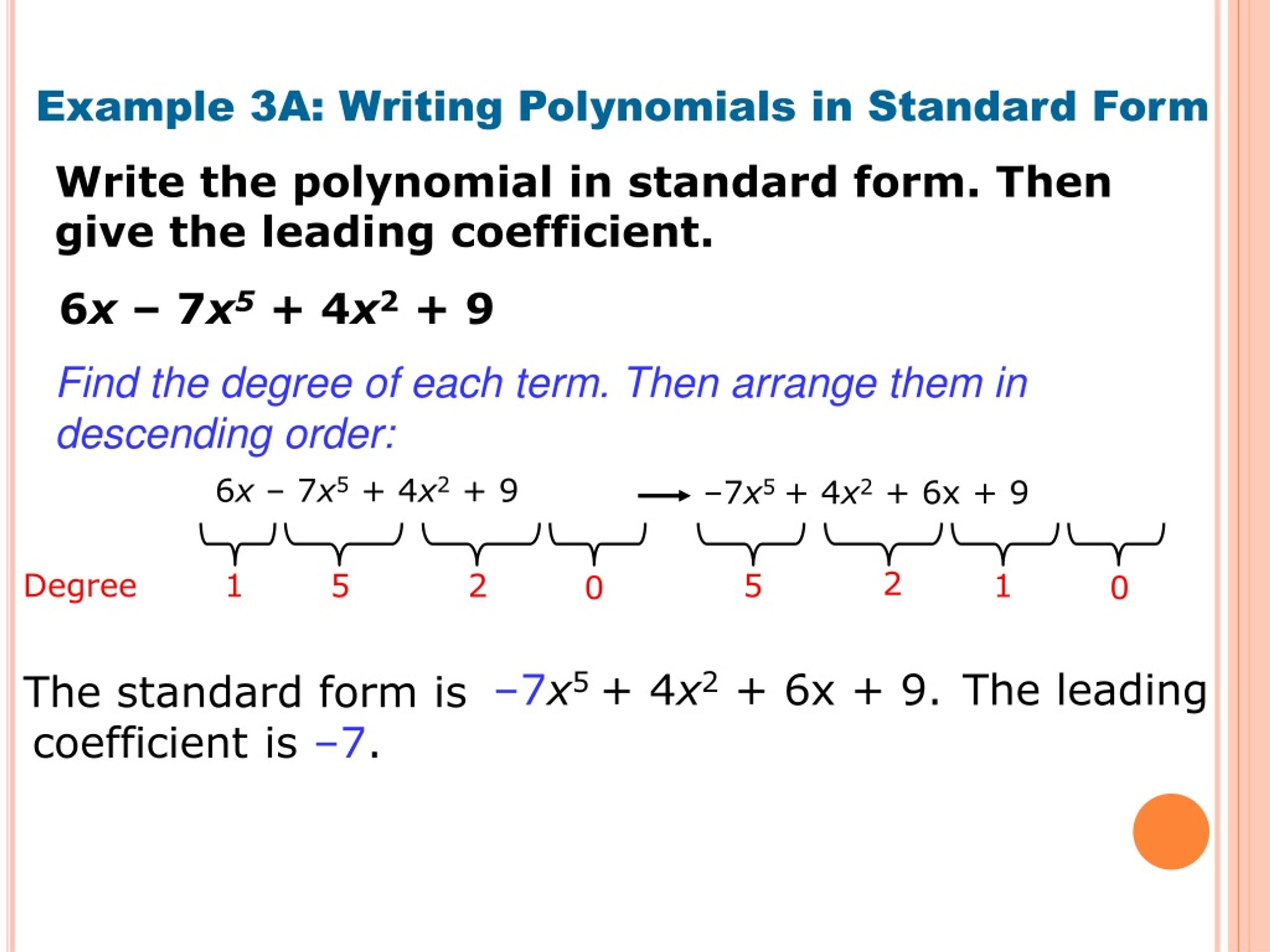
2. Identify Terms and Powers

Identifying the terms in a polynomial is essential:
- Every term has a variable (like x) raised to some power.
- Identify the coefficient, which can be positive or negative.
Here's how to identify and rearrange terms:
| Original Polynomial | Identify Terms | Standard Form |
|---|---|---|
| 3x² + 5x³ - 2x + 7 | 5x³ (Degree: 3), 3x² (Degree: 2), -2x (Degree: 1), 7 (Degree: 0) | 5x³ + 3x² - 2x + 7 |
💡 Note: Pay attention to the sign of the coefficient as it affects the term's position in the standard form.
3. Use Addition and Subtraction
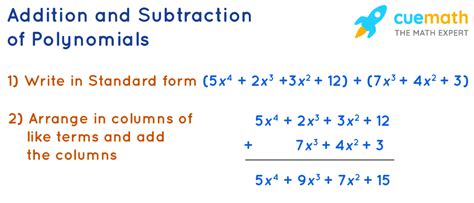
To convert polynomials into standard form:
- Add or subtract like terms by following the rules of addition and subtraction.
- Reorder the terms based on their degree.
Example:
- Original: x² + 5x - 3 - 4x + 2x²
- Combine like terms: 2x² + 3x - 3
- Sort by degree: 2x² + 3x - 3
4. Factorization Techniques

Factoring polynomials can aid in understanding their standard form:
- Factor common monomials.
- Identify and apply factorization patterns like sum and difference of cubes.
- Simplify the polynomial to make standard form conversion straightforward.
Here's an example:
- Original: x³ + 3x² - 4x
- Factor out common factor x: x(x² + 3x - 4)
- Check for patterns: (x + 4)(x - 1)x
- Sort in standard form: x³ - x² - 4x
5. Online Tools and Software
Modern technology can be your ally in mastering standard form:
- Use polynomial calculators for quick conversion.
- Online tools often allow for step-by-step solutions, helping to grasp the process.
- Software like Mathematica, Maple, or even Desmos can manipulate and simplify polynomials into standard form.
Understanding polynomial standard form not only simplifies algebraic operations but also deepens your insight into mathematical structures. By applying these five simple methods, you can enhance your proficiency in algebra, paving the way for more complex mathematical concepts. Remember that practice is key, and familiarity with these techniques will ensure you can seamlessly convert any polynomial into its standard form, making further algebraic manipulations straightforward and efficient.
📚 Note: Consistent practice with a variety of polynomials will reinforce these techniques and improve your speed and accuracy.
To further expand your understanding, let's explore some common questions and answers related to polynomials and their standard forms:
What is the degree of a polynomial?

+
The degree of a polynomial is the highest power of the variable in the expression. For example, in the polynomial 3x4 + 2x2 - 7, the degree is 4.
Why is it important to write polynomials in standard form?

+
Writing polynomials in standard form allows for easier comparison, addition, subtraction, and factorization. It also helps in recognizing patterns and simplifying algebraic expressions.
Can all polynomials be put into standard form?

+
Yes, any polynomial can be written in standard form by ordering terms in descending order of their degrees.