Master Nuclear Equations Easily: Chem Worksheet 4-4 Guide

Delving into the world of nuclear chemistry can often seem daunting. However, mastering the basics of nuclear equations through educational tools like Chem Worksheet 4-4 can significantly simplify this complex subject. Whether you're a high school student or an undergrad, understanding nuclear reactions is fundamental for a deeper grasp of chemistry. This guide aims to walk you through the steps to master nuclear equations, using the structure provided in Chem Worksheet 4-4 as our framework.
Understanding Nuclear Reactions

Nuclear reactions involve changes within an atomic nucleus, leading to the transformation of one nuclide into another. These transformations are driven by principles such as radioactivity, nuclear fusion, or fission. Here’s a brief overview:
- Radioactivity: The spontaneous decay of unstable atomic nuclei into more stable configurations by emitting particles or radiation.
- Nuclear Fusion: The process of combining light nuclei to form a heavier nucleus, releasing vast amounts of energy.
- Nuclear Fission: The splitting of a heavy nucleus into two or more lighter nuclei, often accompanied by the release of energy.
🚨 Note: Do not confuse nuclear reactions with chemical reactions, where electrons are rearranged; nuclear reactions involve changes in the protons and neutrons within the nucleus itself.
Key Components of Chem Worksheet 4-4

Chem Worksheet 4-4 provides a structured approach to balance nuclear equations:
- Symbol: Representation of the element, e.g., U for uranium.
- Mass Number (A): The total number of protons and neutrons in the nucleus.
- Atomic Number (Z): The number of protons in the nucleus.
- Charge: Any charge associated with the particle, mainly applicable in the case of charged ions or elementary particles like electrons.
Here’s a simple example of how these components come together in a nuclear equation:
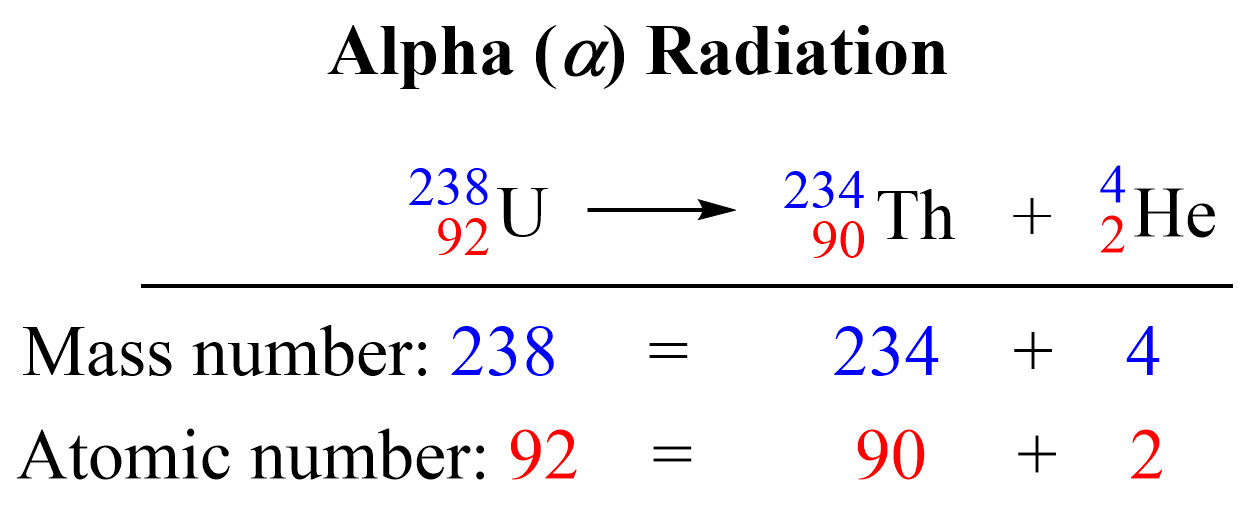
| Original Element | Nuclear Reaction | Product |
|---|---|---|
| 238U | α decay | 234Th + 4He |

Balancing Nuclear Equations

Balancing a nuclear equation ensures that the total number of nucleons (protons + neutrons) and the total charge (atomic numbers) are conserved. Follow these steps:
- Identify the reaction type: This could be alpha (α) decay, beta (β) decay, positron emission, electron capture, or induced nuclear reactions.
- Determine changes in Z and A:
- Alpha decay reduces Z by 2 and A by 4.
- Beta decay increases Z by 1 without changing A.
- Positron emission decreases Z by 1 without changing A.
- Electron capture decreases Z by 1 without changing A.
- Calculate the atomic and mass numbers for products or reactants: Use the conservation laws to find the missing particles or elements.
- Check and balance: Ensure that the sum of atomic and mass numbers on both sides of the equation are equal.
Here’s how you might approach a balancing problem:
- Suppose you have the decay of Thorium-234:
234Th90 -> 234Pa91 + X
📝 Note: Balancing nuclear equations helps in understanding the stability and transformation of isotopes, which is crucial in fields like radiochemistry and nuclear medicine.
Advanced Nuclear Reactions

Beyond the basic reactions, Chem Worksheet 4-4 introduces you to more complex scenarios:
- Positron Emission: A proton converts to a neutron, emitting a positron.
- Electron Capture: An electron from the inner atomic shell is captured by the nucleus, converting a proton to a neutron.
- Induced Reactions: Reactions where an external particle is used to initiate nuclear transformations.
These reactions broaden the scope of nuclear transformations, enriching the study of nuclear physics and its applications:
- Nuclear medicine: Radionuclide therapies and imaging.
- Energy production: Nuclear reactors for electricity.
- Archaeology: Carbon dating.
- Geology: Age dating of rocks.
Key Points to Master

To fully grasp nuclear equations using Chem Worksheet 4-4, keep these key points in mind:
- Always balance for both mass number (A) and atomic number (Z).
- Understand the different types of nuclear decay and the corresponding changes in A and Z.
- Use a periodic table to check the identity of the elements involved in reactions.
- Familiarize yourself with common decay particles like alpha (α), beta (β), gamma (γ), and their characteristics.
Summary

In summary, nuclear equations, although seemingly complex, can be mastered with a systematic approach like that provided by Chem Worksheet 4-4. By understanding the basic components of nuclear reactions, learning to balance equations, and exploring more advanced transformations, students can appreciate the vast applications of nuclear chemistry. From the foundations of atomic stability to practical applications in various scientific fields, nuclear reactions illuminate our understanding of the universe at its most fundamental level. The principles outlined here are not just academic exercises but are the keys to unlocking a deeper understanding of the physical world, with implications for technology, medicine, and our comprehension of natural processes.
What is the difference between nuclear fusion and nuclear fission?
+
Nuclear fusion is the process of combining light atomic nuclei to form heavier nuclei, releasing energy. Nuclear fission, conversely, is the splitting of a heavy nucleus into two or more lighter nuclei, also releasing energy.
How does alpha decay change an element’s atomic and mass numbers?

+
Alpha decay reduces the atomic number (Z) by 2 and the mass number (A) by 4 because an alpha particle (which is a helium nucleus) is ejected from the decaying atom.
Why is balancing nuclear equations important?

+
Balancing nuclear equations ensures conservation of both mass and charge, reflecting the laws of physics that govern nuclear reactions. It allows us to predict the products of these reactions and understand the stability of atomic nuclei.