5 Essential Tips for Creating Linear Equation Worksheets

The Power of Linearity

Linear equations stand as one of the most fundamental pillars in algebra, introducing students to the realm of mathematical logic and calculation. These equations, represented by a straight line on a graph, are not only essential for high school students but also form the basis for advanced mathematical concepts in calculus, economics, physics, and more. Here are five essential tips to ensure you're creating effective and educational linear equation worksheets for your students:
1. Understand the Core Concepts


Before you start crafting your worksheets, it's imperative to have a deep understanding of linear equations:
- Slope-intercept form: This form, y = mx + b, is where m represents the slope or gradient of the line, and b is the y-intercept.
- Standard form: ax + by = c, where the coefficients tell us about the equation's orientation.
- Point-slope form: y - y1 = m(x - x1), where a point and the slope are known.
Make sure your worksheets cover these forms comprehensively. This helps students grasp that linear equations are not just about solving for a variable, but also about understanding how the numbers relate to the graph's visual representation.
🔎 Note: Providing students with varied forms of linear equations reinforces their understanding that linear equations are not just mathematical constructs but also represent real-world phenomena.
2. Increase Complexity Gradually
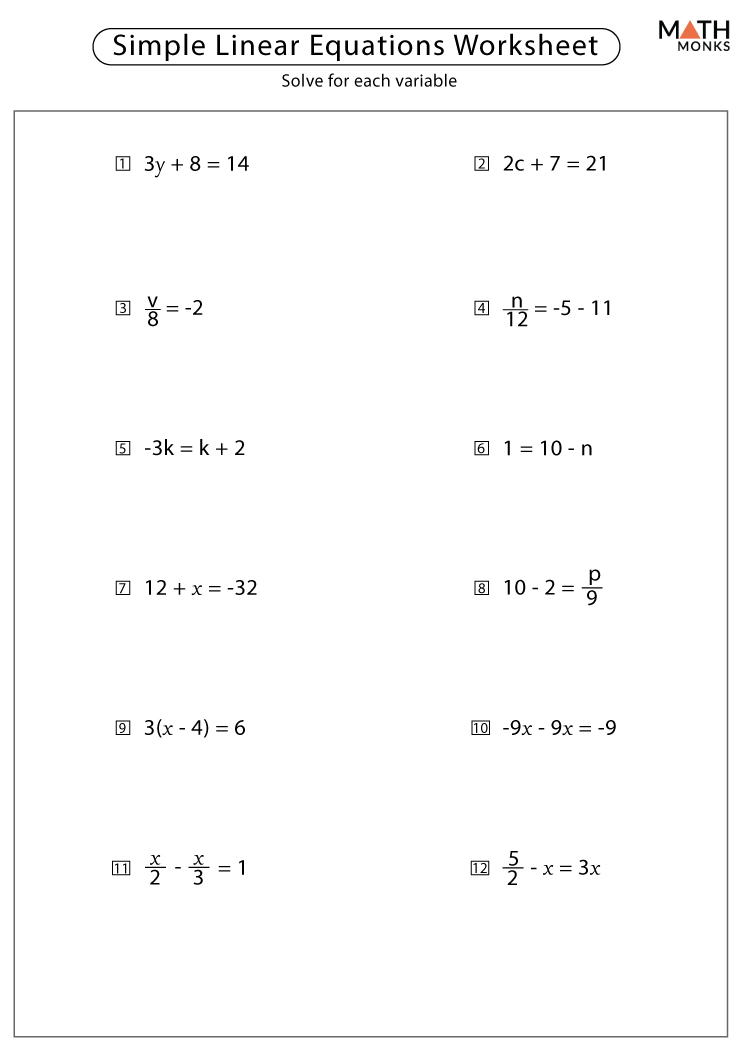
Linear equations can range from simple to quite complex. Here's how you can structure your worksheet to reflect this:
| Complexity Level | Example |
|---|---|
| Basic | y = 2x + 3 |
| Intermediate | 3x + y = 6 |
| Advanced | 2x - 3y + 15 = 0 |

Start with simple problems to ensure your students are comfortable with the basics. Then, progressively introduce complexity:
- Use negative numbers, fractions, or decimals for slopes and intercepts.
- Include mixed forms where students need to convert between different forms.
- Incorporate real-world problems where understanding the equation's slope and intercept has practical applications.
3. Visual and Contextual Engagement

Mathematics is often criticized for being too abstract, but linear equations provide an opportunity for visual and contextual engagement:
- Use graphs to illustrate the equations. Provide grids where students can plot points or draw lines.
- Real-world context is key. Create problems where students must find slopes, intercepts, or solve for variables in scenarios like cost analysis, distance calculations, or growth trends.
- Introduce applications from science, engineering, or economics to show the utility of linear equations.
These strategies make the abstract more tangible, fostering a deeper understanding and appreciation for the subject.
🔧 Note: Students often need to see the 'why' behind mathematics. Real-world examples can bridge the gap between theory and application, making linear equations more relatable.
4. Diverse Problem Types

Your worksheet should not just be about solving equations. Here are types of problems to consider:
- Equation solving
- Graphing equations
- Converting between equation forms
- Word problems requiring linear equations
- Systems of linear equations
By including a range of problem types, students are more likely to understand the versatility of linear equations in problem-solving.
5. Regular Feedback and Self-Checking

The learning process is iterative, and providing feedback is crucial:
- Include answers to some problems at the back of the worksheet or on a separate page.
- Design problems that allow students to self-check their work, perhaps by providing graphs where students can compare their plotted lines with the correct solutions.
- Provide feedback on common mistakes to guide students in the right direction.
Self-assessment helps students recognize their understanding and areas where they need more practice.
To sum up, crafting effective linear equation worksheets requires a balance between foundational knowledge, progressive complexity, visual aids, diverse problem types, and a system for feedback. With these five tips, your worksheets will not only challenge students but also inspire them to see the beauty and utility in the world of linear equations. The art of linear equations is all about connecting numbers and variables to represent and interpret the world around us in a way that's both logically sound and visually intuitive.
Why are linear equations important for students?

+
Linear equations are fundamental in algebra, providing a foundation for understanding how to solve equations, interpret data, and predict outcomes in various real-life scenarios.
Can linear equations be used in real-world applications?

+
Yes, they are widely used in fields like economics for cost analysis, physics for motion calculations, engineering for designing structures, and even in everyday situations like budgeting.
What’s the best way to teach the slope-intercept form?
+
Use visual aids like graphs to show how changes in m and b affect the line. Real-world examples where students calculate slope based on data points can also be effective.



