Mastering Linear Equations: Free Worksheet and Guide

In the realm of algebra, mastering the art of solving linear equations is a fundamental skill that forms the bedrock for more advanced mathematical exploration. Whether you're a student trying to grasp this concept or a parent looking to aid your child's learning, this guide and accompanying free worksheet will provide you with the tools to understand, practice, and excel in linear equations. Let's delve into the essentials of linear equations, understanding their structure, how to solve them, and how to apply this knowledge in various scenarios.
The Basics of Linear Equations

Linear equations are mathematical expressions of the form ax + b = c where:
- a is the coefficient of the variable, x,
- b is a constant term, and
- c is the value on the other side of the equals sign.
Here's what you need to remember:
- Linear equations are always straight lines when graphed.
- They have one variable raised to the power of one.
Examples and Explanation

Consider the equation 3x + 5 = 17:
- The coefficient a is 3, the variable is x, and b is 5.
- To solve, you aim to isolate x on one side of the equation:
- Subtract 5 from both sides to get 3x = 12.
- Divide by 3 to solve for x, resulting in x = 4.
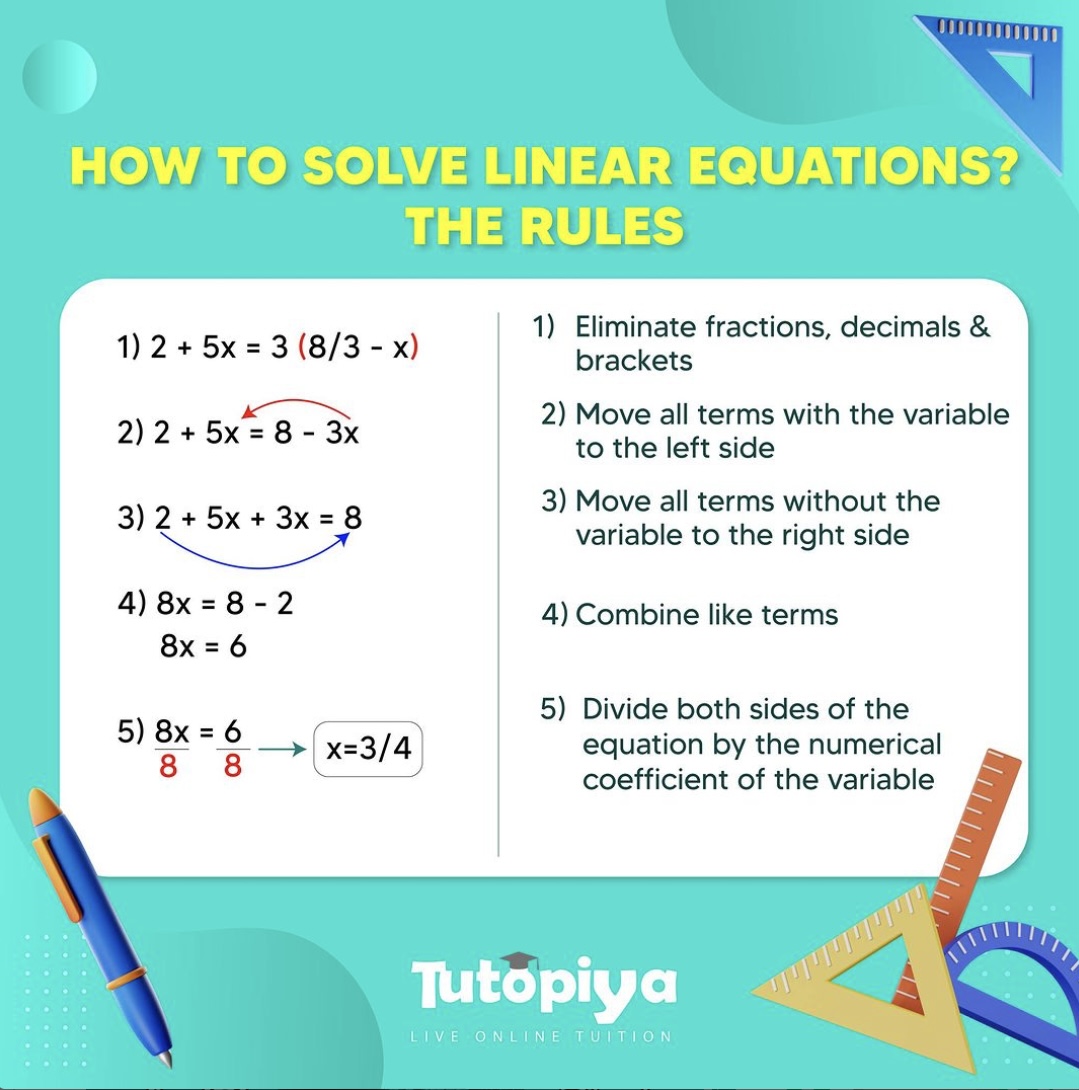
Step-by-Step Guide to Solving Linear Equations

Here's how you can solve a linear equation:
- Eliminate Constants: Subtract or add numbers to both sides to get rid of constants beside the variable.
- Isolate the Variable: Use multiplication or division to ensure the variable is on its own.
- Check your Solution: Plug your answer back into the original equation to verify it's correct.
Here are some specific techniques:
- Using inverse operations to isolate the variable.
- Solving equations with variables on both sides.
- Handling fractions or decimals in linear equations.
🔍 Note: While solving, remember that whatever you do to one side, you must do to the other to maintain the equation's balance.
Solving More Complex Linear Equations

When you encounter equations with fractions or multiple variables:
- Clear Fractions: Multiply every term by the least common denominator to eliminate fractions.
- Distribute: If there’s a parenthesis, distribute the term outside to all terms inside.
- Combine Like Terms: Simplify the equation by combining terms that can be added or subtracted.
Applications of Linear Equations

Linear equations aren’t just abstract; they’re everywhere in real-life:
- Business: Calculating profit margins, break-even points, and expenses.
- Physics: Determining force, velocity, or acceleration in straightforward cases.
- Travel and Geography: Calculating distances, time, and speeds.
Practical Examples
Here’s how you might use linear equations in real life:
- If you know a car travels at 60 mph and want to find out how far it will travel in 2 hours, you can use the equation distance = speed x time.
| Speed (mph) | Time (hours) | Distance (miles) |
|---|---|---|
| 60 | 2 | 120 |
| 45 | 3 | 135 |

🌐 Note: Linear equations often model real-world phenomena where things change at a constant rate.
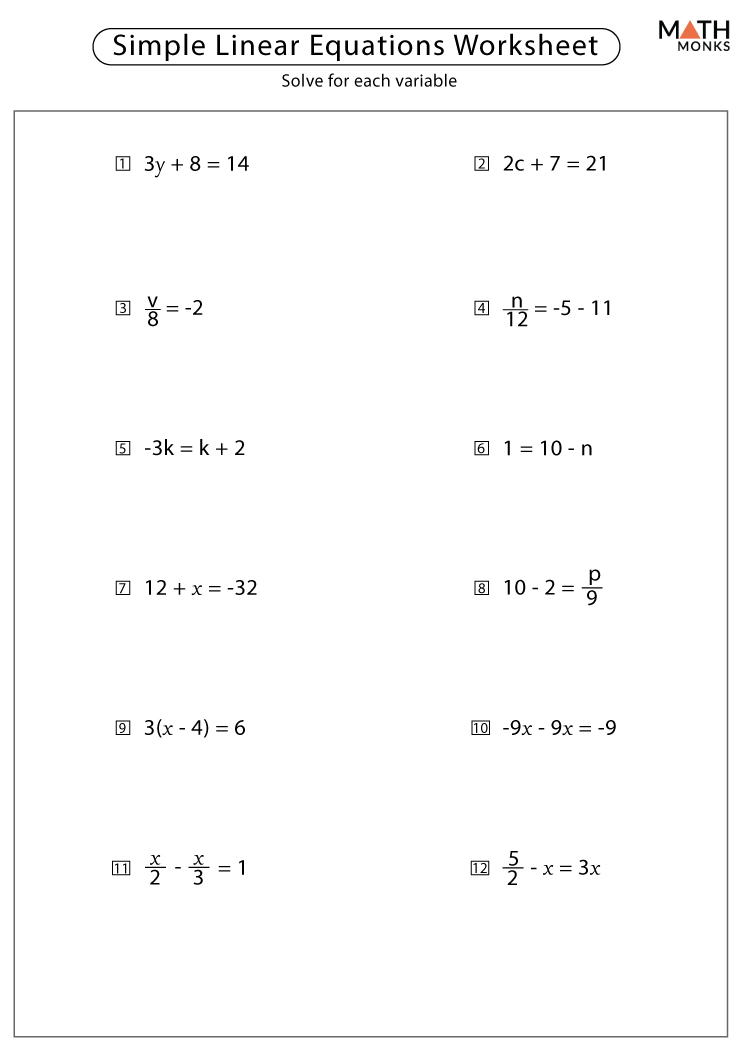
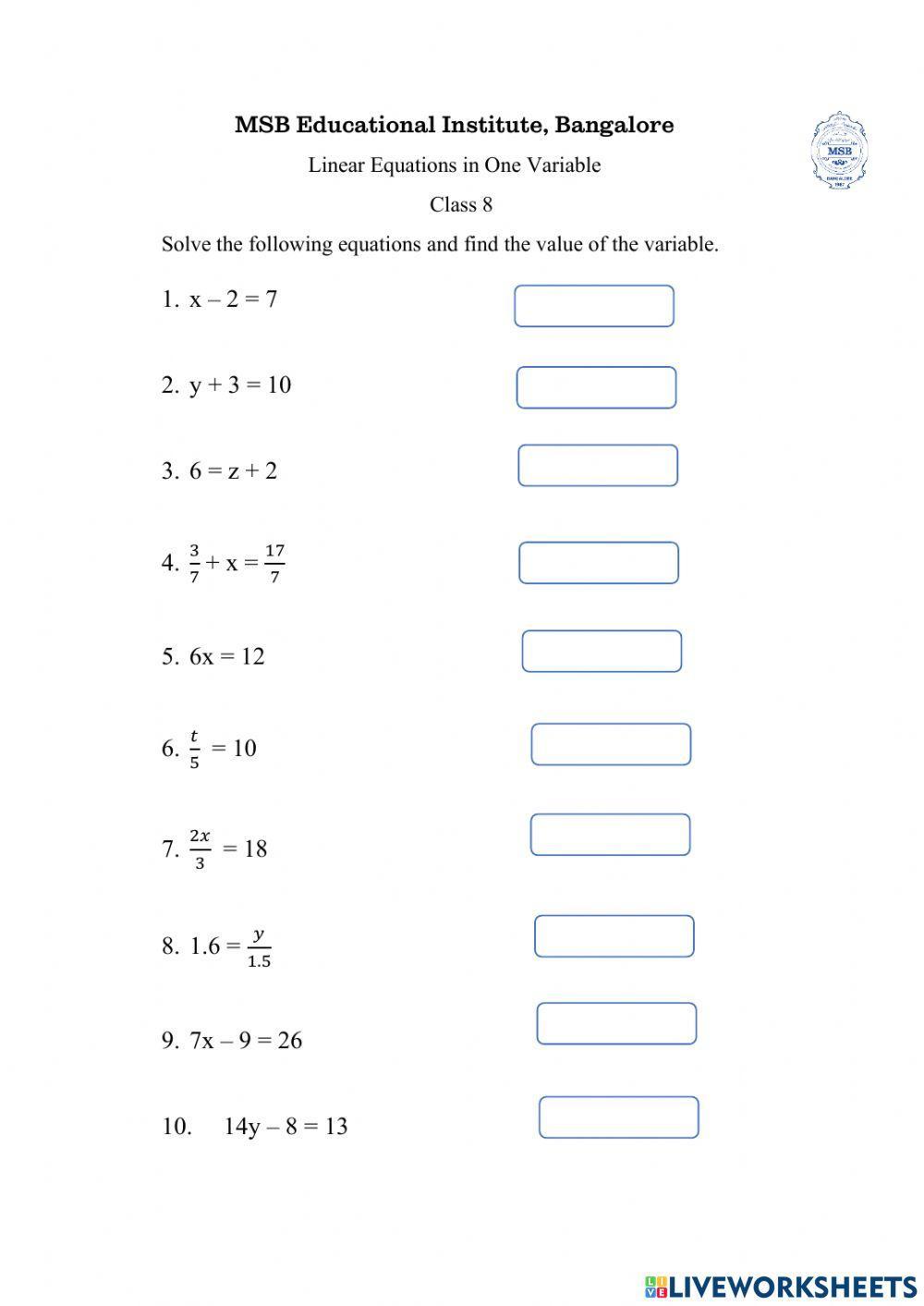
Free Worksheet for Mastering Linear Equations

Download and practice with our free worksheet to solidify your understanding. This worksheet includes:
- Basic linear equations to solve
- More complex equations with fractions and decimals
- Word problems that require setting up and solving linear equations
✍️ Note: Regular practice is key to mastering any subject, especially math. Keep practicing to increase your proficiency.
Through mastering linear equations, you've now gained a valuable tool in mathematics. From understanding basic structures to solving complex equations, you've explored both theoretical and practical applications. This foundational knowledge not only makes future math subjects easier to tackle but also empowers you to approach real-world problems with a structured mindset. With the step-by-step guides, practical examples, and the free worksheet, you're well-equipped to deepen your understanding of linear equations, enhance your problem-solving skills, and ensure success in your mathematical endeavors.
What is the difference between a linear equation and other types of equations?
![[35% Off] Mastering Graphs For Linear Equations: Part 2 [35% Off] Mastering Graphs For Linear Equations: Part 2](https://support.althea.net/assets/img/35-off-mastering-graphs-for-linear-equations-part-2.jpeg)
+
Linear equations have variables raised to the first power, resulting in a straight line on a graph. Other types, like quadratic equations, have variables raised to higher powers, like x², leading to curves or other complex shapes.
How can I check if my solution to a linear equation is correct?
+
Plug your solution back into the original equation. If both sides balance, your solution is correct.
Why is it important to understand linear equations?
+
Understanding linear equations is crucial because they are foundational for advanced mathematics, enabling problem-solving in various real-life scenarios involving constant rates of change.