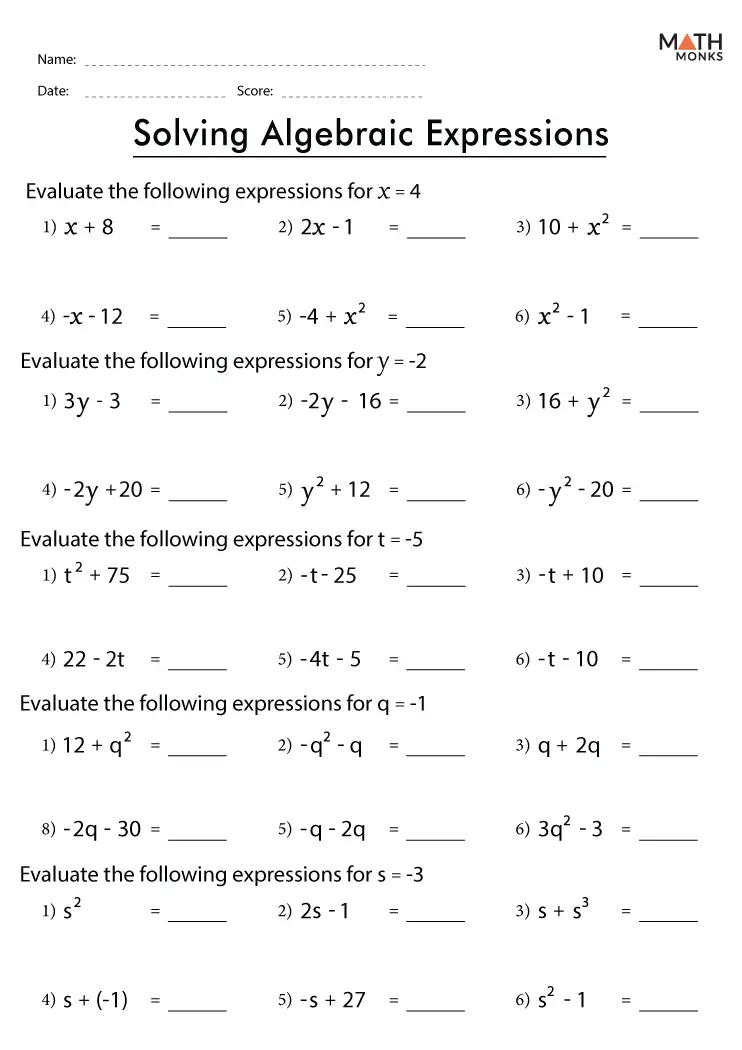
Algebraic Expressions Worksheet: Mastering Math Basics

The world of algebra can be both exciting and challenging for students as they navigate through their math education. Mastering algebraic expressions is a foundational step in this journey. This blog post dives deep into the essentials of algebraic expressions, offering insights, practical examples, and step-by-step guidance to enhance your understanding and proficiency.
Understanding Algebraic Expressions

Algebraic expressions are mathematical phrases that can contain numbers, variables, and mathematical operations like addition, subtraction, multiplication, and division. Understanding these expressions is crucial because they are the building blocks of more complex algebraic equations.
- Variables: These are symbols, usually letters, that represent unknown values. For example, in the expression x + 5, x is a variable.
- Constants: These are fixed numbers that do not change, like the 5 in the previous example.
- Operations: These include addition (+), subtraction (-), multiplication (×), and division (÷).
Let's start with basic algebraic expressions and then move on to more complex scenarios.
Basic Algebraic Expressions

At their simplest, algebraic expressions can be just a single term, like 3x or y. Here’s a look at some basic forms:
- 2 + x
- 5y - 3
- z * 4 (or 4z)
- m / 2 (or m/2)
📝 Note: Remember, when dealing with multiplication, you can omit the multiplication symbol for clarity and brevity, especially when multiplying a variable by a number. So, 3 × x is typically written as 3x.
Working with More Complex Expressions

As we delve into algebra, expressions become more intricate with multiple terms and operations:
- 2x2 + 3x - 7
- (5y + 4) - (3y - 2)
- 3(a + b) / c
Combining Like Terms
One of the first skills in simplifying expressions is learning to combine like terms, where terms with the same variable are added or subtracted:
- If you have 3x + 4x, you add the coefficients to get 7x.
- With 6y - 2y, you get 4y.
📚 Note: When combining like terms, only the coefficients are combined; the variable remains the same.
Distributing Over Parenthesis
When dealing with parenthetical expressions, distribution is key. Here’s how it works:
2(x + 3) becomes 2x + 6.
🔍 Note: Distribution means you multiply the term outside the parenthesis by each term inside.
Manipulating Expressions for Problem Solving

Algebraic expressions often need manipulation to isolate variables or solve for unknowns:
- Solve for x in the expression: 3x - 5 = 10
- Add 5 to both sides: 3x = 15
- Divide both sides by 3: x = 5
💡 Note: The goal in solving equations is to isolate the variable on one side of the equal sign, ensuring that operations performed on one side are also done on the other to maintain equality.
Applications in Real Life
Algebraic expressions are not just academic exercises; they have practical applications:
- Budgeting: y = x + 50 where y is the total amount you need to save, and x is your current savings. Here, the expression helps determine how much more you need to save.
- Physics: F = ma (Force equals mass times acceleration) is an algebraic equation used to calculate force in physics.
- Business: Calculating profits or losses can often be expressed algebraically, e.g., Profit = Revenue - (Fixed Costs + Variable Costs).
Algebraic expressions help simplify and make calculations more straightforward, transforming complex problems into manageable steps.
Wrapping Up

Algebraic expressions are fundamental in mathematics, serving as the groundwork for more advanced topics. By mastering these basics, students unlock a deeper understanding of mathematics and its applications in everyday life. From combining like terms, understanding the distribution of terms, to applying these concepts in real-world scenarios, the journey through algebraic expressions equips students with critical thinking and problem-solving skills that transcend academic settings. Keep practicing, and remember, the key to algebra is not just in solving equations but in understanding the logic and relationships between numbers and variables.
What are algebraic expressions?

+
Algebraic expressions are mathematical statements involving variables, constants, and mathematical operations. They represent relationships between quantities or the structure of equations.
Why are like terms important in algebra?

+
Like terms are crucial because they allow us to simplify and combine parts of an expression, making the calculation process more manageable and efficient.
Can you provide an example of distribution in algebra?

+
Yes, for instance, distributing 2 over the expression (x + 3) would result in 2x + 6. This means each term inside the parenthesis is multiplied by 2.
How does algebra apply to real life?

+
Algebra is used in budgeting, engineering, physics, business planning, and even daily problem-solving where you need to determine variables or outcomes based on known quantities.